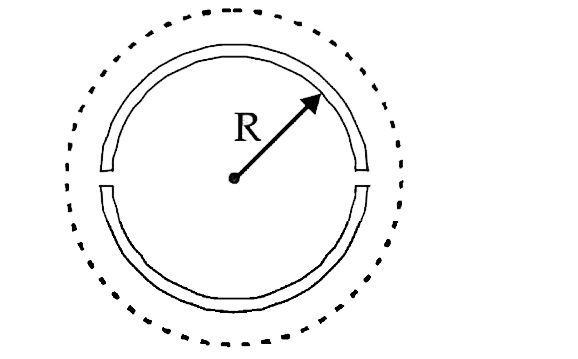
A wooden wheel of radius R is made of two semicircular part . The two parts are held together by a ring made of a metal strip of cross sectional area S and length L. L is slightly less than 2πR. To fit the ring on the wheel, it is heated so that its temperature rises by ΔTand it just steps over the wheel. As it cools down to surrounding temperature, it process the semicircle parts together. If the coefficient of linear expansion of the metal is α, and it Young's modulus is Y, the force that one part of the wheel applies on the other part is :
2πSYαΔT
SYαΔT
πSYαΔT
2SYαΔT
The correct Answer is:D
Step by step video & image solution for A wooden wheel of radius R is made of two semicircular part . The two parts are held together by a ring made of a metal strip of cross sectional area S and length L. L is slightly less than 2piR. To fit the ring on the wheel, it is heated so that its temperature rises by DeltaTand it just steps over the wheel. As it cools down to surrounding temperature, it process the semicircle parts together. If the coefficient of linear expansion of the metal is alpha, and it Young's modulus is Y, the force that one part of the wheel applies on the other part is : by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
Iron rims are heated before they are fixed on the wooden wheels. Explain the reason.
View SolutionA compressive force, F is applied at the two ends of a long thin steel rod. It is heated, simultaneously, such that its temeprature increass by ΔT. The net change in its length is zero. Let l be the length of the rod, A its area of cross-section, Y its Young's modulus, and α its coefficient of linear expansion. Then, F is equal to
View SolutionA compressive force, F is applied at the two ends of a long thin steel rod. It is heated, simultaneously, such that its temeprature increass by ΔT. The net change in its length is zero. Let l be the length of the rod, A its area of cross-section, Y its Young's modulus, and α its coefficient of linear expansion. Then, F is equal to
View SolutionTwo wires of the same materical having equal area of cross-section have L and 2L. Their respective resistances are in the ratio
View SolutionA uniform ring of radius R and made up of a wire of cross - sectional radius r is rotated about its axis with a frequency f. If density of the wire is ρ and young's modulus is Y . Find the fractional change in radius of the ring .
View SolutionThe radii of the wheel of a car is 28 cm. Find the number of rotations made by the wheel in order to cover a distance of 4.4 km ?
View SolutionThe length of an iron wire is L and area of cross-section is A. The increase in length is l on applying the force F on its two ends. Which of the statement is correct
View SolutionA current i passes through a wire of length l , radius of cross-section r and resistivity ρ . The rate of heat generation is
View SolutionTwo straight metallic strips each of thickness t and length l are rivetted together. Their coefficients of linear expansions areα1andα2. If they are heated through temperature ΔT, the bimetallic strip will bend to form an arc of radius
View SolutionA metal ring of initial radius r and cross-sectional area A is fitted onto a wooden disc of radius R > r. If Youngs modules of metal is Y then tension in the ring is
View Solution
