A compressive force, F is applied at the two ends of a long thin steel rod. It is heated, simultaneously, such that its temeprature increass by ΔT. The net change in its length is zero. Let l be the length of the rod, A its area of cross-section, Y its Young's modulus, and α its coefficient of linear expansion. Then, F is equal to
IAYαΔT
AYαΔT
AYαΔT
I2YαΔT
The correct Answer is:C
To solve the problem, we will analyze the effects of the compressive force and the thermal expansion of the steel rod. The goal is to find the expression for the force F when the net change in length is zero.
1. Understanding Thermal Expansion:
When the temperature of the rod increases by ΔT, the change in length ΔL due to thermal expansion can be expressed as:
ΔL=LαΔT
where:
- L is the original length of the rod,
- α is the coefficient of linear expansion,
- ΔT is the change in temperature.
2. Understanding Young's Modulus:
Young's modulus Y is defined as the ratio of stress to strain. Mathematically, it can be expressed as:
Y=StressStrain=F/AΔL/L
Rearranging this gives us the change in length ΔL in terms of the applied force F:
ΔL=FA⋅LY
3. Setting Up the Equation:
Since the net change in length is zero, the thermal expansion ΔL due to heating must equal the contraction ΔL due to the compressive force:
LαΔT=FA⋅LY
4. Canceling Common Terms:
We can cancel L from both sides of the equation (assuming L≠0):
αΔT=FA⋅1Y
5. Solving for the Force F:
Rearranging the equation to solve for F:
F=AYαΔT
Thus, the expression for the compressive force F is:
F=AYαΔT
When the temperature of the rod increases by ΔT, the change in length ΔL due to thermal expansion can be expressed as:
ΔL=LαΔT
where:
- L is the original length of the rod,
- α is the coefficient of linear expansion,
- ΔT is the change in temperature.
Young's modulus Y is defined as the ratio of stress to strain. Mathematically, it can be expressed as:
Y=StressStrain=F/AΔL/L
Rearranging this gives us the change in length ΔL in terms of the applied force F:
ΔL=FA⋅LY
Since the net change in length is zero, the thermal expansion ΔL due to heating must equal the contraction ΔL due to the compressive force:
LαΔT=FA⋅LY
We can cancel L from both sides of the equation (assuming L≠0):
αΔT=FA⋅1Y
Rearranging the equation to solve for F:
F=AYαΔT
F=AYαΔT
Topper's Solved these Questions
Similar Questions
What force is required to stretch a steel wire to double its length when its area of cross section 1 cm2 and Young.s modulus 2×1011N/m2.
View SolutionA rod of length L kept on a smooth horizontal surface is pulled along its length by a force F. The area of cross-section is A and Young's modulus is Y. The extension in the rod is

View SolutionA metal rod of length l, cross-sectional area A, Young's modulus Y and coefficient of linear expansion α is heated to t∘C. The work that can be performed by the rod when heated is
View SolutionA thin rod of negligible mass and area of cross section S is suspended vertically from one end. Length of the rod is L0 at T∘C . A mass m is attached to the lower end of the rod so that when temperature of the rod is reduced to 0∘C its length remains L0 Y is the Young’s modulus of the rod and α is coefficient of linear expansion of rod. Value of m is :
View SolutionA wooden wheel of radius R is made of two semicircular part . The two parts are held together by a ring made of a metal strip of cross sectional area S and length L. L is slightly less than 2πR. To fit the ring on the wheel, it is heated so that its temperature rises by ΔTand it just steps over the wheel. As it cools down to surrounding temperature, it process the semicircle parts together. If the coefficient of linear expansion of the metal is α, and it Young's modulus is Y, the force that one part of the wheel applies on the other part is :
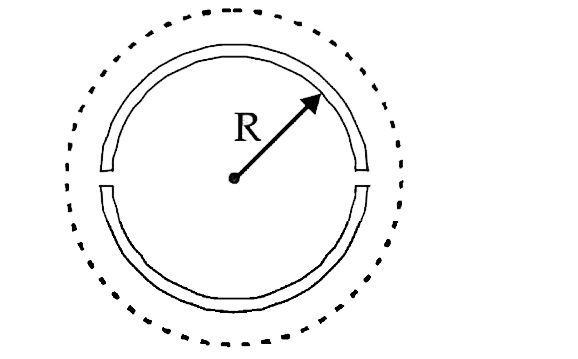
View Solutionon heating a cylindrical metallic rod, its length increases by 2% . Then area of cross section of rod will
View SolutionA rod PQ of length L revolves in a horizontal plane about the axis YY´. The angular velocity of the rod is w. If A is the area of cross-section of the rod and ρ be its density, its rotational kinetic energy is
View SolutionA bar of mass m and length l is hanging from point A as shown in figure.Find the increase in its length due to its own weight. The young's modulus of elasicity of the wire is Y and area of cross-section of the wire is A.

View SolutionA bar of mass m and length l is hanging from point A as shown in figure.Find the increase in its length due to its own weight. The young,s modulus of elasicity of the wire is Y and area of cross-section of the wire is A.

View SolutionTwo rods of equal cross sections, one of copper and the other of steel, are joined to form a composite rod of length 2.0 m at 20∘C, the length of the copper rod is 0.5 m. When the temperature is raised to 120∘C, the length of composite rod increases to 2.002m. If the composite rod is fixed between two rigid walls and thus not allowed to expand, it is found that the lengths of the component rods also do not change with increase in temperature. Calculate Young's moulus of steel. (The coefficient of linear expansion of copper, αc=1.6×10−5∘C and Young's modulus of copper is 1.3×1013N/m2).
View Solution
