Question

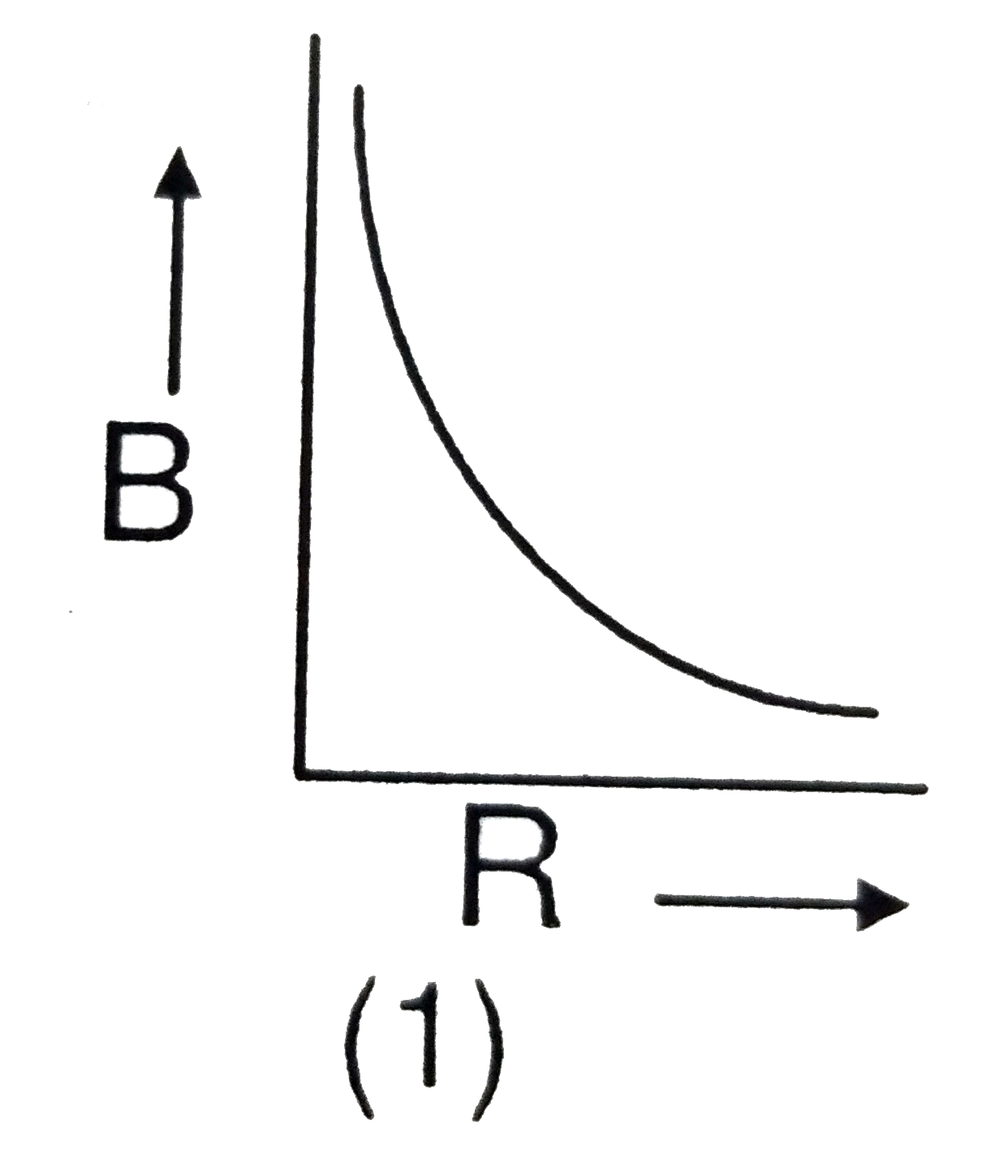
A charge $Q$ is uniformly distributed over the surface of non - conducting disc of radius $R$. The disc rotates about an axis perpendicular to its plane and passing through its centre with an angular to its plane and passing through its centre of the disc. If we keep both the amount of charge placed on the disc and its angular velocity to be constant and vary the radius of the disc then the variation of the magnetic induction at the centre of the disc will br represented by the figure:

Options

A.
B.
C.
D.

Answer

A

Explanation

To solve the problem step by step, we will analyze the magnetic field at the center of a rotating non-conducting disc with a uniformly distributed charge $$Q$$ over its surface.

Step 1: Understanding the Setup
We have a non-conducting disc of radius \( R \) with a total charge \( Q \) uniformly distributed over its surface. The disc rotates about an axis perpendicular to its plane and passing through its center with a constant angular velocity \( \omega \).

Step 2: Consider an Elemental Ring
To find the magnetic field at the center of the disc, we can consider an elemental ring of thickness \( dr \) at a distance \( r \) from the center of the disc. The area of this elemental ring is given by:
\( dA = 2 \pi r \, dr \)

Step 3: Charge on the Elemental Ring
The surface charge density \( \sigma \) is defined as:
\( \sigma = \frac{Q}{\pi R^2} \)
The charge \( dQ \) on the elemental ring can be expressed as:
\( dQ = \sigma \, dA = \sigma \cdot 2 \pi r \, dr = \frac{Q}{\pi R^2} \cdot 2 \pi r \, dr = \frac{2Qr}{R^2} \, dr \)

Step 4: Current Due to Rotation
The current \( I \) associated with the rotating ring can be calculated. The time period \( T \) for one complete rotation is given by:
\( T = \frac{2\pi}{\omega} \)
Thus, the current \( I \) through the ring is:
\( I = \frac{dQ}{T} = \frac{dQ \cdot \omega}{2\pi} = \frac{2Qr}{R^2} \cdot \frac{\omega}{2\pi} \, dr = \frac{Qr\omega}{\pi R^2} \, dr \)

Step 5: Magnetic Field Contribution from the Elemental Ring
The magnetic field \( dB \) at the center of the disc due to the current \( I \) in the elemental ring is given by:
\( dB = \frac{\mu_0 I}{2r} \)
Substituting the expression for \( I \):
\( dB = \frac{\mu_0}{2r} \cdot \frac{Qr\omega}{\pi R^2} \, dr = \frac{\mu_0 Q \omega}{2\pi R^2} \, dr \)

Step 6: Total Magnetic Field at the Center
To find the total magnetic field \( B \) at the center of the disc, we integrate \( dB \) from \( r = 0 \) to \( r = R \):
\( B = \int_0^R dB = \int_0^R \frac{\mu_0 Q \omega}{2\pi R^2} \, dr = \frac{\mu_0 Q \omega}{2\pi R^2} \cdot R = \frac{\mu_0 Q \omega}{2\pi R} \)

Step 7: Analyzing the Variation of Magnetic Field with Radius
From the expression we derived, we see that the magnetic field \( B \) is inversely proportional to the radius \( R \):
\( B \propto \frac{1}{R} \)
This indicates that as the radius \( R \) increases, the magnetic field \( B \) at the center decreases.

Conclusion
The variation of the magnetic induction \( B \) at the center of the disc as the radius \( R \) changes, while keeping \( Q \) and \( \omega \) constant, will be represented by a hyperbolic relationship, confirming that \( B \) decreases as \( R \) increases.