An ideal gas enclosed in a cylindrical container supports a freely moving piston of mass M. The piston and the cylinder have equal cross-sectional area A. When the piston is in equilibrium, the volume of the gas is V0 and its pressure is P0. The piston is slightly displaced from the equilibrium position and released. Assuming that the system is completely isolated from its surrounding, the piston executes a simple harmonic motion with frequency
12πV0MP0A2γ
12π√A2γP0MV0
12π√MV0AγP0
12πAγP0V0M
The correct Answer is:B
To find the frequency of the simple harmonic motion (SHM) of the piston in the ideal gas system, we can follow these steps:
1. Understanding the System:
- We have a piston of mass M in a cylindrical container with an ideal gas. The piston can move freely, and when in equilibrium, the gas has a volume V0 and pressure P0.
2. Displacement of the Piston:
- When the piston is displaced slightly downwards by a distance x, the volume of the gas changes. The change in volume dV due to the displacement of the piston is given by:
dV=A⋅dx
where A is the cross-sectional area of the piston.
3. Change in Pressure:
- For an ideal gas, the relationship between pressure and volume can be expressed using the adiabatic condition. The change in pressure dP can be derived from the equation:
dP=−γPVdV
where γ is the adiabatic index. Substituting dV into this equation gives:
dP=−γP0V0A⋅dx
4. Force on the Piston:
- The increase in force dF acting on the piston due to the change in pressure is:
dF=A⋅dP=−γP0V0A2⋅dx
5. Equating to Hooke's Law:
- The force can also be expressed in terms of Hooke's law for SHM:
F=−k⋅x
where k is the effective spring constant. From our previous equation, we can identify:
k=γP0A2V0
6. Finding the Frequency:
- The frequency f of SHM is given by:
f=12π√kM
Substituting the expression for k:
f=12π√γP0A2V0M
Final Answer:
Thus, the frequency of the simple harmonic motion of the piston is:
f=12π√γP0A2V0M
- We have a piston of mass M in a cylindrical container with an ideal gas. The piston can move freely, and when in equilibrium, the gas has a volume V0 and pressure P0.
- When the piston is displaced slightly downwards by a distance x, the volume of the gas changes. The change in volume dV due to the displacement of the piston is given by:
dV=A⋅dx
where A is the cross-sectional area of the piston.
- For an ideal gas, the relationship between pressure and volume can be expressed using the adiabatic condition. The change in pressure dP can be derived from the equation:
dP=−γPVdV
where γ is the adiabatic index. Substituting dV into this equation gives:
dP=−γP0V0A⋅dx
- The increase in force dF acting on the piston due to the change in pressure is:
dF=A⋅dP=−γP0V0A2⋅dx
- The force can also be expressed in terms of Hooke's law for SHM:
F=−k⋅x
where k is the effective spring constant. From our previous equation, we can identify:
k=γP0A2V0
- The frequency f of SHM is given by:
f=12π√kM
Substituting the expression for k:
f=12π√γP0A2V0M
Thus, the frequency of the simple harmonic motion of the piston is:
f=12π√γP0A2V0M
Topper's Solved these Questions
Similar Questions
An ideal gas enclosed in a cylinder fitted with a frictionless and weightless piston, pushes it outside. What would be the sign convention for the work ?
View SolutionAn ideal gas enclosed in a cylinder fitted with a frictionless and weightless piston, pushes it outside. What would be the sign convention for the work ?
View SolutionA cylindrical vessel containing a liquid is closed by a smooth piston of mass m as shown in the figure. The area of cross section of the piston is A. If the atmospheric pressure is P0, find the pressure of the liquid just below the piston.
 ,
,
View SolutionA cylidrical vesel containing a liquid is closed by a smooth piston of mass m as shown in the figure. The area of cross section of the piston is A. If the atmospheric pressure is P0, find the pressure of the liquid just below the piston.
 ,
,
View SolutionA smooth piston of mass m area of cross - section A is in equilibrium in a gas jar when the pressure of the gas is P0. Find the angular frequency of oscillation of the piston, assuming adiabatic Change of state of the gas.

View SolutionAn ideal gas is enclosed in a cylinder fitted with a frictionless piston. The piston is connected with a light rod to one plate of capacitor whose other plate is fixed as shown. Initially volume of the gas inside the cylinder is V0 Atmospheric pressure is P0 separation between the plates is L, area of the piston as well as of the capacitor plates is A and emf of battery is ε . A heater supplies heat to the gas so that pressure of the gas is given as P=P0−nε0ε2L2 when piston is displaced by a distance L2 . Find value of n.
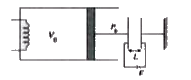
View SolutionMethod 3 of W
Mass of a piston shown in Fig. is m and area of cross-section is A. Initially spring is in its natural length. Find work done by the gas.

View SolutionAn ideal monoatomic gas is enclosed in a fixed horizontal adiabatic cylinder of cross sectional area A. The cylinder is fitted with an adiabatic piston of mass m (attached to one end of a spring as shown) which can move horizontally without friction inside the cylinder. In equilibrium, the spring is in natural length and pressure and volume are P0 and V0 respectively. The piston is slightly displaced from equilibrium and released. Then, frequency of small oscillation is

View Solutionshows a cylindrical tube of cross-sectional area A fitted with two frictionless pistons. The pistons are connected to each other by a metallic wire. Initially, the temperature of the gas is T0and its pressure is p0which equals the atmospheric pressure, (a)What is the tention in the wire?(b)What will be the tention if the temperature is increased to 2T_(0)?

View SolutionAn ideal gas is enclosed in a cyclinder with a movable piston on top. The piston has mass of 8000g and an area of 5.00cm2 and is free to slide up and down, keeping the pressure of the gas constant. How much work is done as the temperature of 0.200mol of the gas is raised from 200∘C to 300∘C?
View Solution
