When a rubber band is streched by a distance x , if exerts restoring force of magnitube F=ax+bx2 wherea and b are constant . The work in streached the unstreched rubber - band by L is
aL22+bL+33
12(aL22+bL33)
aL3+bL3
12(aL2+bL3)
To find the work done in stretching the unstretched rubber band by a length L, we start with the given restoring force:
F=ax+bx2
where a and b are constants, and x is the distance stretched. The work done W in stretching the rubber band from 0 to L can be calculated using the integral of the force over the distance stretched.
1. Define the Work Done:
The work done W in stretching the rubber band can be expressed as the integral of the force over the distance. Mathematically, this is given by:
W=∫L0Fdx
2. Substitute the Force Expression:
Substitute the expression for the force F into the integral:
W=∫L0(ax+bx2)dx
3. Split the Integral:
We can split the integral into two parts:
W=∫L0axdx+∫L0bx2dx
4. Calculate Each Integral:
- For the first integral:
∫L0axdx=a(x22)L0=a(L22−0)=aL22
- For the second integral:
∫L0bx2dx=b(x33)L0=b(L33−0)=bL33
5. Combine the Results:
Now, combine the results of the two integrals to find the total work done:
W=aL22+bL33
Final Answer:
Thus, the work done in stretching the unstretched rubber band by length L is:
W=aL22+bL33
The work done W in stretching the rubber band can be expressed as the integral of the force over the distance. Mathematically, this is given by:
W=∫L0Fdx
Substitute the expression for the force F into the integral:
W=∫L0(ax+bx2)dx
We can split the integral into two parts:
W=∫L0axdx+∫L0bx2dx
- For the first integral:
∫L0axdx=a(x22)L0=a(L22−0)=aL22
- For the second integral:
∫L0bx2dx=b(x33)L0=b(L33−0)=bL33
Now, combine the results of the two integrals to find the total work done:
W=aL22+bL33
Thus, the work done in stretching the unstretched rubber band by length L is:
W=aL22+bL33
Topper's Solved these Questions
- JEE MAINSBook:JEE MAINS PREVIOUS YEAR ENGLISHChapter:JEE MAINSExercise:Chemistry
Explore 1 Video
- JEE MAINBook:JEE MAINS PREVIOUS YEAR ENGLISHChapter:JEE MAINExercise:All Questions
Explore 452 Videos
- JEE MAINS 2020Book:JEE MAINS PREVIOUS YEAR ENGLISHChapter:JEE MAINS 2020Exercise:PHYSICS
Explore 250 Videos
Similar Questions
The relation between time t and distance x is t=ax2+bx where aandb are constants. The acceleration is
View SolutionWhen a spring a stretched through a distance x, it exerts a force given by F=(−5x−16x3)N. What is the work done, when the spring is stretched from 0.1 m to 0.2 m?
View SolutionForm the differential equation for the curve: y=Ax2+Bx, where a and b are arbitrary constant.
View SolutionLet f(x)=a+b|x|+c|x|2, where a,b,c are real constants. The, f'(0) exists if
View SolutionThe equation of motion of a projectile is y=ax−bx2, where a and b are constants of motion. Match the quantities in Column I with the relations in Column II.
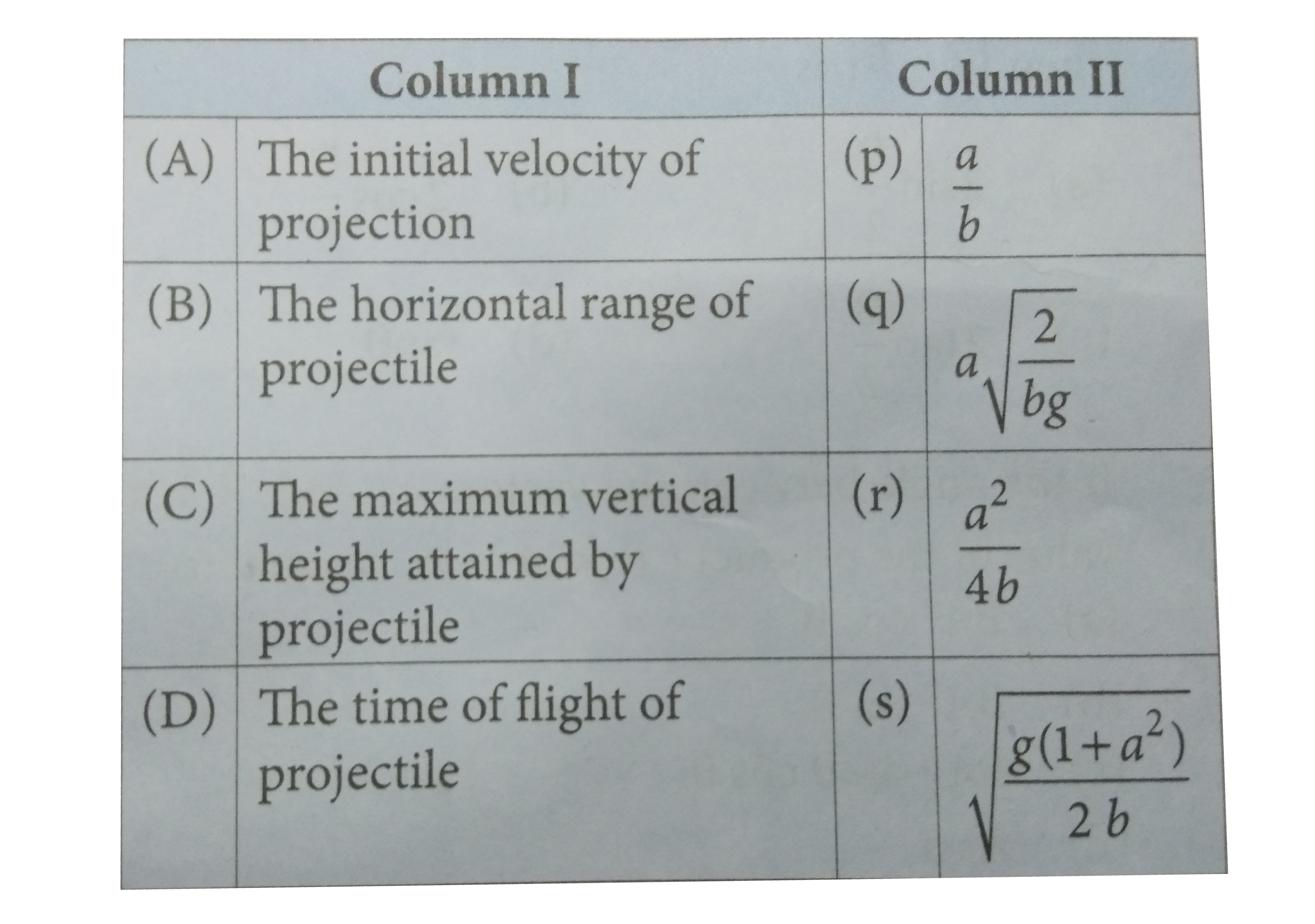
View SolutionA force F=a+bx acts on a particle in x-direction, where a and b are constants. Find the work done by this force during the displacement from x1 to x2.
View SolutionIf f(x)=a+bx+cx2 where a,b,c∈R then ∫1of(x)dx
View SolutionIn SHM restoring force is F=−kx, where k is force constant, x is displacement and a is amplitude of motion, then total energy depends upon
View SolutionA particle free to move along x-axis is acted upon by a force F=−ax+bx2whrteaandbarepositiveconstants., the correct variation of potential energy function U(x) is best represented by.
View SolutionA function has the form f(x)=ax+b, where a and b are constants. If f(2)=1 and f(−3)=11, the function is defined by
View Solution
