Question

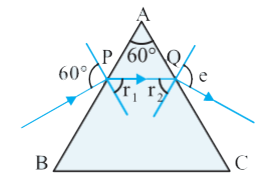
A ray of light is incident at an angle of ${60}^{\circ }$ on one face of a prism of angle ${30}^{\circ }$. The ray emerging out of the prism makes an angle of ${30}^{\circ }$ with the incident ray. The emergent ray is

Options

A. `0^(@)`
B. `45^(@)`
C. `90^(@)`
D. `30^(@)`

Answer

D

Explanation

To solve the problem, we need to determine the angle of the emergent ray as it exits the prism. Here's a step-by-step solution:

Step 1: Understand the given information
We are given:
- Angle of incidence (i) = 60°
- Angle of the prism (A) = 30°
- Angle between the incident ray and the emergent ray (Δ) = 30°

Step 2: Use the formula for the angle of deviation
The angle of deviation (Δ) for a prism can be expressed using the formula:
\( \Delta = i + e - A \)
where:
- \(i\) = angle of incidence
- \(e\) = angle of emergence
- \(A\) = angle of the prism

Step 3: Substitute the known values into the formula
Substituting the known values into the formula:
\( 30° = 60° + e - 30° \)

Step 4: Simplify the equation
Now, simplify the equation:
\( 30° = 60° + e - 30° \)
\( 30° = 30° + e \)

Step 5: Solve for the angle of emergence (e)
To isolate \(e\), we can subtract 30° from both sides:
\( 30° - 30° = e \)
\( 0° = e \)

Conclusion
The angle of emergence \(e\) is 0°. This means that the emergent ray is parallel to the base of the prism.

Final Answer
The emergent ray is at an angle of 0° with respect to the normal at the exit face of the prism.

---