Question

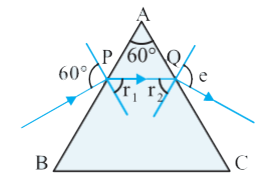
A ray of light is incident at an angle of ${60}^{\circ }$ on one face of a prism which has refracting angle of ${30}^{\circ }$ . The ray emerging out of the prism makes an angle of ${30}^{\circ }$ with the incident ray. If the refractive index of the material of the prism is $\mu =\sqrt{a}$ , find the value of a .

Answer

3

Explanation

To solve the problem, we will use Snell's law and the geometry of the prism. Let's break down the solution step by step.

Step 1: Understand the Geometry of the Prism
We have a prism with a refracting angle \( A = 30^\circ \). A ray of light is incident on one face of the prism at an angle \( i = 60^\circ \). The ray emerges from the prism making an angle of \( 30^\circ \) with the incident ray.

Step 2: Use the Relation Between Angles
According to the prism formula, the relationship between the angles is given by:
\( i + e - A = \Delta \)
where:
- \( i \) = angle of incidence = \( 60^\circ \)
- \( e \) = angle of emergence
- \( A \) = angle of the prism = \( 30^\circ \)
- \( \Delta \) = angle between the incident ray and the emergent ray = \( 30^\circ \)

Substituting the known values into the equation:
\( 60^\circ + e - 30^\circ = 30^\circ \)

Step 3: Solve for the Angle of Emergence
Rearranging the equation to solve for \( e \):
\( e = 30^\circ - 60^\circ + 30^\circ \)
\( e = 0^\circ \)
This means the ray emerges perpendicularly from the second face of the prism.

Step 4: Apply Snell's Law at the First Face
Using Snell's law at the first face of the prism:
\( n_1 \sin(i) = n_2 \sin(r) \)
Where:
- \( n_1 = 1 \) (refractive index of air)
- \( n_2 = \mu \) (refractive index of the prism)
- \( r \) = angle of refraction at the first face

From the geometry of the prism, since the angle of emergence \( e = 0^\circ \), the angle of refraction \( r \) at the first face can be calculated as:
\( r = 90^\circ - A = 90^\circ - 30^\circ = 60^\circ \)

Now applying Snell's law:
\( 1 \cdot \sin(60^\circ) = \mu \cdot \sin(30^\circ) \)
\( \sin(60^\circ) = \mu \cdot \sin(30^\circ) \)

Step 5: Substitute Known Values
Substituting the values of sine:
\( \frac{\sqrt{3}}{2} = \mu \cdot \frac{1}{2} \)

Step 6: Solve for the Refractive Index \( \mu \)
Rearranging gives:
\( \mu = \frac{\sqrt{3}}{2} \cdot 2 = \sqrt{3} \)

Step 7: Relate \( \mu \) to \( a \)
Given that \( \mu = \sqrt{a} \):
\( \sqrt{3} = \sqrt{a} \)

Step 8: Square Both Sides
Squaring both sides:
\( 3 = a \)

Final Answer
Thus, the value of \( a \) is:
\( \boxed{3} \)