Two masses each with mass 0.10kg are moving with velocities 3m/s along x axis and 5m/s along y-axis respectively. After an elastic collision one of the mass moves with a velocity 4ˆi+4ˆj . The energy of other mass after collision is x10 then x is
The correct Answer is:1
To solve the problem step by step, we will use the principles of conservation of momentum and conservation of kinetic energy, as it is an elastic collision.
Step 1: Identify the initial conditions
We have two masses, each with a mass m=0.10kg. The first mass is moving with a velocity →v1=3^im/s and the second mass is moving with a velocity →v2=5^jm/s.
Step 2: Calculate the initial kinetic energy
The initial kinetic energy (KEi) of the system can be calculated using the formula:
KEi=12mv21+12mv22
Substituting the values:
KEi=12(0.10)(32)+12(0.10)(52)
=12(0.10)(9)+12(0.10)(25)
=0.05×9+0.05×25=0.45+1.25=1.70J
Step 3: Determine the final velocity of one mass
After the collision, one mass moves with a velocity →v′1=4^i+4^j. We need to calculate the kinetic energy of this mass.
Step 4: Calculate the kinetic energy of the first mass after collision
The kinetic energy (KEf) of the first mass after the collision is given by:
KEf=12mv′21
Calculating v′1:
v′1=√(4)2+(4)2=√16+16=√32=4√2m/s
Now substituting this into the kinetic energy formula:
KEf=12(0.10)(4√2)2
=12(0.10)(32)=0.05×32=1.6J
Step 5: Apply conservation of kinetic energy
Since the collision is elastic, the total initial kinetic energy equals the total final kinetic energy:
KEi=KEf+KEother
Where KEother is the kinetic energy of the second mass after the collision. Thus:
1.70=1.6+KEother
Solving for KEother:
KEother=1.70−1.6=0.10J
Step 6: Relate KEother to x
According to the problem, the energy of the other mass after the collision is given as x10. Therefore:
0.10=x10
Multiplying both sides by 10 gives:
x=1
Final Answer
Thus, the value of x is 1.
---
We have two masses, each with a mass m=0.10kg. The first mass is moving with a velocity →v1=3^im/s and the second mass is moving with a velocity →v2=5^jm/s.
The initial kinetic energy (KEi) of the system can be calculated using the formula:
KEi=12mv21+12mv22
Substituting the values:
KEi=12(0.10)(32)+12(0.10)(52)
=12(0.10)(9)+12(0.10)(25)
=0.05×9+0.05×25=0.45+1.25=1.70J
After the collision, one mass moves with a velocity →v′1=4^i+4^j. We need to calculate the kinetic energy of this mass.
The kinetic energy (KEf) of the first mass after the collision is given by:
KEf=12mv′21
Calculating v′1:
v′1=√(4)2+(4)2=√16+16=√32=4√2m/s
Now substituting this into the kinetic energy formula:
KEf=12(0.10)(4√2)2
=12(0.10)(32)=0.05×32=1.6J
Since the collision is elastic, the total initial kinetic energy equals the total final kinetic energy:
KEi=KEf+KEother
Where KEother is the kinetic energy of the second mass after the collision. Thus:
1.70=1.6+KEother
Solving for KEother:
KEother=1.70−1.6=0.10J
According to the problem, the energy of the other mass after the collision is given as x10. Therefore:
0.10=x10
Multiplying both sides by 10 gives:
x=1
Thus, the value of x is 1.
Topper's Solved these Questions
Similar Questions
Two balls of masses m each are moving at right angle to each other with velocities 6 m/s and 8 m/s respectively. If collision between them is perfectly inelastic, the velocity of combined mass is
View SolutionTwo elastic bodies P and Q having equal mases are moving along the same line with velocities of 16 m/s and 10 m/s respectively. Their velocities after the elastic collision will be in m/s :
View Solution
Knowledge Check
A particle of mass 1 g moving with a velocity →v1=3ˆi−2ˆjms−1 experiences a perfectly in elastic collision with another particle of mass 2 g and velocity →v2=4ˆj−6ˆkms−1. The velocity of the particle is
A2.3ms−1B4.6ms−1C9.2ms−1D6ms−1A ball weighing 4 kg and moving with velocity of 8 m/sec. Collides with a stationary ball of mass 12 kg. After the collision, the two balls move together. The final velocity is
A0B2 m/secC8 m/secD16 m/secA body of mass m, moving with velocity μ collides elasticity with another body at rest having mass M. If the body of mass M moves with velocity V, then the velocity of the body of mass m after the impact is
Am−Mm+MμBm−Mm+MVCm+MVm+MDmu−MVm
A particle moving with velocity (2ˆi−3ˆj) m/s collides with a surface at rest in xz–plane as shown in figure and moves with velocity (2ˆi+2ˆj) m/s after collision. Then coefficient of restitution is :
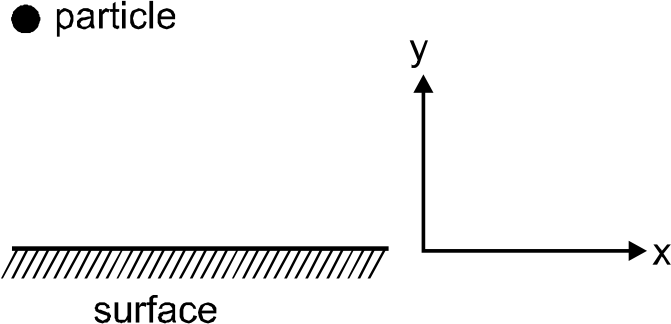
View SolutionA body A, of m=0.1 kg has a initial velocity of 3ˆikms−1. It collides elastically with another body. B of the same mass which has an initial velocity of 5ˆjms−1. After collision, A moves with a velocity →v=4(ˆi+ˆj). The energy of B after collision is written as x10J. The value of x is.
View SolutionTwo balls of masses 2kg and 4kg are moved towards each other with velocities 4m/s and 2m/s, respectively, on a frictionless surface. After colliding, the 2kg ball returns back with velocity 2m/s. then find
a. velocity of the 4kg after collision
b. coefficient of restitution e,
c. impulse of deformation JD
d. maximum potential energy of deformation,
e. impulse of reformation JR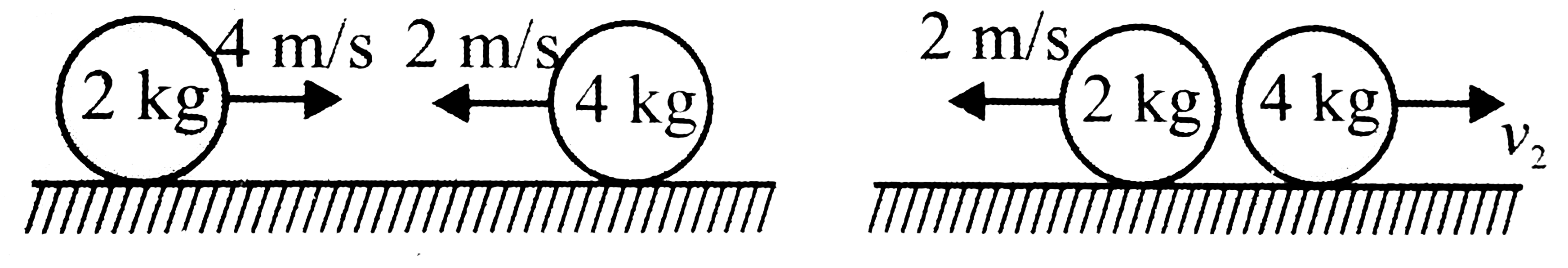
View SolutionA body of mass M moving with a speed u has a ‘head on’, perfectly elastic collision with a body of mass m initially at rest. If M>>m , the speed of the body of mass m after collision, will be nearly :
View SolutionA body of mass 3 kg moving with a velocity (2ˆi+3ˆj+3ˆk) m/s collides with another body of mass 4 kg moving with a velocity (3ˆi+2ˆj−3ˆk) m/s. The two bodies stick together after collision. The velocity of the composite body is
View Solution
