A current carrying circular loop is placed in an infinite plane if ϕi is the magnetic flux through the inner region and ϕo is magnitude of magnetic flux through the outer region, then
ϕi=−ϕ0
ϕi=ϕ0
ϕi<ϕ0
ϕi>ϕ0
The correct Answer is:A
To solve the problem, we need to understand the relationship between the magnetic flux through the inner and outer regions of a current-carrying circular loop placed in an infinite plane.
1. Understanding Magnetic Flux:
Magnetic flux (Φ) through a surface is defined as the product of the magnetic field (B) passing through that surface and the area (A) of the surface, mathematically given by:
Φ=∫→B⋅d→A
where d→A is the differential area vector.
2. Magnetic Field Due to a Circular Loop:
A circular loop carrying current generates a magnetic field that forms closed loops around the wire. The direction of the magnetic field inside the loop is different from that outside the loop.
3. Analyzing the Inner and Outer Regions:
- For the inner region of the loop, the magnetic field lines are directed inwards (towards the center of the loop).
- For the outer region, the magnetic field lines are directed outwards (away from the center of the loop).
4. Direction of Magnetic Flux:
Since the magnetic field direction is opposite in the inner and outer regions, the magnetic flux through these regions will also have opposite signs.
5. Mathematical Representation:
Let Φi be the magnetic flux through the inner region and Φo be the magnetic flux through the outer region. Due to the opposite directions of the magnetic fields in these regions, we can express the relationship as:
Φi=−Φo
This indicates that the magnetic flux in the inner region is equal in magnitude but opposite in sign to the magnetic flux in the outer region.
6. Conclusion:
Therefore, the relationship between the magnetic fluxes through the inner and outer regions can be summarized as:
Φi=−Φo
Final Answer:
The relationship between the magnetic flux through the inner region (Φi) and the outer region (Φo) is:
Φi=−Φo
Magnetic flux (Φ) through a surface is defined as the product of the magnetic field (B) passing through that surface and the area (A) of the surface, mathematically given by:
Φ=∫→B⋅d→A
where d→A is the differential area vector.
A circular loop carrying current generates a magnetic field that forms closed loops around the wire. The direction of the magnetic field inside the loop is different from that outside the loop.
- For the inner region of the loop, the magnetic field lines are directed inwards (towards the center of the loop).
- For the outer region, the magnetic field lines are directed outwards (away from the center of the loop).
Since the magnetic field direction is opposite in the inner and outer regions, the magnetic flux through these regions will also have opposite signs.
Let Φi be the magnetic flux through the inner region and Φo be the magnetic flux through the outer region. Due to the opposite directions of the magnetic fields in these regions, we can express the relationship as:
Φi=−Φo
This indicates that the magnetic flux in the inner region is equal in magnitude but opposite in sign to the magnetic flux in the outer region.
Therefore, the relationship between the magnetic fluxes through the inner and outer regions can be summarized as:
Φi=−Φo
The relationship between the magnetic flux through the inner region (Φi) and the outer region (Φo) is:
Φi=−Φo
Topper's Solved these Questions
Similar Questions
A closed current carrying circular loop is placed on one face of a cubical box as shown in fig. The magnetic flux through area of the box contained by the loop is ϕ1 and the magnetic flux through remaining surface area of the box is ϕ2 . Which of the following option is correct

View SolutionA closed current carrying circular loop is placed on one face of a cubical box as shown in fig. The magnetic flux through area of the box contained by the loop is ϕ1 and the magnetic flux through remaining surface area of the box is ϕ2 . What is relation between them

View Solution
Knowledge Check
The net magnetic flux through any closed surface, kept in a magnetic field is
AzeroBμ04πC4πμ0D4μ0π
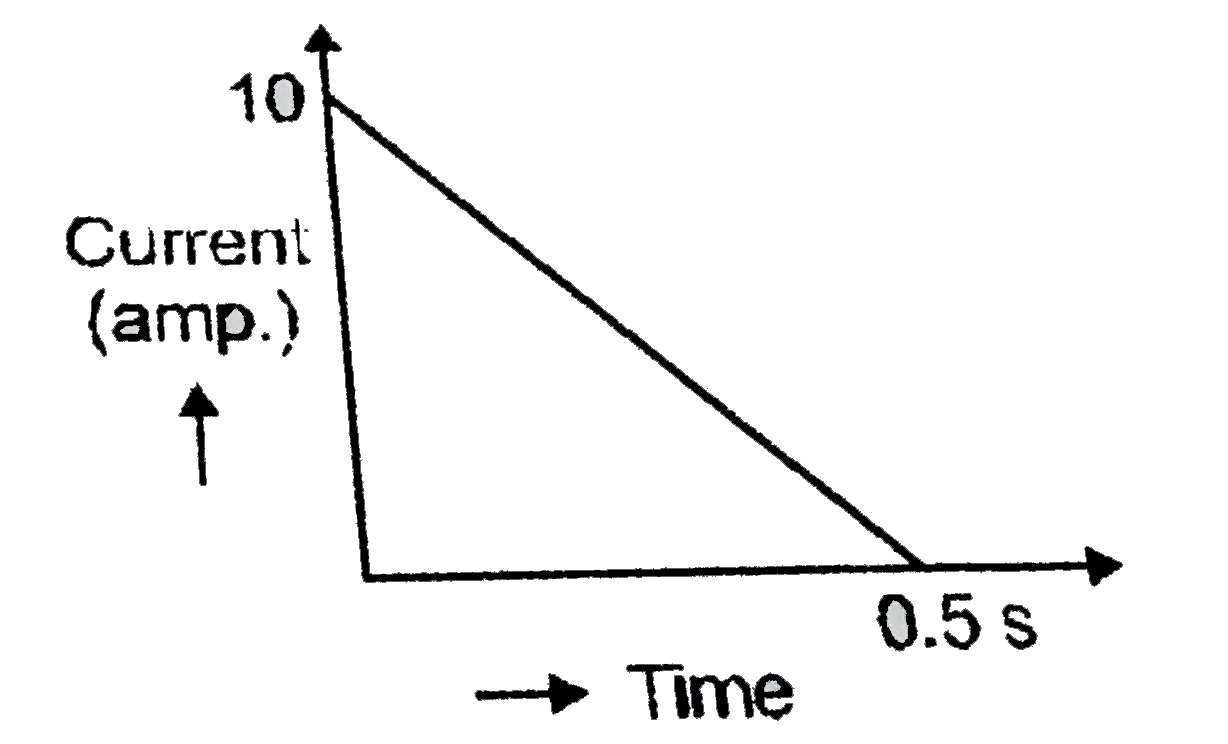
In a coil of resistance 100Ω , a current is induced by changing the magnetic flux through it as shown in the figure. The magnitude of change in flux through the coil is
View SolutionA current carrying circular loop is located in a uniform external magnetic field. If the loop if free to turn, what is its orientation of stable equilibrium? Show that in this orientation, the flux of the total field (external field + field produced by the loop) is maximum.
View SolutionA circular coil, carrying a constant current i s kept in the x - y plane. The magnetic flux through the entire x - y plane exluding the area of the circular coil is given by ϕ and the magetic flux through the area of the circular coil area is given by ϕ0, then
View SolutionConsider a short bar magnet forming a magnetic dipole enclosed by an imaginary co-axial cylindrical surface with circular base area. Magnet is at the middle of cylinder. If magnetic flux through one of the circular base is phi _(0) then the magnetic flux through the other circular base will be:
View SolutionA circular loop of radius R , carrying I, lies in x−y plane with its origin . The total magnetic flux through x−y plane is
View SolutionA circular loop of radius R , carrying I, lies in x−y plane with its origin . The total magnetic flux through x−y plane is
View SolutionConsider a short bar magnet forming a magnetic dipole enclosed by an imaginary co-axial cylindrical surface with circular base area. Magnet is at the middle of cylinder. If magnetic flux through one of the circular base is ϕ0 then the magnetic flux through the other circular base will be:
View Solution
