Consider a loop ABCDEFA. With coordinates A (0, 0, 0), B(5, 0, 0), C(5, 5, 0), D(0, 5, 0) E(0, 5, 5) and F(0, 0, 5). Find magnetic flux through loop due to magnetic field →B=3ˆi+4ˆk
The correct Answer is:175 Wb
To find the magnetic flux through the loop ABCDEFA due to the magnetic field →B=3^i+4^k, we will follow these steps:
Step 1: Identify the coordinates of the loop
The coordinates of the loop ABCDEFA are:
- A (0, 0, 0)
- B (5, 0, 0)
- C (5, 5, 0)
- D (0, 5, 0)
- E (0, 5, 5)
- F (0, 0, 5)
Step 2: Determine the area of the loop
The loop ABCD lies in the xy-plane, forming a square. The side length of the square is 5 units (from A to B or A to D).
The area A of the square is given by:
A=side2=52=25square units
Step 3: Determine the area vector
The area vector →A for the loop ABCD is perpendicular to the plane of the loop. Since the loop lies in the xy-plane, the area vector points in the positive z-direction:
→AABCD=25^k
For the loop ADEF, which is vertical, the area vector points in the negative x-direction:
→AADEF=25^i
Step 4: Combine the area vectors
The total area vector →A for the entire loop ABCDEFA is the sum of the area vectors of the two sections:
→A=→AABCD+→AADEF=25^k+25^i
Step 5: Calculate the magnetic flux
The magnetic flux Φ through the loop is given by the dot product of the magnetic field →B and the area vector →A:
Φ=→B⋅→A
Substituting the values:
→B=3^i+4^k
→A=25^i+25^k
Calculating the dot product:
Φ=(3^i+4^k)⋅(25^i+25^k)=(3⋅25)+(4⋅25)=75+100=175Weber
Final Answer
The magnetic flux through the loop ABCDEFA is 175Weber.
---
The coordinates of the loop ABCDEFA are:
- A (0, 0, 0)
- B (5, 0, 0)
- C (5, 5, 0)
- D (0, 5, 0)
- E (0, 5, 5)
- F (0, 0, 5)
The loop ABCD lies in the xy-plane, forming a square. The side length of the square is 5 units (from A to B or A to D).
A=side2=52=25square units
The area vector →A for the loop ABCD is perpendicular to the plane of the loop. Since the loop lies in the xy-plane, the area vector points in the positive z-direction:
→AABCD=25^k
→AADEF=25^i
The total area vector →A for the entire loop ABCDEFA is the sum of the area vectors of the two sections:
→A=→AABCD+→AADEF=25^k+25^i
The magnetic flux Φ through the loop is given by the dot product of the magnetic field →B and the area vector →A:
Φ=→B⋅→A
Substituting the values:
→B=3^i+4^k
→A=25^i+25^k
Φ=(3^i+4^k)⋅(25^i+25^k)=(3⋅25)+(4⋅25)=75+100=175Weber
The magnetic flux through the loop ABCDEFA is 175Weber.
Topper's Solved these Questions
Similar Questions
A loop made of straight edges has six corners at A(0,0,0),B(L,O,0),C(L,L,0),D(0,L,0),E(0,L,L) and F(0,0,L). Where L is in meter. A magnetic field B=B0(ˆi+ˆk)T is present in the region. The flux passing through the loop ABCDEFA (in that order) is
View SolutionA loop made of straight edegs has six corners at A(0,0,0),B(L,O,0)C(L,L,0),D(0,L,0)E(0,L,L) and F(0,0,L). Where L is in meter. A magnetic field B=B0(ˆi+ˆk)T is present in the region. The flux passing through the loop ABCDEFA (in that order) is
View SolutionA loop made of straight edegs has six corners at A(0,0,0),B(L,O,0)C(L,L,0),D(0,L,0)E(0,L,L) and F(0,0,L). Where L is in meter. A magnetic field B=B0(ˆi+ˆk)T is present in the region. The flux passing through the loop ABCDEFA (in that order) is
View SolutionA loop made of straight edegs has six corners at A(0,0,0),B(L,O,0)C(L,L,0),D(0,L,0)E(0,L,L) and F(0,0,L). Where L is in meter. A magnetic field B=B0(ˆi+ˆk)T is present in the region. The flux passing through the loop ABCDEFA (in that order) is
View SolutionA loop A(0,0,0)B(5,0,0)C(5,5,0)D(0,5,0)E(0,5,10) and F(0,0,10) of straight edges has six corner points and two sides. The magnetic field in this region is →B=(3ˆi+4ˆk) T. The quantity of flux through the loop ABCDEFA (in Wb) is
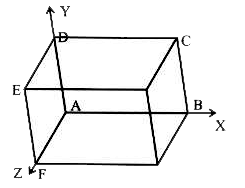
View SolutionA loop A(0,0,0)B(5,0,0)C(5,5,0)D(0,5,0)E(0,5,10) and F(0,0,10) of straight edges has six corner points and two sides. The magnetic field in this region is →B=(3ˆi+4ˆk) T. The quantity of flux through the loop ABCDEFA (in Wb) is

View SolutionA loop ABCA of straight edges has three corner points A (8, 0, 0), B (0, 8, 0 ) and C (0, 0, 8). The magnetic field in this region is →B=5(ˆi+ˆj+ˆk)T0 . The quantity of flux through the loop ABCA (in Wb) is _____.
View SolutionA loop ABCA of straight edges has three corner points A (8, 0, 0), B (0, 8, 0 ) and C (0, 0, 8). The magnetic field in this region is →B=5(ˆi+ˆj+ˆk)T0 . The quantity of flux through the loop ABCA (in Wb) is _____.
View SolutionFind the radius of the sphere through the points (0,5,0),(4,3,0),(4,0,3)and(0,4,3)
View SolutionIf A=⎡⎢⎣234046589⎤⎥⎦ and B=⎡⎢⎣305532047⎤⎥⎦ , find 3A−2B .
View Solution
