Let P be the set of points (x,y) such that x2≤y≤–2x+3. Then area of region bounded by points in set P is
293
343
323
313
The correct Answer is:C
To find the area of the region bounded by the points in the set P defined by the inequalities x2≤y≤−2x+3, we will follow these steps:
Step 1: Identify the curves
We have two equations to consider:
1. y=x2 (a parabola opening upwards)
2. y=−2x+3 (a straight line)
Step 2: Find the points of intersection
To find the area between these two curves, we first need to determine where they intersect. We set the equations equal to each other:
x2=−2x+3
Rearranging gives us:
x2+2x−3=0
Now, we can factor this quadratic equation:
(x−1)(x+3)=0
Thus, the solutions are:
x=1andx=−3
Step 3: Set up the integral for the area
The area A between the curves from x=−3 to x=1 can be calculated using the integral:
A=∫1−3((−2x+3)−(x2))dx
This simplifies to:
A=∫1−3(3−2x−x2)dx
Step 4: Compute the integral
Now we will compute the integral:
A=∫1−3(3−2x−x2)dx
Calculating the integral term by term:
1. The integral of 3 is 3x.
2. The integral of −2x is −x2.
3. The integral of −x2 is −x33.
Thus, we have:
A=(3x−x2−x33)1−3
Step 5: Evaluate the definite integral
Now we will evaluate this from −3 to 1:
1. For x=1:
3(1)−(1)2−(1)33=3−1−13=2−13=63−13=53
2. For x=−3:
3(−3)−(−3)2−(−3)33=−9−9+9=−9
Now we compute:
A=(53)−(−9)=53+9=53+273=323
Final Answer
Thus, the area of the region bounded by the points in set P is:
323
We have two equations to consider:
1. y=x2 (a parabola opening upwards)
2. y=−2x+3 (a straight line)
To find the area between these two curves, we first need to determine where they intersect. We set the equations equal to each other:
x2=−2x+3
Rearranging gives us:
x2+2x−3=0
Now, we can factor this quadratic equation:
(x−1)(x+3)=0
Thus, the solutions are:
x=1andx=−3
The area A between the curves from x=−3 to x=1 can be calculated using the integral:
A=∫1−3((−2x+3)−(x2))dx
This simplifies to:
A=∫1−3(3−2x−x2)dx
Now we will compute the integral:
A=∫1−3(3−2x−x2)dx
Calculating the integral term by term:
1. The integral of 3 is 3x.
2. The integral of −2x is −x2.
3. The integral of −x2 is −x33.
A=(3x−x2−x33)1−3
Now we will evaluate this from −3 to 1:
1. For x=1:
3(1)−(1)2−(1)33=3−1−13=2−13=63−13=53
2. For x=−3:
3(−3)−(−3)2−(−3)33=−9−9+9=−9
A=(53)−(−9)=53+9=53+273=323
Thus, the area of the region bounded by the points in set P is:
323
Topper's Solved these Questions
- JEE MAINBOOK - JEE MAINS PREVIOUS YEAR ENGLISHCHAPTER - JEE MAINEXERCISE - MATHEMATICS
121 Videos
- INVERSE TRIGONOMETRIC FUNCTIONSBOOK - JEE MAINS PREVIOUS YEAR ENGLISHCHAPTER - INVERSE TRIGONOMETRIC FUNCTIONSEXERCISE - All Questions
2 Videos
- JEE MAINSBOOK - JEE MAINS PREVIOUS YEAR ENGLISHCHAPTER - JEE MAINSEXERCISE - All Questions
445 Videos
Similar Questions
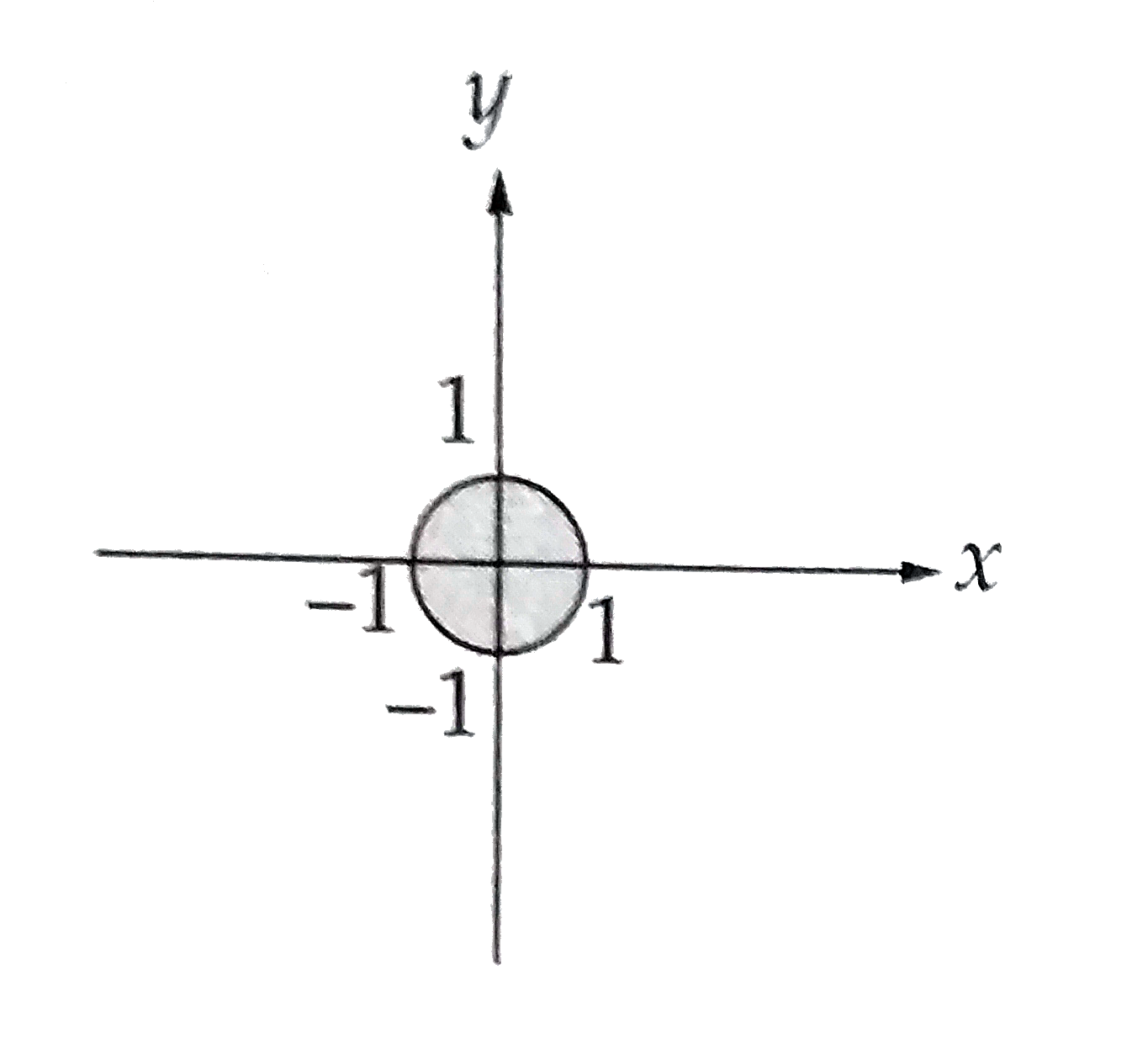
The shaed region represents the set C of all points (x, y) such that x2+y2≤1. The transformation T maps the point (x, y) to the point (2x, 4y). Which of the following shows the mapping of the set C by the transformation T ?
View SolutionThe area of the region on place bounded by max (|x|,|y|)≤12 is
View SolutionFind the equation of the set of all points P(x,y) such that the line OP is coincident with the line joining P and the point (3,2).
View SolutionThe area of the region R={(x,y)/x2≤y≤x} is
View SolutionThe area of the region bounded by x2+y2−2x−3=0andy=|x|+1 is
View SolutionFind the area of the region {(x,y):x2+y2≤1≤x+y}
View SolutionFind the area of the region {(x,y)/x2−x−1≤y≤−1}
View SolutionThe area bounded by 4x2≤y≤8x+12 is -
View SolutionFor points P≡(x1,y1) and Q≡(x2,y2) of the coordinates plane, a new distance d (P,Q) is defined by d(P,Q)=|x1−x2|+|y1−y2|. Let O≡(0,0) and A≡(3,2). Consider the set of points P in the first quadrant which are equidistant (with respect to the new distance) from O and A.
The area of the ragion bounded by the locus of P and the line y=4 in the first quadrant is
View SolutionA point P(x,y) moves that the sum of its distance from the lines 2x-y-3=0 and x+3y+4=0 is 7. The area bounded by locus P is (in sq. unit)
View Solution
