Efficiency of cyclic process is if heat , , , then is unknown then find the value of .
Option1 1080 J
Option2 -980 J
Option3 -1080J
Option4 -1280J
The correct Answer is:B
To solve the problem, we need to find the value of Q4 given the efficiency of a cyclic process is 50%, and the values of Q1=1915J, Q2=−40J, and Q3=125J.
1. Understanding Efficiency:
The efficiency η of a cyclic process is defined as:
η=WQsupply
where W is the work done and Qsupply is the total heat supplied to the system.
2. Identifying Heat Supplied and Rejected:
In this case, the heat supplied Qsupply is the sum of Q1 and Q3:
Qsupply=Q1+Q3=1915J+125J=2040J
The heat rejected Qrejected is the sum of Q2 and Q4:
Qrejected=Q2+Q4=−40J+Q4
3. Setting Up the Efficiency Equation:
Given that the efficiency is 50% or 12:
WQsupply=12
Rearranging gives:
W=12Qsupply=12×2040J=1020J
4. Relating Work Done to Heat Supplied and Rejected:
The work done can also be expressed as:
W=Qsupply−Qrejected
Substituting the expressions we have:
1020J=2040J−(−40J+Q4)
Simplifying this gives:
1020J=2040J+40J−Q4
1020J=2080J−Q4
Rearranging to find Q4:
Q4=2080J−1020J=1060J
5. Final Calculation:
Since Q4 is heat rejected, we need to express it as a negative value:
Q4=−1060J
Conclusion:
The value of Q4 is −1060J.
The efficiency η of a cyclic process is defined as:
η=WQsupply
where W is the work done and Qsupply is the total heat supplied to the system.
In this case, the heat supplied Qsupply is the sum of Q1 and Q3:
Qsupply=Q1+Q3=1915J+125J=2040J
The heat rejected Qrejected is the sum of Q2 and Q4:
Qrejected=Q2+Q4=−40J+Q4
Given that the efficiency is 50% or 12:
WQsupply=12
Rearranging gives:
W=12Qsupply=12×2040J=1020J
The work done can also be expressed as:
W=Qsupply−Qrejected
Substituting the expressions we have:
1020J=2040J−(−40J+Q4)
Simplifying this gives:
1020J=2040J+40J−Q4
1020J=2080J−Q4
Rearranging to find Q4:
Q4=2080J−1020J=1060J
Since Q4 is heat rejected, we need to express it as a negative value:
Q4=−1060J
The value of Q4 is −1060J.
Topper's Solved these Questions
Similar Questions
Q find the value of:- (−4)÷(23)
View SolutionA heat engine is involved with exchange of heat of 1915 J , - 40 J , + 125 J and -QJ , during one cycle achieving an efficiency of 50.0% The value of Q is:
View SolutionAn ideal gas is taken through a cyclic thermodynamic process through four steps. The amounts of heat involved in these steps are Q1=5960J, Q2=−5585J, Q3=−2980J and Q4=3645J respectively. The corresponding quantities of work involved are W1=2200J, W2=−825J, W3=−1100J and W4 respectively.
(a) Find the value of W4.
(b) What is the efficiency of the cycle?
View SolutionAn ideal gas is taken through a cyclic thermodynamic process through four steps. The amounts of heat involved in these steps are Q1=5960J,Q2=−5585J,Q3=−2980JandQ4=3645J, respectively. The corresponding quantities of work involved are W1=2200J,W2=−825J,W3=−1100JandW4 respectively.
(1) Find the value of W4.
(2) What is the efficiency of the cycle
View SolutionAn ideal gas is taken through a cyclic thermodynamic process through four steps. The amounts of heat involved in these steps are Q1=5960J,Q2=−5585J,Q3=−2980JandQ4=3645J, respectively. The corresponding quantities of work involved are W1=2200J,W2=−825J,W3=−1100JandW4 respectively.
(1) Find the value of W4.
(2) What is the efficiency of the cycle
View SolutionAn ideal gas is taken through a cyclic thermodynamics process through four steps. The amount of heat given to the system in these steps are Q1=1000J, Q2=−800J, Q3=450J, Q4=−200J respectively. The efficiency of cycle is nearly
View SolutionA gas is taken through a cyclic process ABCA as shown in, if 2.4 cal of heat is given in the process, what is the value of J ?
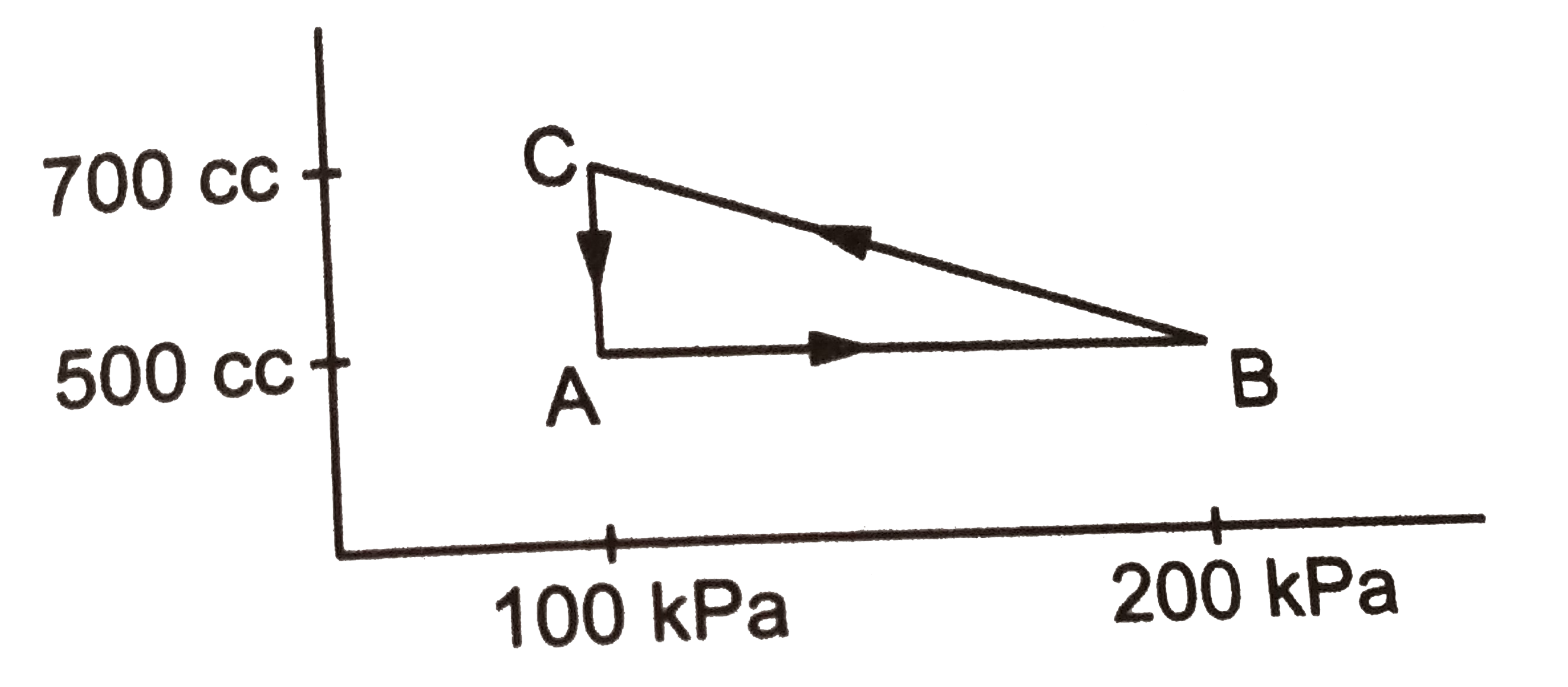
View SolutionA gas is taken through a cyclic process ABCA as shown in, if 2.4cal of heat is given in the process, what is the value of J ?

View SolutionIf p = 4,q = 3 and r = -2, find the values of :
3pq+2qr2p+q−r
View SolutionIf p = 4,q = -3 and r = 2, find the value of :p3+q3−r3−3pqr
View Solution
