An inductive coil has a reactance of . When an AC signal of frequency 1000 Hz is applied to the coil, the voltage leads the current by . What is the inductance of the coil?
H
H
H
H
The correct Answer is:D
To find the inductance of the coil, we can follow these steps:
Step 1: Understand the relationship between reactance and phase angle
In an LR circuit, the phase difference (φ) between the voltage and current is given by the formula:
tan(ϕ)=XLR
where XL is the inductive reactance and R is the resistance.
Step 2: Use the given phase angle
The problem states that the voltage leads the current by 45∘. Therefore:
tan(45∘)=1
This implies:
XLR=1⟹XL=R
Step 3: Use the given reactance value
We know that the reactance XL is given as 100Ω. Therefore:
XL=100Ω⟹R=100Ω
Step 4: Calculate the impedance
The impedance Z in an LR circuit is given by:
Z=√R2+X2L
Substituting the values we have:
Z=√1002+1002=√10000+10000=√20000=100√2Ω
Step 5: Relate reactance to inductance
The inductive reactance XL is also related to the inductance L and frequency f by the formula:
XL=2πfL
Substituting XL=100Ω and f=1000Hz:
100=2π(1000)L
Step 6: Solve for inductance L
Rearranging the equation to solve for L:
L=1002π×1000
Calculating this gives:
L=1002000π=120πH
Step 7: Convert to a numerical value
Using π≈3.14:
L≈120×3.14≈162.8≈0.0159H≈1.59×10−2H
Final Answer
Thus, the inductance of the coil is approximately:
L≈1.59×10−2H or 15.9mH
In an LR circuit, the phase difference (φ) between the voltage and current is given by the formula:
tan(ϕ)=XLR
where XL is the inductive reactance and R is the resistance.
The problem states that the voltage leads the current by 45∘. Therefore:
tan(45∘)=1
This implies:
XLR=1⟹XL=R
We know that the reactance XL is given as 100Ω. Therefore:
XL=100Ω⟹R=100Ω
The impedance Z in an LR circuit is given by:
Z=√R2+X2L
Substituting the values we have:
Z=√1002+1002=√10000+10000=√20000=100√2Ω
The inductive reactance XL is also related to the inductance L and frequency f by the formula:
XL=2πfL
Substituting XL=100Ω and f=1000Hz:
100=2π(1000)L
Rearranging the equation to solve for L:
L=1002π×1000
Calculating this gives:
L=1002000π=120πH
Using π≈3.14:
L≈120×3.14≈162.8≈0.0159H≈1.59×10−2H
Thus, the inductance of the coil is approximately:
L≈1.59×10−2H or 15.9mH
Topper's Solved these Questions
Similar Questions
An ideal inductive coil has a resistance of 100Ω When an ac signal of frequency 1000Hz is applied to the coil the voltage leads the current by 45∘ The inductance of the coil is .
View SolutionWhen an AC signal of frequency 1kHz is applied across a coil of resistance 100Ω, then the applied voltage leads the current by 45∘. The inductance of the coil is
View SolutionMagnetic flux of 5 microweber is linked with a coil, when a current of 1mA flows through it. What is the self-inductance of the coil?
View SolutionA coil has inductance of 115πH and is joined in series with a resistance of 220Ω. When an alternating emf of 220V at 50Hz is applied to it, then the wattless component of the current in the circuit is
View SolutionWhen 100 voltdc is applied across a coil, a current of 1amp flows through it, when 100V ac of 50Hz is applied to the same coil, only 0.5 amp flows. Calculate the resistance and inductance of the coil.
View SolutionA choke coil of resistance 5Ω and inductance 0.6 H is in series with a capacitance of 10(μ)F. If a voltage of 200 V is applied and the frequency is adjusted to resonance, the current and voltage across the inductance and capacitance are (I0),(V0)and(V1) respectively. we have
View SolutionA coil of resistance 40Ω is connected across a 4.0 V battery. 0.10 s after the battery is connected, the current in the coil is 63 mA. Find the inductance of the coil.
View SolutionA coil has resistance 30Ω and inductive reactance 20Ω at 50 Hz frequency. If an ac source of 200V, 100 Hz is connected across the coil, the current in the coil will be
View SolutionA coil having a resistance of 5 Ω and an inductance of 0.02H is arranged in parallel with another coil having a resistance of 1Ω and an inductance of 0.08H. Calculate the power absorbed when a voltage of 100V at 50Hz is applied.
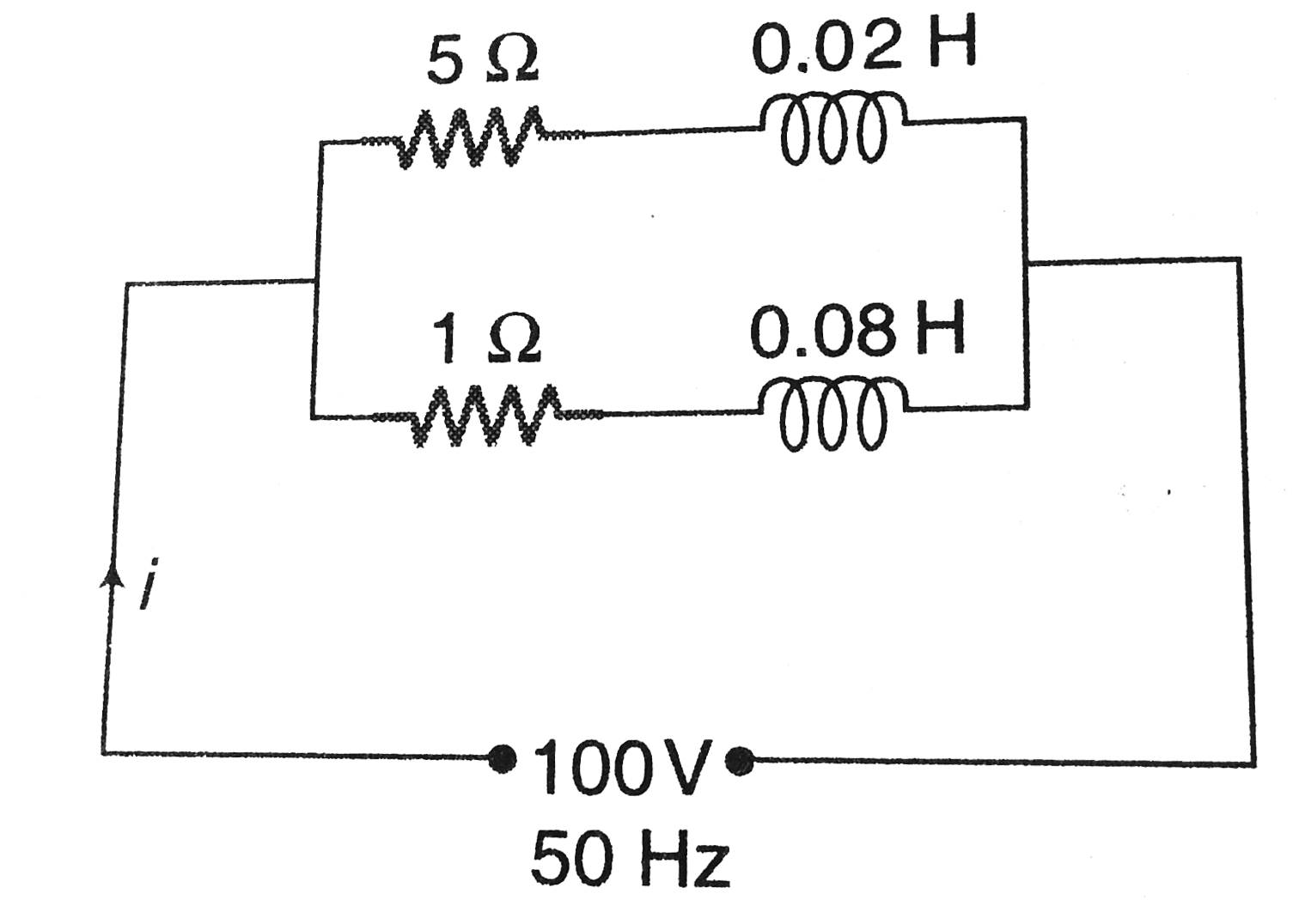 .
.
View SolutionAn LCR series circuit consists of a resistance of a 10Ω a capacitance of reactance 60Ω and an inductor coil The circuit is found to resonate when put across a 300V,100 Hz supply The inductance of the coil is (takenπ=3) .
View Solution
