A choke coil of resistance 5Ω and inductance 0.6 H is in series with a capacitance of 10(μ)F. If a voltage of 200 V is applied and the frequency is adjusted to resonance, the current and voltage across the inductance and capacitance are (I0),(V0)and(V1) respectively. we have
(I0)=40A
(V0)=9.8kV
(V1)=9.8kV
(V1)=19.6kV
The correct Answer is:A, B, C
To solve the problem, we will follow these steps:
Step 1: Determine the Current at Resonance
At resonance in an RLC circuit, the inductive reactance XL equals the capacitive reactance XC. The formula for the current I in the circuit is given by:
I=V√R2+(XC−XL)2
Since XC=XL at resonance, the formula simplifies to:
I0=VR
Given:
- Voltage V=200V
- Resistance R=5Ω
Substituting the values:
I0=2005=40A
Step 2: Calculate the Inductive Reactance XL
The inductive reactance XL is given by:
XL=ωL
Where ω=2πf. To find XL, we first need to calculate the resonant frequency f.
Step 3: Calculate the Resonant Frequency f
The resonant frequency f is given by:
f=12π√LC
Given:
- Inductance L=0.6H
- Capacitance C=10μF=10×10−6F
Substituting the values:
f=12π√0.6×10×10−6
Calculating:
f≈12π√6×10−7≈12π×0.000775≈65Hz
Step 4: Calculate XL
Now, substituting f into the equation for XL:
XL=2πfL=2π(65)(0.6)≈245Ω
Step 5: Calculate the Voltage Across the Inductor V0
The voltage across the inductor V0 is given by:
V0=I0⋅XL
Substituting the values:
V0=40⋅245=9800V=9.8kV
Step 6: Calculate the Voltage Across the Capacitor V1
The capacitive reactance XC is given by:
XC=1ωC
Substituting ω and C:
XC=12π(65)(10×10−6)
Calculating XC:
XC≈12π(65)(10−5)≈245Ω
Now, the voltage across the capacitor V1 is:
V1=I0⋅XC=40⋅245=9800V=9.8kV
Final Results
- I0=40A
- V0=9.8kV
- V1=9.8kV
At resonance in an RLC circuit, the inductive reactance XL equals the capacitive reactance XC. The formula for the current I in the circuit is given by:
- Voltage V=200V
- Resistance R=5Ω
The inductive reactance XL is given by:
The resonant frequency f is given by:
- Inductance L=0.6H
- Capacitance C=10μF=10×10−6F
Now, substituting f into the equation for XL:
The voltage across the inductor V0 is given by:
The capacitive reactance XC is given by:
- I0=40A
- V0=9.8kV
- V1=9.8kV
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ALTERNATING CURRENTEXERCISE - Exercises Assertion-reasoning7videos
ALTERNATING CURRENT
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ALTERNATING CURRENTEXERCISE - Exercises Linked Comprehension25videos
ALTERNATING CURRENT
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ALTERNATING CURRENTEXERCISE - Exercises Single Correct63videos
ALTERNATING CURRENT
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ATOMIC PHYSICSEXERCISE - ddp.4.315videos
ATOMIC PHYSICS
Similar Questions
A choke coil of resistance R and inductance is connected to an ac. source of frequency f and peak voltage V If angular frequency will increase then, the average power dissipated in the choke
View SolutionIn a series resonant circuit, the ac, voltage across resistance R, inductance L and capacitance are 5V, 10V and 10V, respectively. The ac. voltage applied to the circuit will be
View SolutionA series LCR circuit containing a resistance of 120Ω has angular resonance frequency 4×105rads−1. At resonance the vlotage across resistance and inductance are 60V and 40 V, repectively,
The value of capacitance C isView SolutionA series LCR circuit containing a resistance of 120Ω has angular resonance frequency 4×105rads−1. At resonance the vlotage across resistance and inductance are 60V and 40 V, repectively,
the value of inductance L isView SolutionIn LC oscillation resistance is 100 Ω and inductance and capacitance is 1 H and 10μF . Find the half power of frequency .
View SolutionA series LCR circuit containing a resistance of 120 ohm has angular resonance frequency 4×103rads−1. At resonance, the voltage across resistance and inductance are 60V and 40 V respectively. The values of Land C are respectively
View SolutionA coil having a resistance of 5 Ω and an inductance of 0.02H is arranged in parallel with another coil having a resistance of 1Ω and an inductance of 0.08H. Calculate the power absorbed when a voltage of 100V at 50Hz is applied.
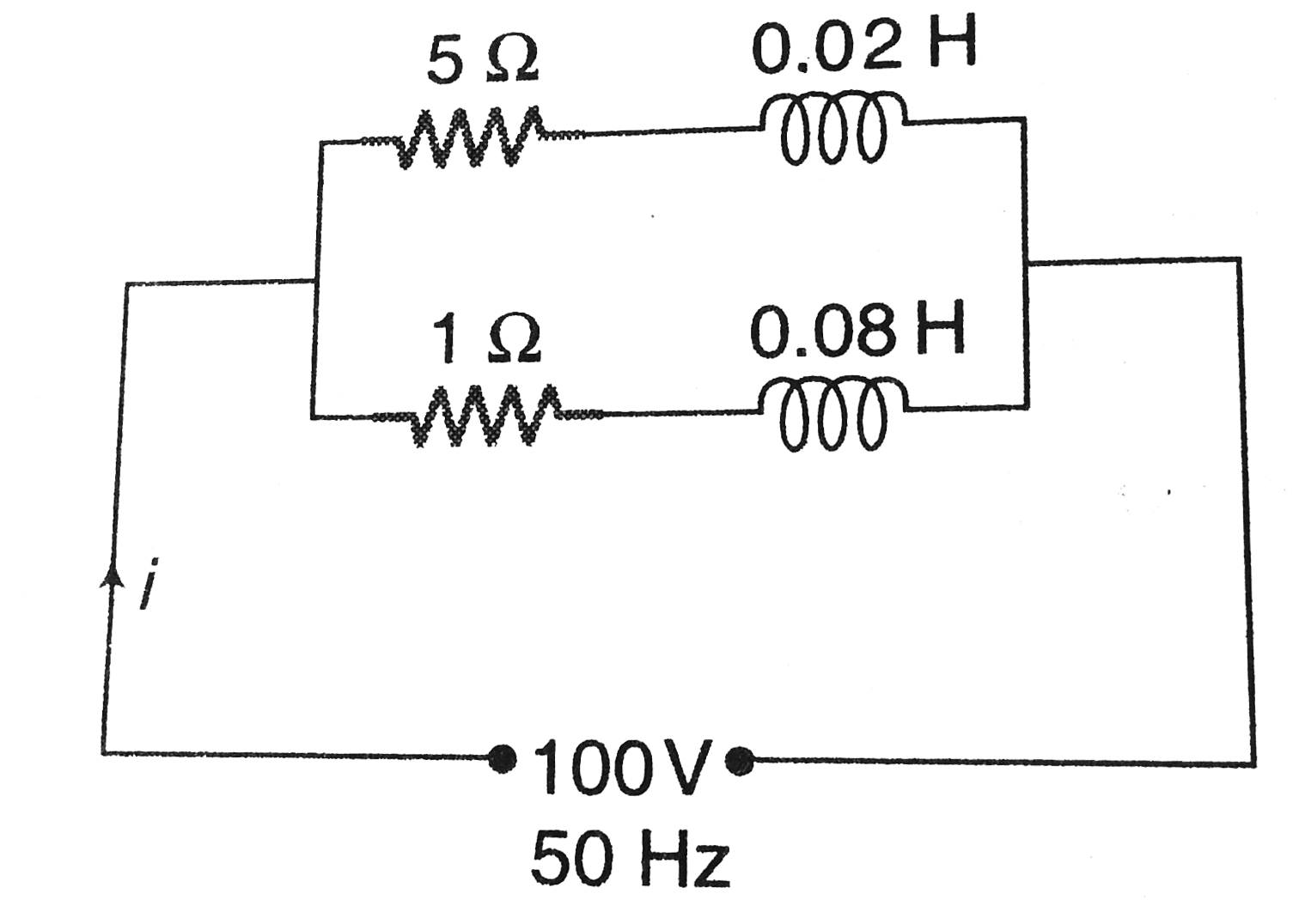 .View Solution
.View SolutionA capacitor of capacitance 100μF and a coil of resistance 50Ω and inductance 0.5H are connected in series with a110V,50HzAC source. Find the rms value of the current.
View SolutionIn a series L−C circuit, the applied voltage is V0. if omega is very low, then the voltage drop across the inductor VL and capacitor VC are
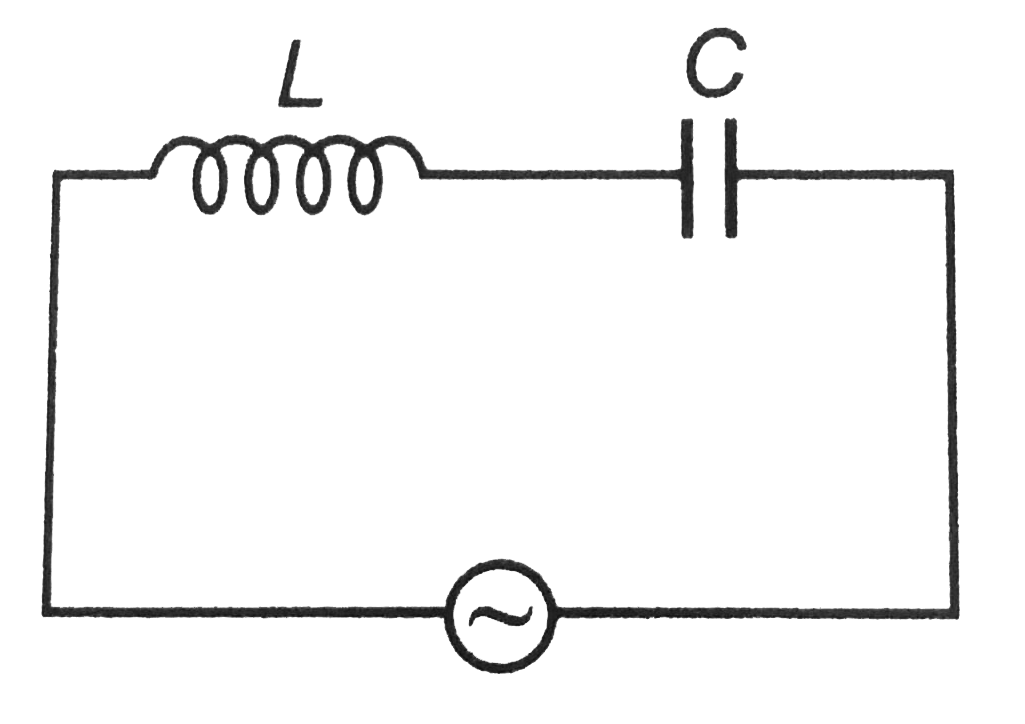 .View Solution
.View SolutionA coil of resistance 300Ω and inductance 1.0 henry is connected across an voltages source of frequency 300/2πHz. The phase difference between the voltage and current in the circuit is
View Solution

