Match the type of interaction in column A with the distance dependence of their interaction energy in column B :
AB(I)ion-ion(a)1r(II)dipole-dipole(b)1r2(III)London dispersion(c)1r3(d)1r6
(I)-(a), (II)-(b), (III)-(d)
(I)-(a), (II)-(b), (III)-(c)
(I)-(a), (II)-(c), (III)-(d)
(I)-(a), (II)-(c), (III)-(b)
To solve the problem of matching the types of interactions with their distance dependence of interaction energy, we can follow these steps:
Step 1: Understand the Types of Interactions
We have three types of interactions to consider:
1. Ion-Ion Interaction
2. Dipole-Dipole Interaction
3. London Dispersion Forces
Step 2: Recall the Distance Dependence of Each Interaction
- Ion-Ion Interaction: The energy of interaction between two ions is inversely proportional to the distance between them, which can be expressed as:
Ion-Ion Interaction∝1r
- Dipole-Dipole Interaction: The energy of interaction between two dipoles decreases with the cube of the distance between them:
Dipole-Dipole Interaction∝1r3
- London Dispersion Forces: The energy of interaction due to London dispersion forces varies inversely with the sixth power of the distance:
London Dispersion Forces∝1r6
Step 3: Match Each Interaction with the Correct Distance Dependence
Now, we can match the interactions from Column A with the corresponding expressions from Column B:
- Ion-Ion Interaction matches with (a) 1r
- Dipole-Dipole Interaction matches with (c) 1r3
- London Dispersion Forces matches with (d) 1r6
Step 4: Write the Final Matches
Based on the above analysis, we can summarize the matches as follows:
- (I) Ion-Ion → (a) 1r
- (II) Dipole-Dipole → (c) 1r3
- (III) London Dispersion → (d) 1r6
Final Answer
The correct matches are:
- I → a
- II → c
- III → d
We have three types of interactions to consider:
1. Ion-Ion Interaction
2. Dipole-Dipole Interaction
3. London Dispersion Forces
- Ion-Ion Interaction: The energy of interaction between two ions is inversely proportional to the distance between them, which can be expressed as:
Ion-Ion Interaction∝1r
- Dipole-Dipole Interaction: The energy of interaction between two dipoles decreases with the cube of the distance between them:
Dipole-Dipole Interaction∝1r3
London Dispersion Forces∝1r6
Now, we can match the interactions from Column A with the corresponding expressions from Column B:
- Ion-Ion Interaction matches with (a) 1r
- Dipole-Dipole Interaction matches with (c) 1r3
- London Dispersion Forces matches with (d) 1r6
Based on the above analysis, we can summarize the matches as follows:
- (I) Ion-Ion → (a) 1r
- (II) Dipole-Dipole → (c) 1r3
- (III) London Dispersion → (d) 1r6
The correct matches are:
- I → a
- II → c
- III → d
Topper's Solved these Questions
Similar Questions
Match the shape of molecules in Column 1 with the type of hybridisation in Column II.
Column IColumn II(a)Tetrahedral(i)sp2(b)Trigonal(ii)sp(c)Linear(iii)sp3(d)Trigonal bipyramidal(iv)sp3d
View SolutionMatch the column-1 with column-ii w.r.t. aestivation
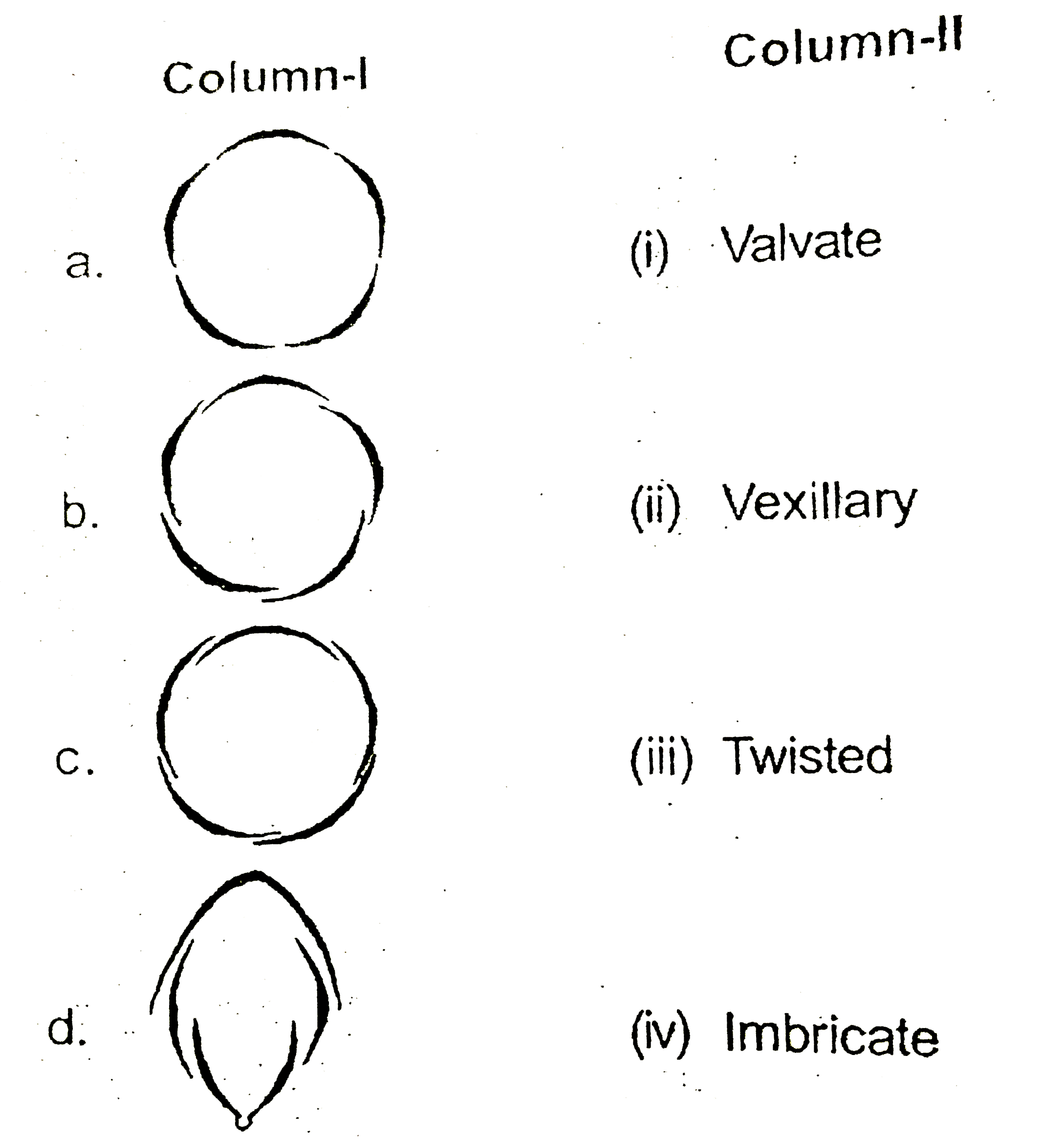
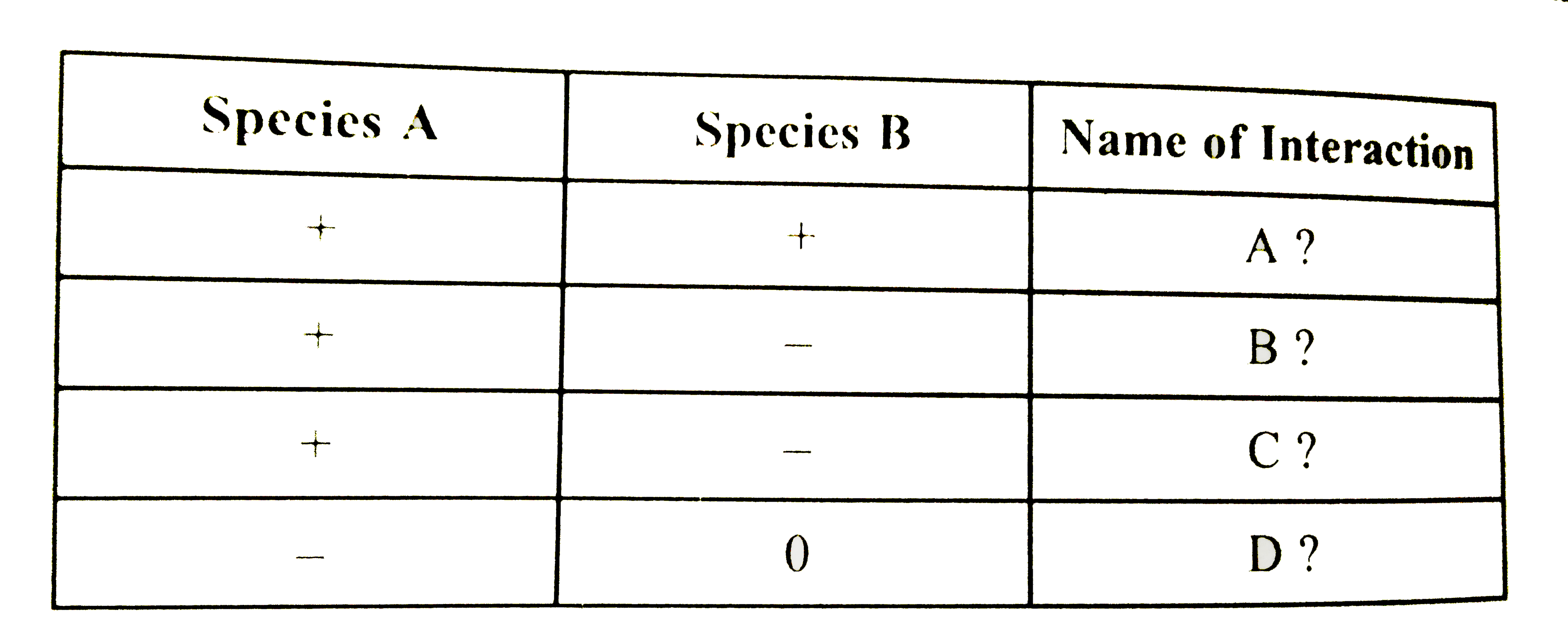
View SolutionWhat type of population interaction in A,B,C,D between the species A and B as per the tabular column given below .
View SolutionMatch the statement (A,B,C,D) in column I with the statement (p,q,r,s) in column II. The answers are to be properly bobbled.
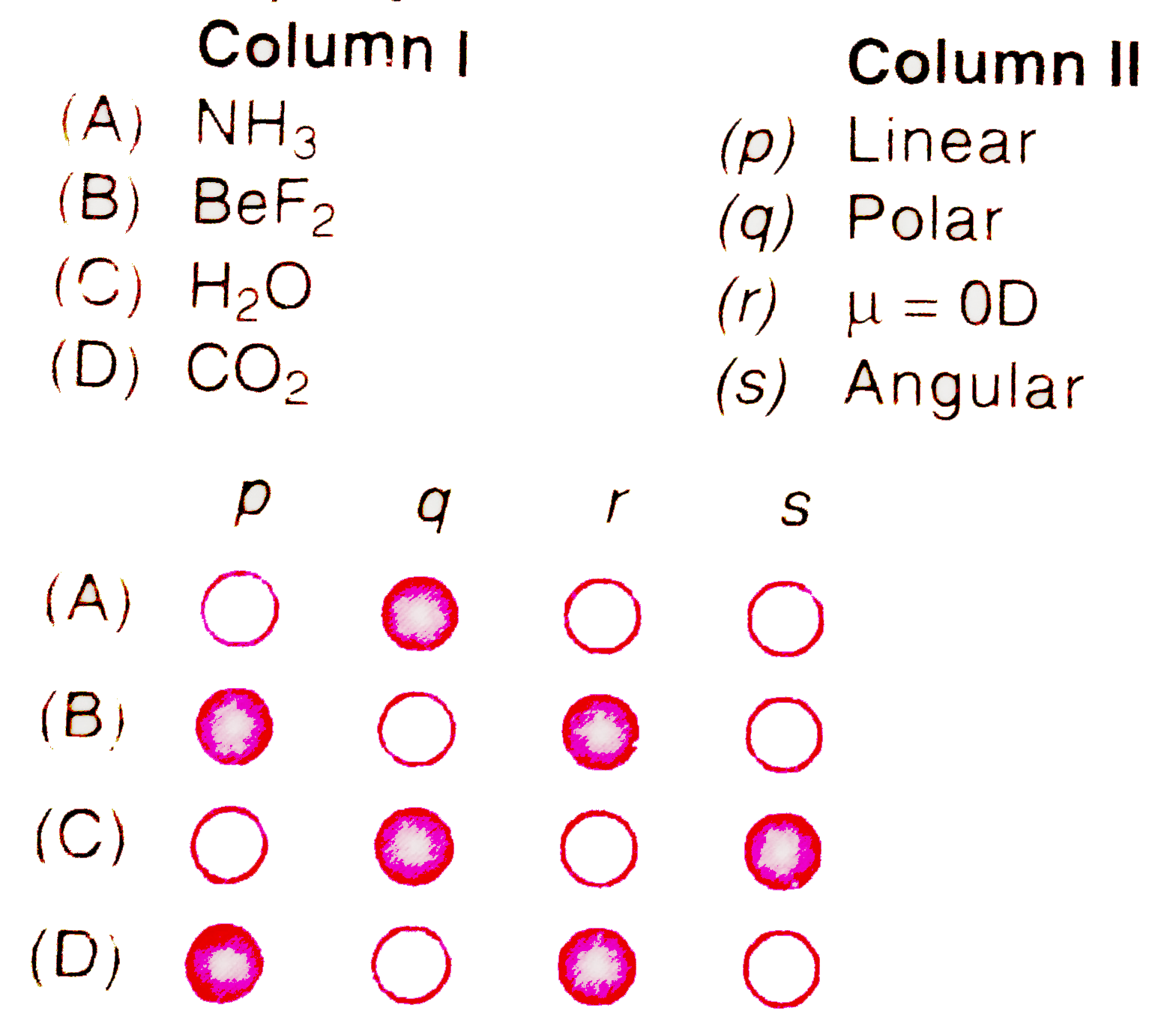
View SolutionName the types of interaction or intermolecular forces of which potential energy-distance function are given below
(a) E∝1r (b) E∝1r2 (c ) E∝1r3
(d) E∝1r4 (e) E∝1r6 .
View SolutionMatch the following columns. (for a satellite in circular orbit)
Column-IColumn-II(A)Kinetic energy(p)−GMm2r(B)Potential energy(q)√GMr(C)Total energy(r)−GMmr(D)Orbital speed(s)GMm2r
View SolutionMatch the columns
Column-AColumn-B(a)Ribs(i)26(b)Vertebrae(ii)14(c)Phalanges(iii)1(d)Sternum(iv)24
View SolutionMatch the statement (A, B, C, D) in Column I with the statements (p, q, r, s) in Column II. The answer are to be properly bubbled.
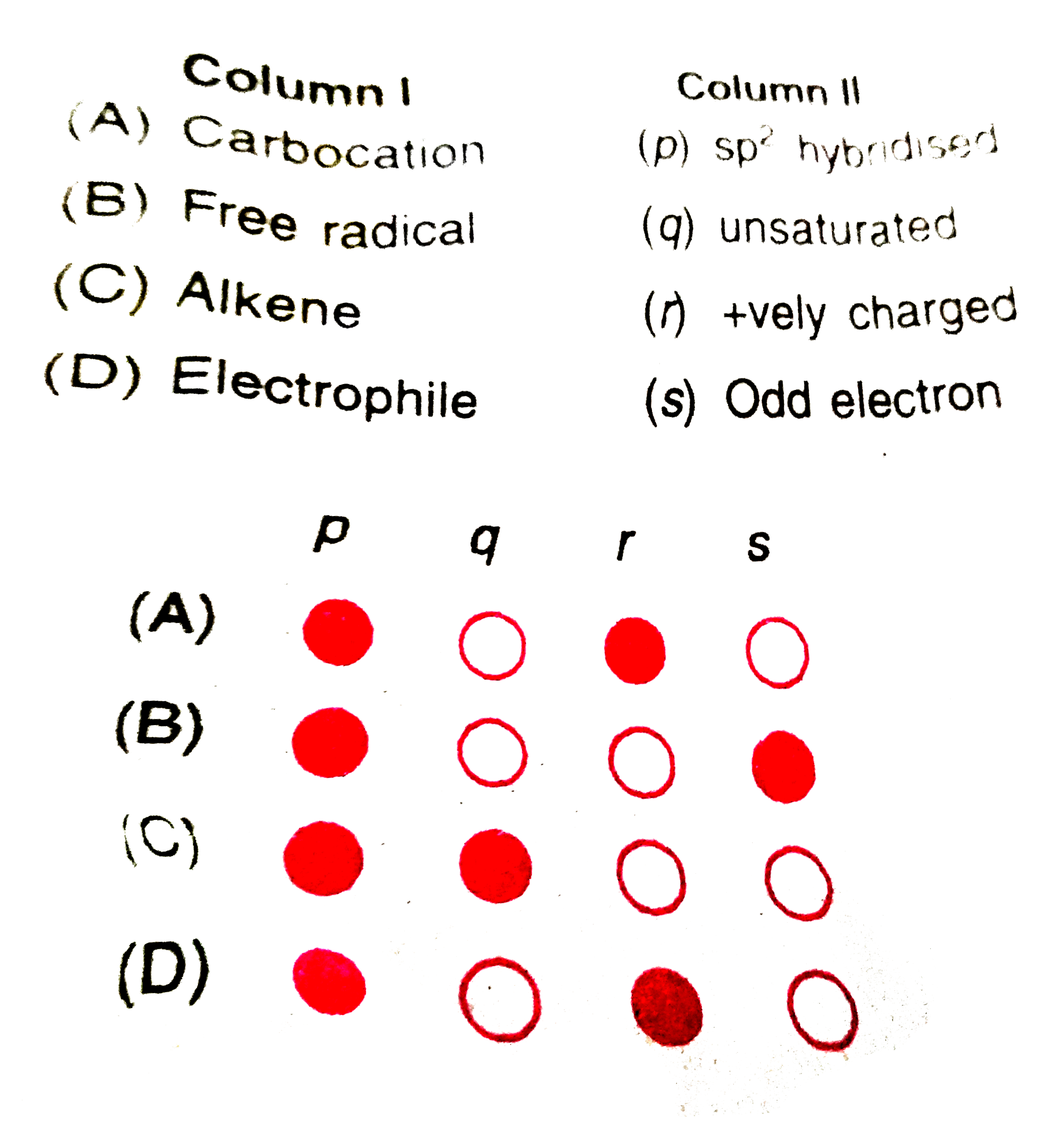
View SolutionMatch the statement (A, B, C, D) in Column I with the statements (p, q, r, s) in Column II. The answer are to be properly bubbled.
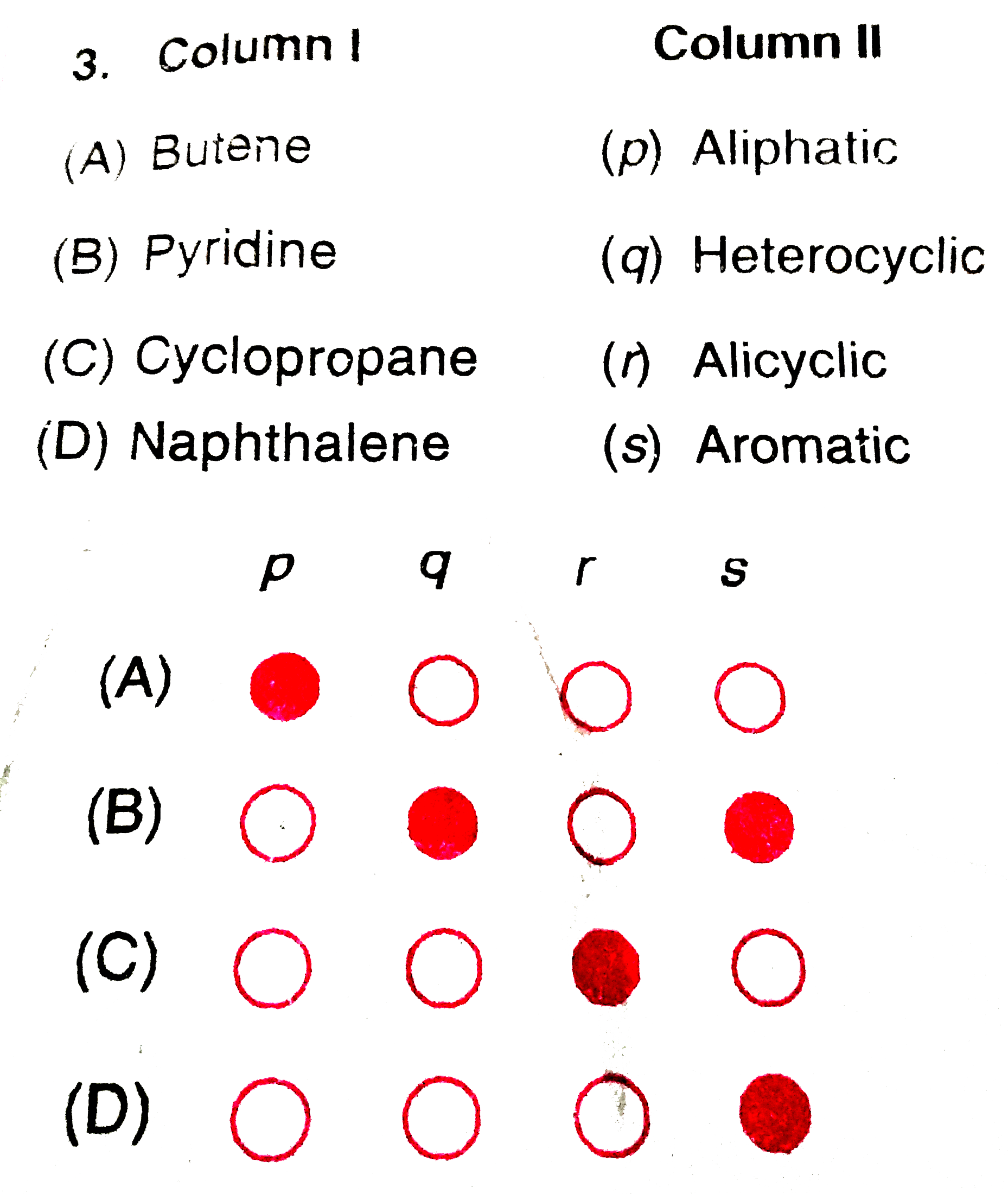
View SolutionMatch the statement (A, B, C, D) in Column I with the statements (p, q, r, s) in Column II. The answer are to be properly bubbled.
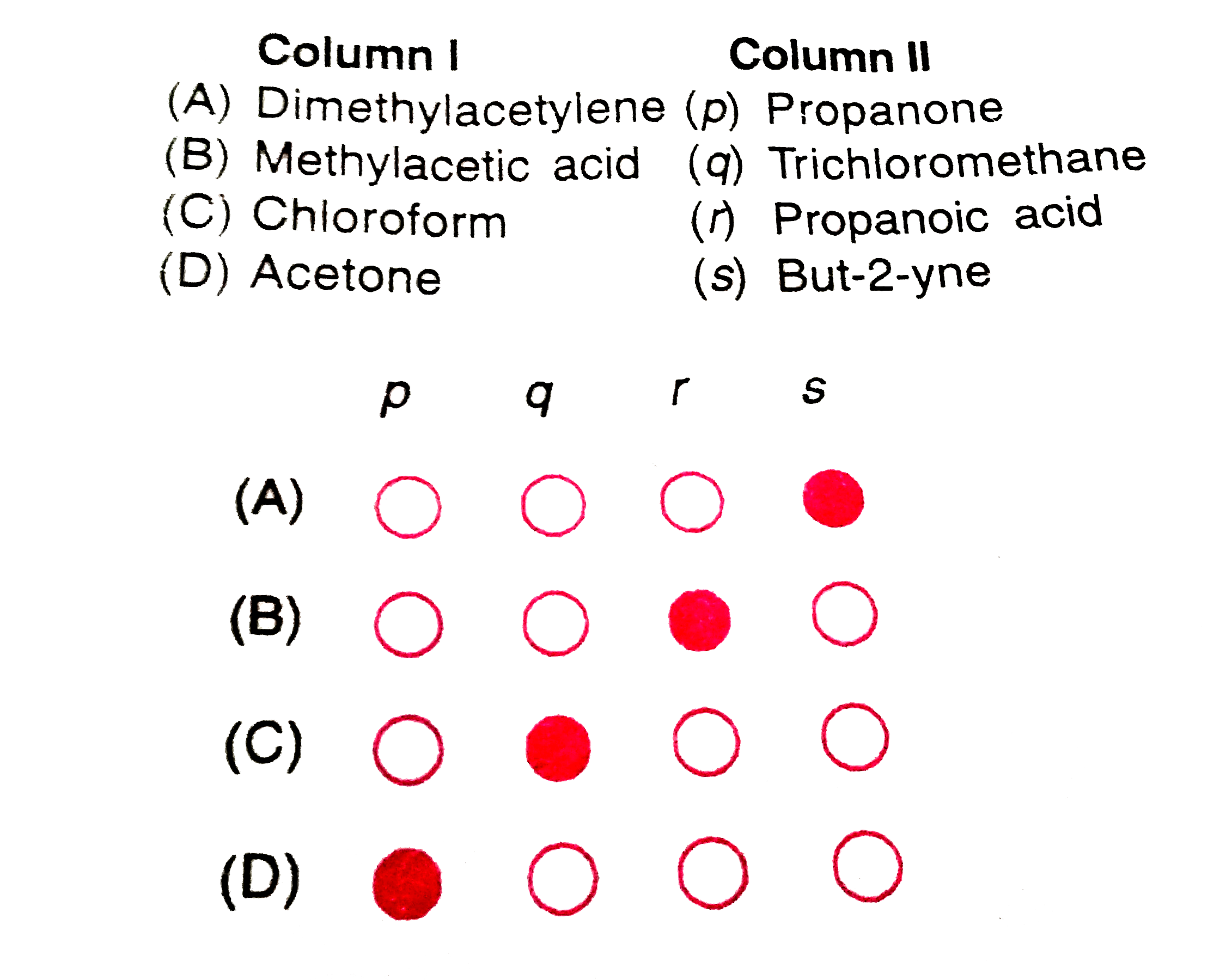
View Solution
