Match the following columns. (for a satellite in circular orbit)
Column-IColumn-II(A)Kinetic energy(p)−GMm2r(B)Potential energy(q)√GMr(C)Total energy(r)−GMmr(D)Orbital speed(s)GMm2r
The correct Answer is:(A→s,B→r,C→p,D→q)
To solve the matching question, we need to identify the correct formulas for each physical quantity related to a satellite in a circular orbit. Let's go through each item step by step.
Step 1: Identify Kinetic Energy (Column I - A)
The kinetic energy (KE) of a satellite in orbit is given by the formula:
KE=12mv2
Where v is the orbital speed. The orbital speed can be derived from the gravitational force acting as the centripetal force:
F=GMmr2=mv2r
From this, we can derive:
v2=GMr
Substituting this back into the kinetic energy formula gives:
KE=12m(GMr)=GMm2r
Thus, we can match:
- (A) Kinetic energy → (p) −GMm2r
Step 2: Identify Potential Energy (Column I - B)
The gravitational potential energy (PE) of a satellite at a distance r from the center of the Earth is given by:
PE=−GMmr
Thus, we can match:
- (B) Potential energy → (r) −GMmr
Step 3: Identify Total Energy (Column I - C)
The total mechanical energy (E) of the satellite in orbit is the sum of its kinetic and potential energy:
E=KE+PE
Substituting the values we found:
E=GMm2r−GMmr=−GMm2r
Thus, we can match:
- (C) Total energy → (s) −GMm2r
Step 4: Identify Orbital Speed (Column I - D)
The orbital speed v we derived earlier is:
v=√GMr
Thus, we can match:
- (D) Orbital speed → (q) √GMr
Final Matches
Now we can summarize the matches:
- (A) Kinetic energy → (p) −GMm2r
- (B) Potential energy → (r) −GMmr
- (C) Total energy → (s) −GMm2r
- (D) Orbital speed → (q) √GMr
The kinetic energy (KE) of a satellite in orbit is given by the formula:
KE=12mv2
Where v is the orbital speed. The orbital speed can be derived from the gravitational force acting as the centripetal force:
F=GMmr2=mv2r
From this, we can derive:
v2=GMr
Substituting this back into the kinetic energy formula gives:
KE=12m(GMr)=GMm2r
Thus, we can match:
- (A) Kinetic energy → (p) −GMm2r
The gravitational potential energy (PE) of a satellite at a distance r from the center of the Earth is given by:
PE=−GMmr
Thus, we can match:
- (B) Potential energy → (r) −GMmr
The total mechanical energy (E) of the satellite in orbit is the sum of its kinetic and potential energy:
E=KE+PE
Substituting the values we found:
E=GMm2r−GMmr=−GMm2r
Thus, we can match:
- (C) Total energy → (s) −GMm2r
The orbital speed v we derived earlier is:
v=√GMr
Thus, we can match:
- (D) Orbital speed → (q) √GMr
Now we can summarize the matches:
- (A) Kinetic energy → (p) −GMm2r
- (B) Potential energy → (r) −GMmr
- (C) Total energy → (s) −GMm2r
- (D) Orbital speed → (q) √GMr
Topper's Solved these Questions
- GRAVITATIONBOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - (C) Chapter Exercises
45 Videos
- GRAVITATIONBOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - (A) Chapter Exercises
68 Videos
- GENERAL PHYSICSBOOK - DC PANDEY ENGLISHCHAPTER - GENERAL PHYSICSEXERCISE - INTEGER_TYPE
2 Videos
- KINEMATICSBOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICSEXERCISE - INTEGER_TYPE
10 Videos
Similar Questions
Match the column I w.r.t column II

View SolutionMatch the column-1 with column-ii w.r.t. aestivation
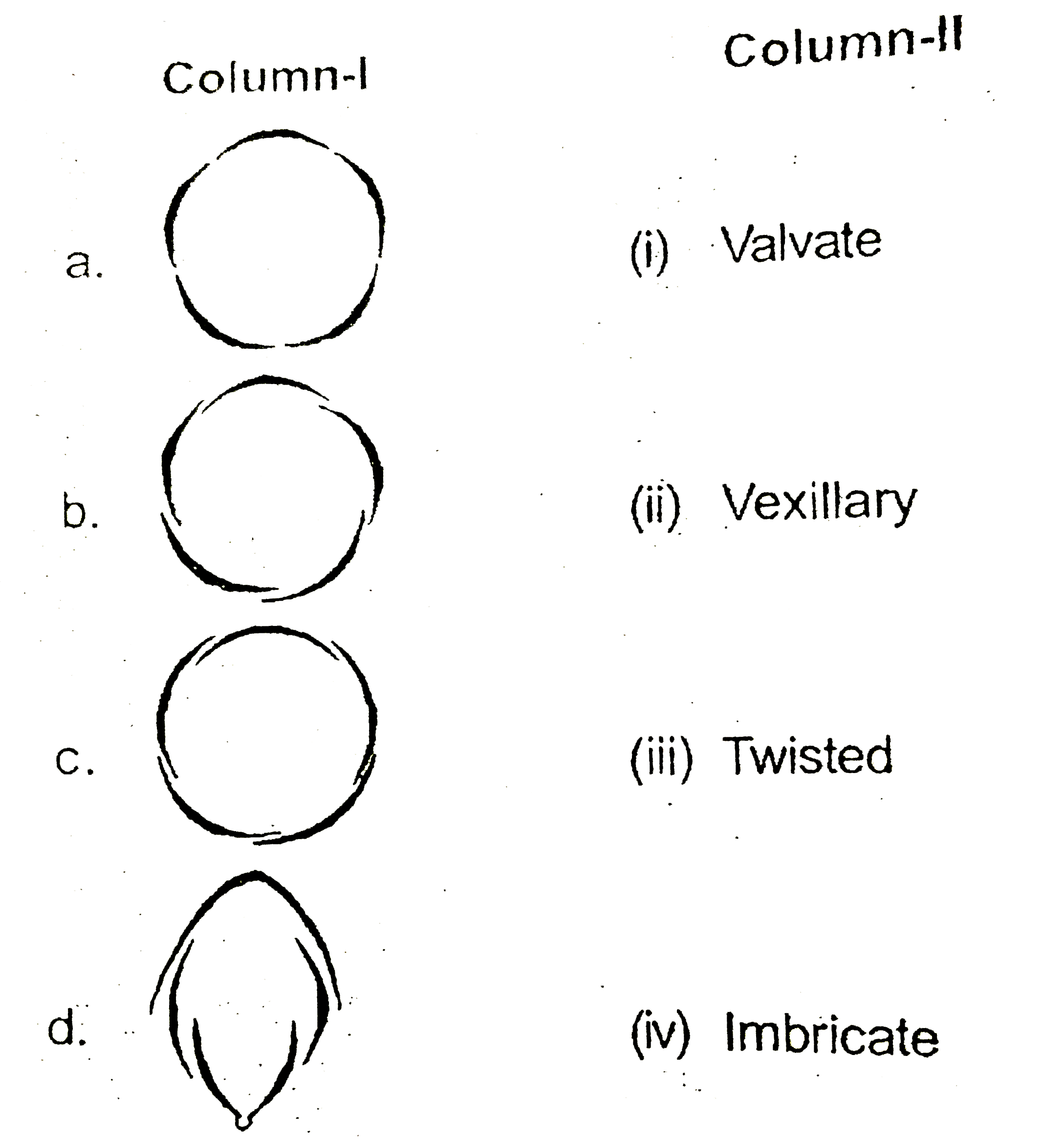
View SolutionIn elliptical orbit of a planet, as the planet moves from apogee position to perigee position to perigee position, match the following columns
Column-IColumn-II(A)Speed of planet(p)Remains same(B)Distance of planet from centre of sun(q)Decreases(C)Potential energy(r)Increase(D)Angular momentum about centre of sun(s)Cannot say
View SolutionMatch the column :
A particle at a distance r from the centre of a uniform spherical planet of mass M radius R(<r) has a velocity v magnitude of which is given in column I. Match trajectory from column II about possible nature of orbit.
Column IColumn II(A)0<V<√GMr(P)Straight line(B)√GMr(Q)Circle(C)√2GMr(R)Parabola(D)v>√2GMr(S)Ellipse
View SolutionIf earth decreases its rotational speed. Match the following columns
Column-IColumn-II(A)Value of g at pole(p)Will remain same(B)Value of g at equator(q)Will increase(C)Distance of geostationary(r)Will decrease(D)Energy of geostationary satellite(s)Cannot say
View SolutionMatch the Column-I with Column-II :
Column-IColumn-II(a)Mole fraction(p)mol kg−1(b)Molality(q)Depends on temperature(c)Molarity(r)Unitless(d)Normality(s)Independent of temperature
View SolutionMatch the following
Column-IColumn-II(A)Enthalpy(p)Extensive property(B)Entropy(q)H - TS(C)Free energy(r)E + PV(D)Pressure(s)Intensive property
View SolutionColumn IColumn II(A)R/L(p)Time(B)C/R(q)Frequency(C)E/B(r)Speed(D)√ε0μ0(s)None
View SolutionColumn IColumn II(A)Stress(p)Pressure(B)Strain(q)Energy density(C)Modulus of elasticity(r)Angle(D)Torque(s)Energy
View SolutionOn the surface of earth acceleration due gravity is g and gravitational potential is V. Match of following columns
Column-IColumn-II(A)At height h=R, value of g(p)Decreases by a factor14(B)At depth h=R2,value of g(q)Decrease by a factor12(C)At height h=R,value of V(r)Increase by a factor118(D)At height h=R2,value of V(s)Increase by a factor 2(t)None
View Solution
