Three forcesstart acting simultaneously on a particle moving with a velocity →v . These forces are represented in magnitude and direction by three sides of a triangle taken in the same order. What now will be the velocity of the particle ?
To solve the problem, we need to analyze the situation where three forces are acting on a particle that is already in motion. The forces are represented by the sides of a triangle, and we need to determine the effect of these forces on the particle's velocity.
1. Understanding the Forces:
- We have three forces, F1, F2, and F3, acting on a particle. These forces are arranged in such a way that they can be represented as the sides of a triangle. This means that the forces are in equilibrium.
2. Applying the Triangle Law of Forces:
- According to the triangle law of forces, if three forces acting at a point can be represented in magnitude and direction by the three sides of a triangle taken in order, then the resultant of these forces is zero. Therefore, we can write:
F1+F2+F3=0
3. Resultant Force Calculation:
- Since the resultant force is zero, we can conclude that:
F1+F2=−F3
- This indicates that the vector sum of the forces acting on the particle is zero.
4. Newton's Second Law of Motion:
- According to Newton's second law, the net force acting on an object is equal to the mass of the object multiplied by its acceleration:
Fnet=m⋅a
- Since the resultant force Fnet is zero, we have:
0=m⋅a
- This implies that the acceleration a of the particle is zero.
5. Conclusion about Velocity:
- If the acceleration is zero, it means that the velocity of the particle does not change. Therefore, the velocity of the particle remains constant.
- Since the particle was initially moving with a velocity →v, the final velocity →vfinal will be:
→vfinal=→v
Final Answer:
The velocity of the particle remains the same, which is →v.
---
- We have three forces, F1, F2, and F3, acting on a particle. These forces are arranged in such a way that they can be represented as the sides of a triangle. This means that the forces are in equilibrium.
- According to the triangle law of forces, if three forces acting at a point can be represented in magnitude and direction by the three sides of a triangle taken in order, then the resultant of these forces is zero. Therefore, we can write:
F1+F2+F3=0
- Since the resultant force is zero, we can conclude that:
F1+F2=−F3
- This indicates that the vector sum of the forces acting on the particle is zero.
- According to Newton's second law, the net force acting on an object is equal to the mass of the object multiplied by its acceleration:
Fnet=m⋅a
- Since the resultant force Fnet is zero, we have:
0=m⋅a
- This implies that the acceleration a of the particle is zero.
- If the acceleration is zero, it means that the velocity of the particle does not change. Therefore, the velocity of the particle remains constant.
- Since the particle was initially moving with a velocity →v, the final velocity →vfinal will be:
→vfinal=→v
The velocity of the particle remains the same, which is →v.
Topper's Solved these Questions
Similar Questions
If →a,→band→c are represented by three sides of equilateral triangle taken in same order.
P.T.→a+→b+→c=→0 .View SolutionWrite the expression for the magnetic force →F acting on a charged particle q moving with velocity →v in the presence of the magnetic field →B in a vector form.
Show that no work is done and no change in the magnitude of the velocity of the particle is produced by this force.View Solution
Knowledge Check
- Question 1 - Select One
Three forces start acting simultaneously on a particle moving with velocity, ¯v. These forces are respresented in magnitude and direction by the three sides of a triangle ABC. The particle will now move with velocity
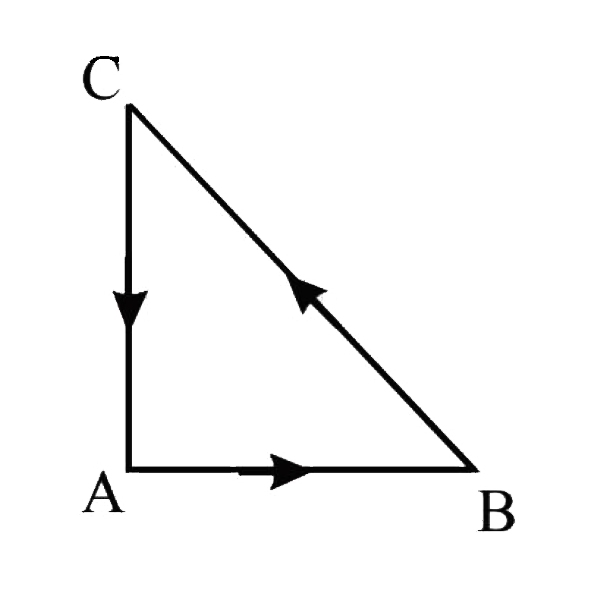 A(a) less than →vB(b) greater than →vC(c) |v| in the direction of the largest force BcD(d) →v, remaining unchanged
A(a) less than →vB(b) greater than →vC(c) |v| in the direction of the largest force BcD(d) →v, remaining unchanged - Question 2 - Select One
Three forces start acting simultaneously on a particle moving with velocity v. These forces are represented in magnitude and direction by the three sides of a triangle ABC (as shown). The particle will now move with velocity.
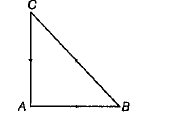 Aless thanBgreater than vCv In the direction of largest force SCDv remaining unchanged
Aless thanBgreater than vCv In the direction of largest force SCDv remaining unchanged - Question 3 - Select One
Three forces start acting simultaneously on a particle moving with velocity, ¯v. These forces are respresented in magnitude and direction by the three sides of a triangle ABC. The particle will now move with velocity
 A→v remaining unchangedBless than →vCgreater than →vD→v in the direction of the largest force BC
A→v remaining unchangedBless than →vCgreater than →vD→v in the direction of the largest force BC
Three forces start acting simultaneously on a particle moving the velocity →V. The forces are represented in magnitude and direction by the three sides of a triangle ABC (as shown). The particle will now move with velocity
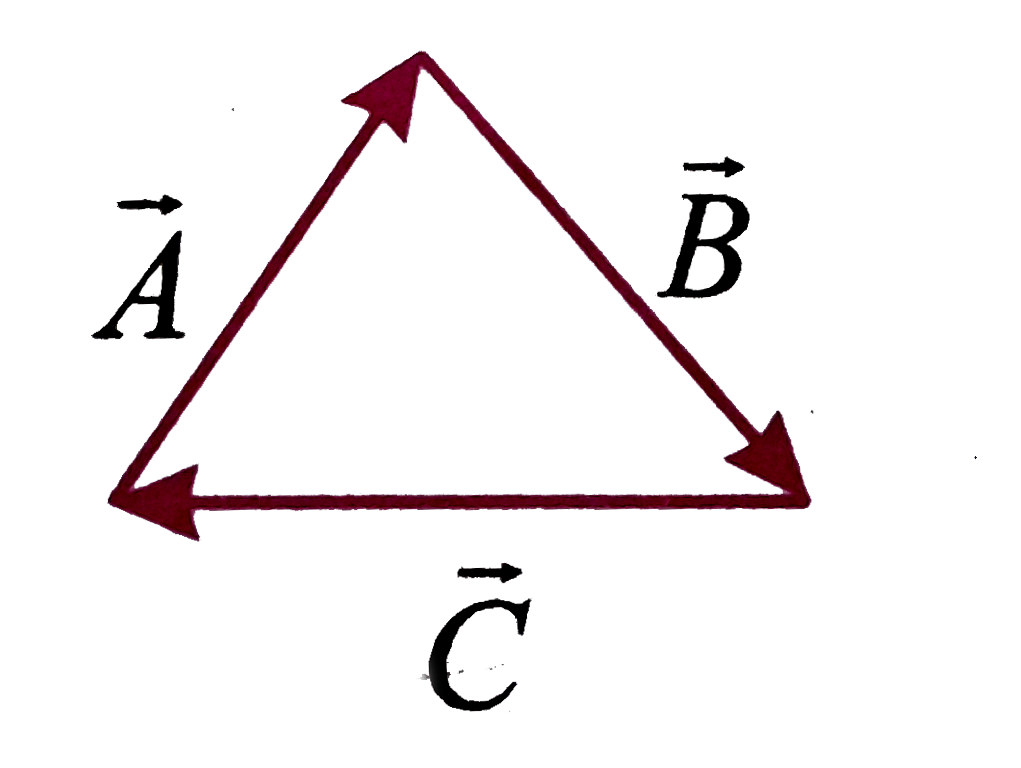 View Solution
View SolutionIf a system of coplanar force acting on a rigid body is represented in magnitude , direction and sence by the sides of a polygon taken in order , then the system is equivalent to
View SolutionThe force acting on a particle of charge q moving in a uniform magnets field with velocity v is
View SolutionA particle moving with velocity →V is acted by the three forces shown by the vector triangle PQR. The velocity of the particle will :
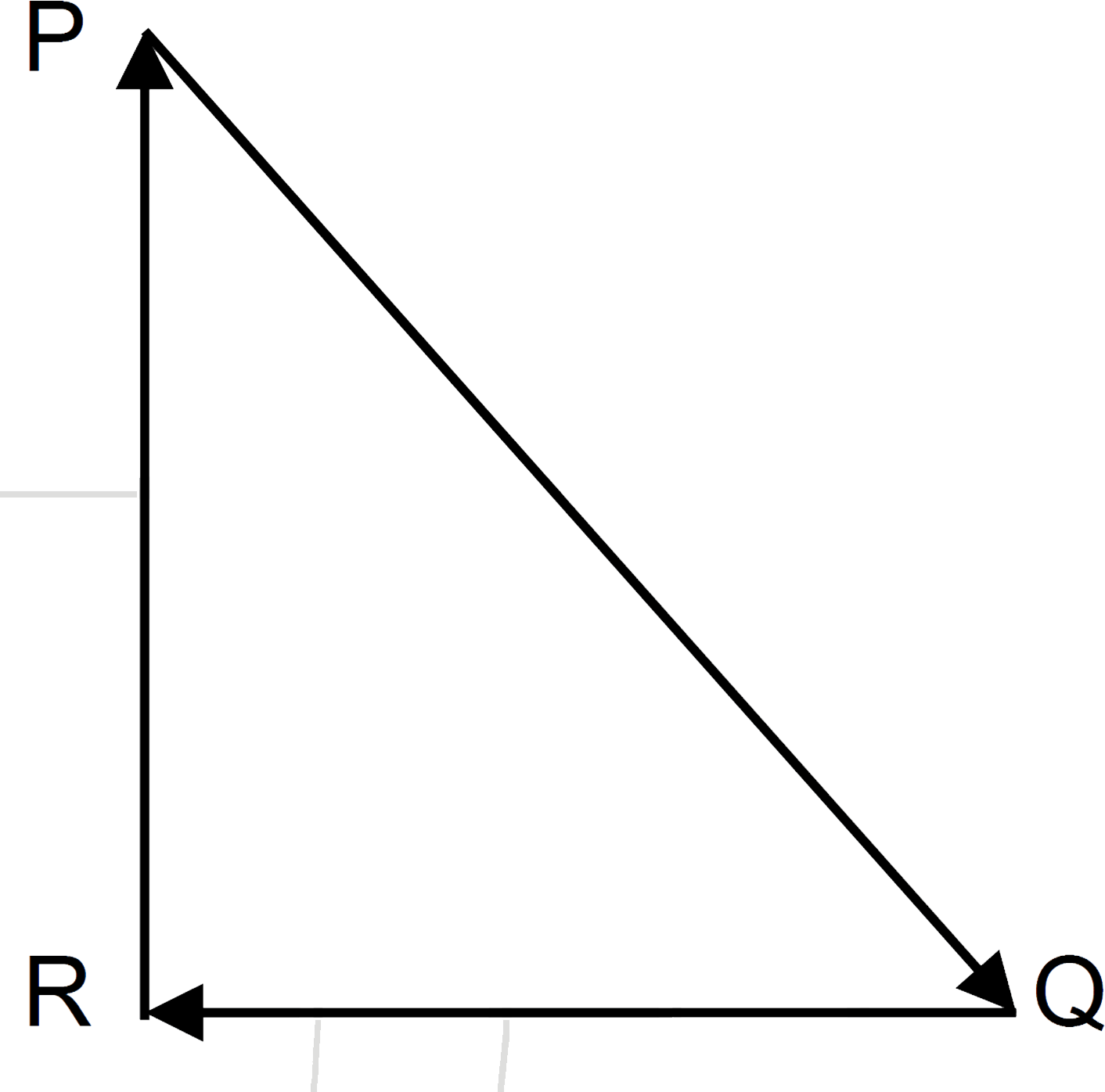 View Solution
View SolutionA particle moving with velocity →V is acted by the three forces shown by the vector triangle PQR. The velocity of the particle will :
 View Solution
View Solution

