Define and explain the term acceleration . Derive the velocity-time re

Which of the following represents the motion of a body moving in a straight line under constant acceleration ?
A
B
C
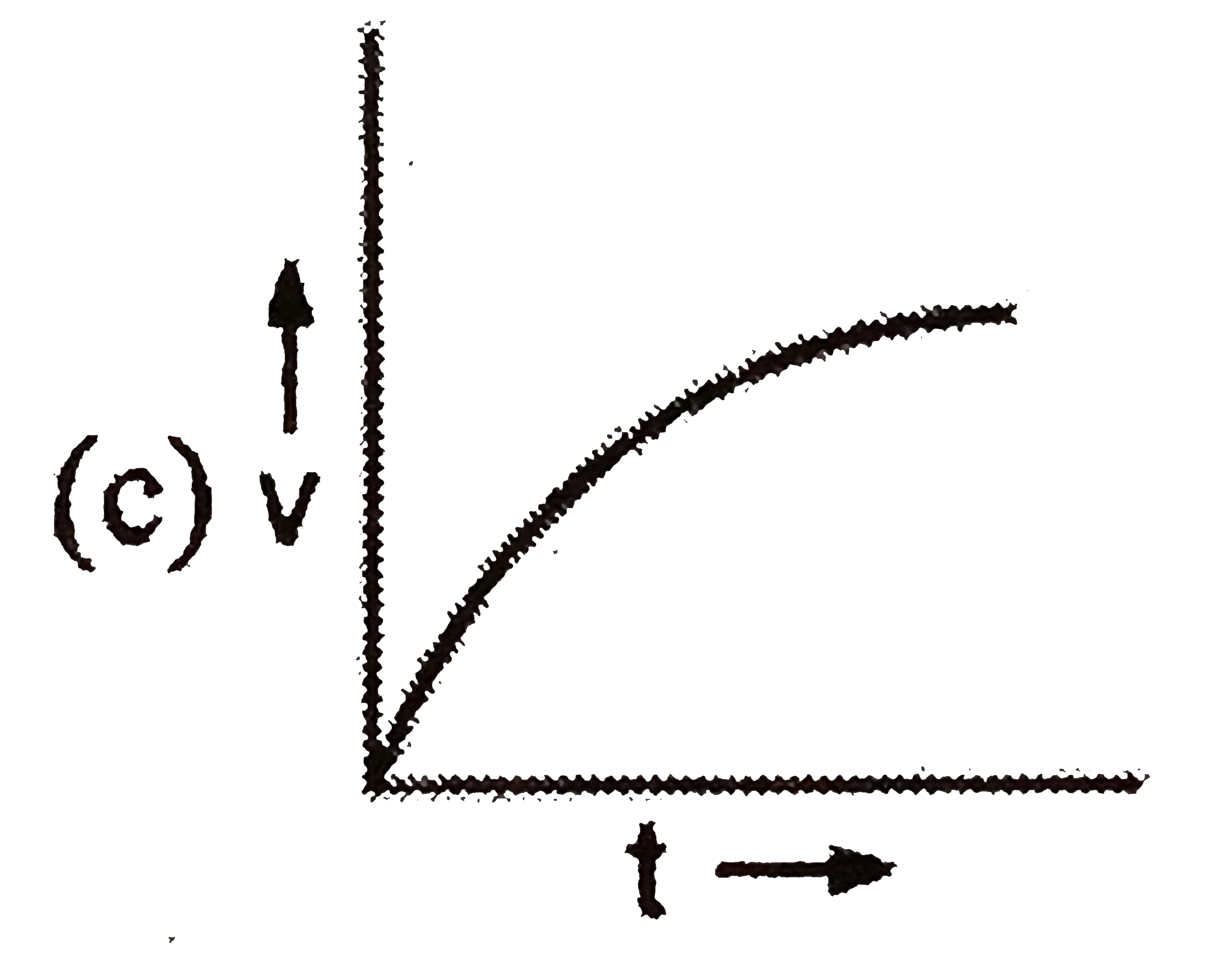D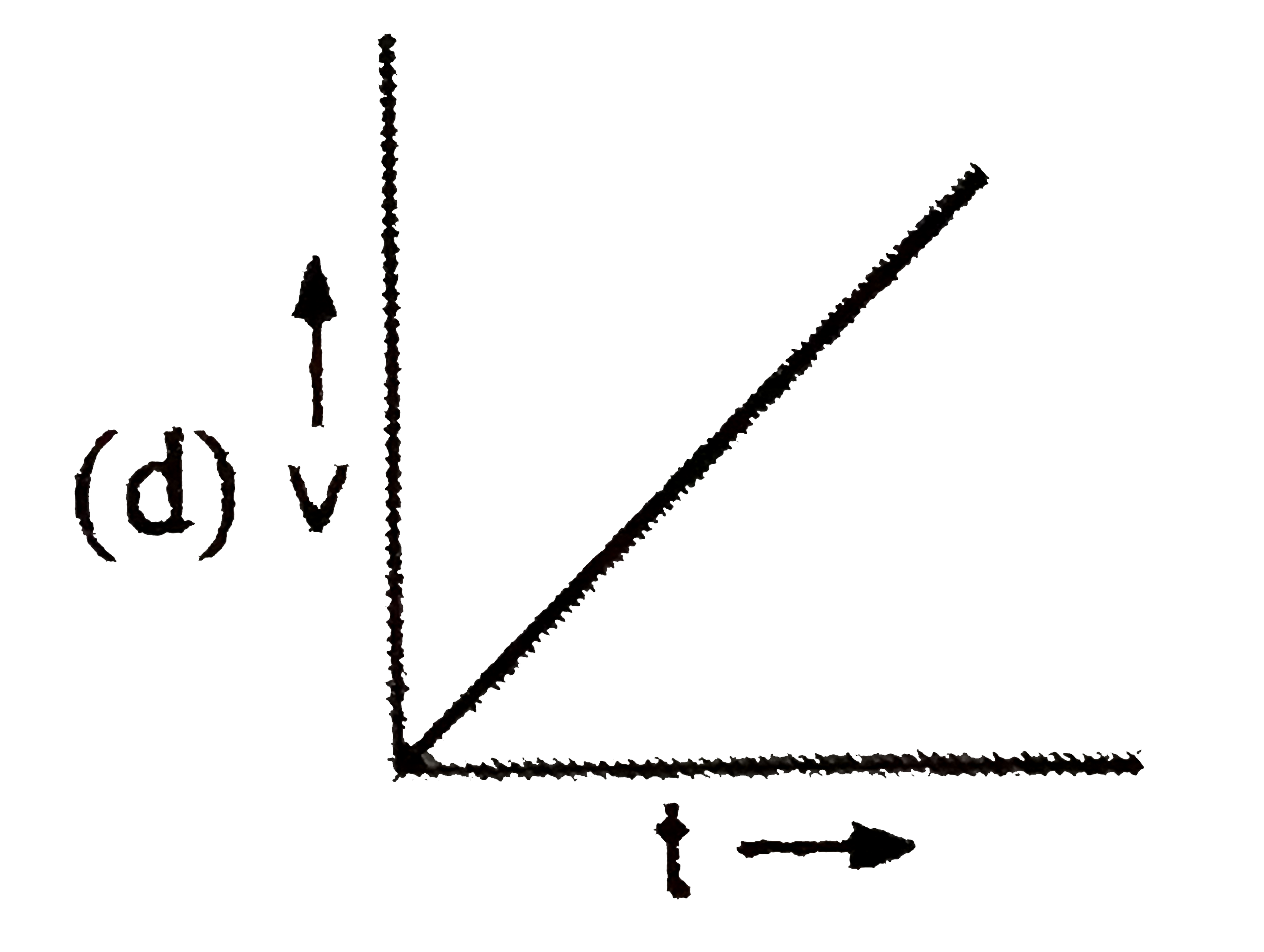
If v is the velocity of a body moving along x-axis, then acceleration of body is
A $\frac{d v}{d x}$
B $v \frac{d v}{d x}$
C $x \frac{d u}{d x}$
D $v \frac{d x}{d v}$
A : The area under acceleration - time graph is equal to velocity of object.
R : For an object moving with constant acceleration, position - time graph is a straight line.
AIf both Assertion & Reason are true and the reason is the correct explanation of the assertion, then mark (1).
BIf both Assertion & Reason are true but the reason is not the correct explanation of the assertion, then mark (2).
CIf Assertion is true statement but Reason is false, then mark (3).
DIf both Assertion and Reason are false statement, then mark (4).

Define and explain the term acceleration . Derive the velocity-time relation of a body moving under constant acceleration.