S.T. the time rate of the total angular momentum of a system of particles about a point is equal to the sum of the external torques acting on the system taken about the same point.
Step by step video & image solution for S.T. the time rate of the total angular momentum of a system of particles about a point is equal to the sum of the external torques acting on the system taken about the same point. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- System of particles & rotational MotionBOOK - SL ARORACHAPTER - System of particles & rotational MotionEXERCISE - EXERCISE
353 Videos
- ROTATIONAL MOTIONBOOK - SL ARORACHAPTER - ROTATIONAL MOTIONEXERCISE - Problem for self practice
90 Videos
- Thermal Properties of MatterBOOK - SL ARORACHAPTER - Thermal Properties of MatterEXERCISE - Exercise
449 Videos
Similar Questions
Knowledge Check
The rate of change of angular momentum of a system of particles about the centre of mass is equal to the sum of external torque about the centre of mass when the centre of mass is :
AFixed with respect to an inertial frame.Bin linear acceleration.Cin rotational motion.Dis in a translational motion.Consider the following statements
I. The rate of change of the total linear momentum of a system consisting of many particles is proportional to the vector sum of all the internal forces due to inter-particle interaction.
The internal forces can change the kinetic energy of the system of particles are not the linear momentum of the system. Choose the correct option.AOnly IBOnly IICI and IIDNone of these
Total linear momentum of a system of particles is equal to ……………..of the system and velocity of ……… .
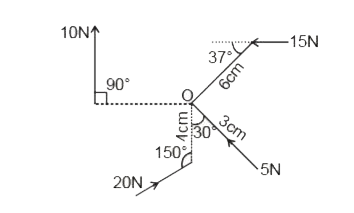
View SolutionCalculate the total torque acting on the body shown in figure about the point O.

View SolutionCalculate the total torque acting on the body shown in figure about the point O
View SolutionShow that the total linear momentum of a system of particles is conserved in the absence of any external force. Also show that total linear momentum of the system is equal to the product of the total mass of the system and the velocity of its centre of mass.
View SolutionAngular momentum of a particle about the origin O is equal to the vector product of its ______ vector and _______vector.
View SolutionProve that the rate of change of angular momentum is equal to torque acting on the particle
View Solution