Derive the equation showing the relation between the concentration and at time and
The intergrated rate equation for first order reaction is as,
In [R]=-k ….V(A)
So,time = and that time concentration then equation is
In and the equation is ……VII(A)
In .......VII(B)
Substracting VII (A) from VII (B),
In
.......VIII(A)
Taking log in this equation ,
2.303 log
....VIII(B)
From the above equation the following equation for k
.......IX(A)
and log .......IX (B)
In these equations .If =zero then and after t time , =t then so,in this situation above both equations are as under:
In .......XA
and log or .......XB

(The equation is like a form comparing with y=mx +c.The plot of log against t is straight line with egative slope.)
Topper's Solved these Questions
- CHEMICAL KINETICSBOOK - KUMAR PRAKASHANCHAPTER - CHEMICAL KINETICSEXERCISE - Section-A - Problem
1 Videos
- CHEMICAL KINETICSBOOK - KUMAR PRAKASHANCHAPTER - CHEMICAL KINETICSEXERCISE - Section-A - TRY YOURSELF
26 Videos
- BOARD EXAM QUESTION PAPER MARCH - 2020 BOOK - KUMAR PRAKASHANCHAPTER - BOARD EXAM QUESTION PAPER MARCH - 2020 EXERCISE - PART -B
23 Videos
- CHEMISTRY IN EVERYDAY LIFEBOOK - KUMAR PRAKASHANCHAPTER - CHEMISTRY IN EVERYDAY LIFEEXERCISE - SECTION - E (MCQs ASKED IN GUJCET/BOARD EXAM)
37 Videos
Similar Questions
Derive the equation, α=i−1n−1
Derive a relation between degree of dissociation and van't Hoff factor.
View SolutionFig, shows a system of two concentric spheres of radii r1 andr2 and kept at temperature T1 and T2(T1>T2) respectively. Find the expression for radial rate of flow of heat through the substance.
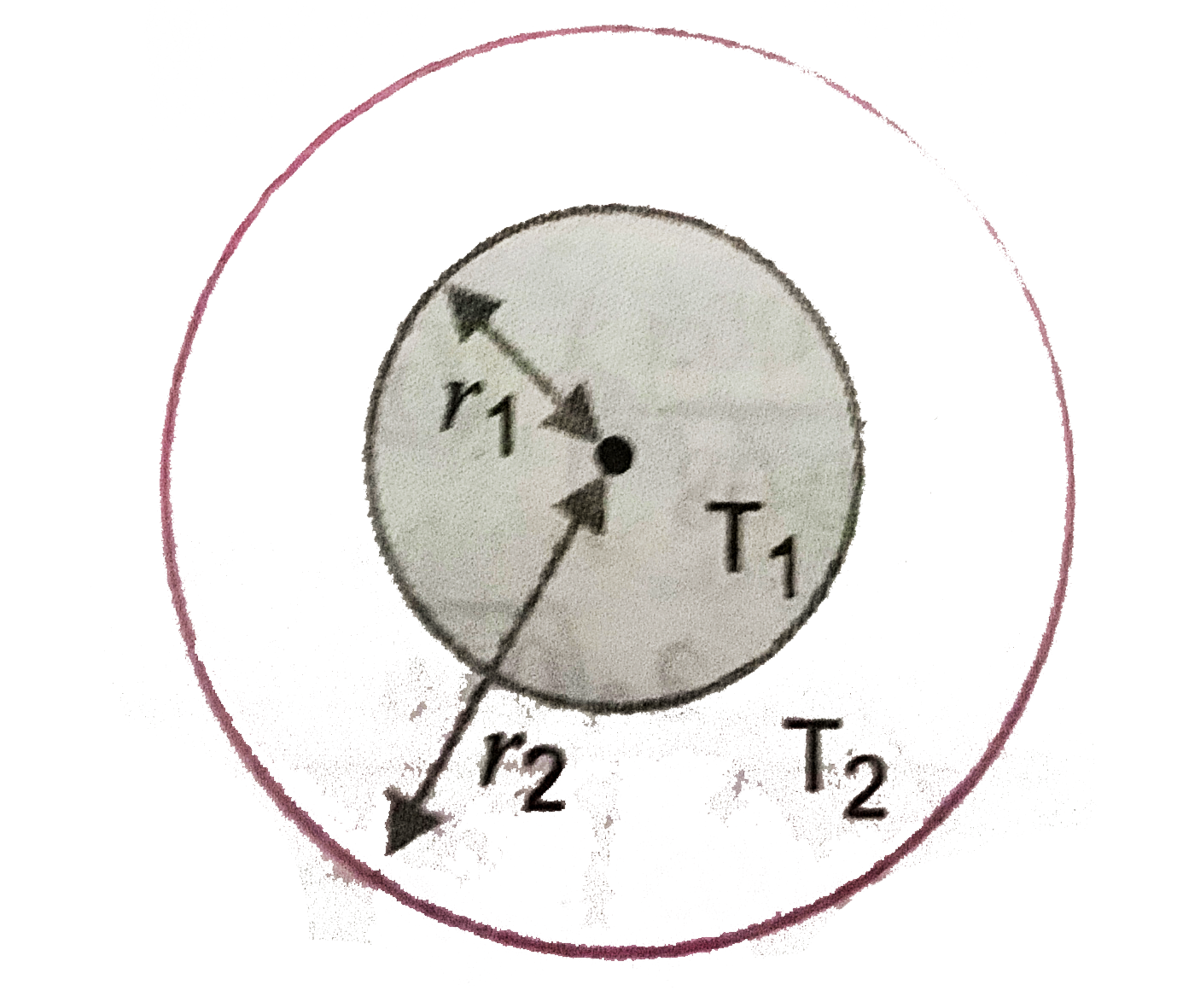
View Solution
Knowledge Check
The order of reaction A to product can be given by the expression (s)
[where r=rate of reaction, [A]1= concentration at tiem t1,[A]2= concentration at time t2Alnr2−lnr1ln[A]1Bln[A0]2−ln[A0]1ln[t1/2]2−ln[t1/2]1Cln(−dAk'dt)ln[A]Dln(rk)ln[A]The half-life of a radioactive sample is T. If the activities of the sample at time t1 and t2 (t1<t2) and R1 and R2 respectively, then the number of atoms disintergrated in time t2−t1 is proportional to
A(R1−R2)TB(R1+R2)TCR1R2R1+R2TDR1R2TActivity of a radioactive substance is R1 at time t1 and R2 at time t2(t2>t1) then the ratio R2R1 is :
At2t1Be−λ(t1+t2)Ce(t1−t2λ)Deλ(t1−t2)
The half-life of a radioactive sample is T. If the activities of the sample at time t1 and t2 (t1<t2) and R1 and R2 respectively, then the number of atoms disintergrated in time t2−t1 is proportional to
View SolutionThe activity of a radioactive substance is R1 at time t1 and R2 at time t2(>t1). Its decay cosntant is λ. Then .
View SolutionThe activity of a radioactive susbtance is R1 at time t1 and R2 at time t2(>t1). its decay constant is λ. Then, number of atoms decayed between time interval t1 and t2 are
View SolutionThe activity of a radioactive substance is R1 at time t1 and R2 at time t2(>t1). Its decay cosntant is λ. Then .
View SolutionThe rate of decay of a radioactive species is given by R1 at time t1 and R2 at later time t2. The mean life of this radioactive species is:
View Solution
