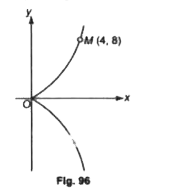
Compute the length of the arc of the semicubical parabola $y^{2} = x^{3}$ between the points (0, 0) and (4, 8)

Video Solution

Text Solution

Verified by Experts

The correct Answer is: $\frac{8}{27} (10 \sqrt{10} - 1)$

|

Answer

Step by step video, text & image solution for Compute the length of the arc of the semicubical parabola y^(2)=x^(3) between the points (0, 0) and (4, 8) by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.

Updated on:21/07/2023

Class 12 MATHS APPLICATION OF INTEGRALS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
The length of the latus rectum of the parabola $x^{2} - 4 x - 8 y + 12 = 0$ is
A4
B6
C8
D10
Question 2 - Select One
The focus of the parabola $y^{2} - 4 y - 8 x + 4 = 0$ is,
A(1, 1)
B(1, 2)
C(2, 1)
D(2, 2)
Question 3 - Select One
The mid-point of the chord $2 x + y - 4 = 0$ of the parabola $y^{2} = 4 x$ is
A(5/2, -1)
B(-1, 5/2)
C(3/2, -1)
Dnone of these

IA MARON-APPLICATIONS OF THE DEFINITE INTEGRAL-The Arc Length of a Plane Curve in Rectangular Coordinates

Home
Ask Doubt
Profile