Question

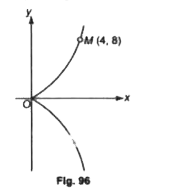
Compute the length of the arc cut off from the curve $y^2=x^3$ by the straight line $x=\frac{4}{3}$

Explanation

To compute the length of the arc cut off from the curve $$y^2=x^3$$ by the straight line $$x=\frac{4}{3}$$, we will follow these steps:

Step 1: Understand the curve and the line
The given curve is \( y^2 = x^3 \). This implies that \( y = \pm \sqrt{x^3} \). The line \( x = \frac{4}{3} \) will intersect the curve at points where \( x = \frac{4}{3} \).

Step 2: Find the points of intersection
To find the points of intersection, substitute \( x = \frac{4}{3} \) into the curve equation:
\( y^2 = \left(\frac{4}{3}\right)^3 = \frac{64}{27} \)
Thus,
\( y = \pm \sqrt{\frac{64}{27}} = \pm \frac{8}{3\sqrt{3}} = \pm \frac{8\sqrt{3}}{9} \)
The points of intersection are \( \left(\frac{4}{3}, \frac{8\sqrt{3}}{9}\right) \) and \( \left(\frac{4}{3}, -\frac{8\sqrt{3}}{9}\right) \).

Step 3: Set up the arc length formula
The formula for the length of the arc from \( x = a \) to \( x = b \) is given by:
\( L = \int_a^b \sqrt{1 + \left(\frac{dy}{dx}\right)^2} \, dx \)
Since the curve is symmetric about the x-axis, we can calculate the arc length for \( y = \sqrt{x^3} \) and then double it.

Step 4: Calculate \( \frac{dy}{dx} \)
From the equation \( y^2 = x^3 \), we differentiate implicitly:
\( 2y \frac{dy}{dx} = 3x^2 \implies \frac{dy}{dx} = \frac{3x^2}{2y} \)
Substituting \( y = \sqrt{x^3} \):
\( \frac{dy}{dx} = \frac{3x^2}{2\sqrt{x^3}} = \frac{3x^2}{2x^{3/2}} = \frac{3}{2} x^{1/2} \)

Step 5: Substitute \( \frac{dy}{dx} \) into the arc length formula
Now substituting into the arc length formula:
\( L = 2 \int_0^{\frac{4}{3}} \sqrt{1 + \left(\frac{3}{2} x^{1/2}\right)^2} \, dx \)
This simplifies to:
\( L = 2 \int_0^{\frac{4}{3}} \sqrt{1 + \frac{9}{4} x} \, dx \)

Step 6: Evaluate the integral
To evaluate the integral, we can use a substitution. Let:
\( u = 1 + \frac{9}{4} x \implies du = \frac{9}{4} dx \implies dx = \frac{4}{9} du \)
When \( x = 0 \), \( u = 1 \) and when \( x = \frac{4}{3} \), \( u = 1 + 3 = 4 \).

Thus, the integral becomes:
\( L = 2 \cdot \frac{4}{9} \int_1^4 \sqrt{u} \, du = \frac{8}{9} \cdot \left( \frac{2}{3} u^{3/2} \right)_1^4 \)
Calculating this gives:
\( = \frac{8}{9} \cdot \frac{2}{3} \left(4^{3/2} - 1^{3/2}\right) = \frac{16}{27} \left(8 - 1\right) = \frac{16}{27} \cdot 7 = \frac{112}{27} \)

Final Result
Thus, the length of the arc cut off from the curve by the line \( x = \frac{4}{3} \) is:
\( \boxed{\frac{112}{27}} \)