Four light sources produce the following waves
(I) $y_{1} = a \sin (ω t + ϕ_{1})$
(II) $y_{2} = a \sin (2 ω t)$
(III) $y_{3} = a^{1} \sin (ω t + ϕ_{2})$
(IV) $y_{4} = a^{2} \sin (3 ω t + ϕ)$
Superposition of which two waves gives rise to interferece

A

I and II

B

II and III

C

I and III

D

III and IV

Text Solution

Verified by Experts

The correct Answer is:C

Updated on:21/07/2023

|

Class 12 PHYSICS WAVE OPTICS

Topper's Solved these Questions

WAVES OPTICSBOOK - AAKASH SERIESCHAPTER - WAVES OPTICSEXERCISE - EXERCISE -II (DIFFRACTION)
17 Videos
WAVES OPTICSBOOK - AAKASH SERIESCHAPTER - WAVES OPTICSEXERCISE - EXERCISE -II (POLARISATION)
11 Videos
WAVES OPTICSBOOK - AAKASH SERIESCHAPTER - WAVES OPTICSEXERCISE - EXERCISE -IB (ASSERTION AND REASON)
68 Videos
WAVESBOOK - AAKASH SERIESCHAPTER - WAVESEXERCISE - EXERCISE-III (Doppler effect :)
15 Videos

Similar Questions

Knowledge Check

Four sound sources produce the following four waves
(i) $y_{1} = a sin (ω t + ϕ_{1})$
(ii) $y_{2} = a sin 2 ω t$
(iii) $y_{3} = a' sin (ω t + ϕ_{2})$
(iv) $y_{4} = a' sin (3 ω t + ϕ)$
Superposition of which two waves gives rise to interference?
A(i) and (ii)
B(ii) and (iii)
C(i) and (iii)
D(iii) and (iv)
Four sound sources produce the following four waves
(i) $y_{1} = a sin (ω t + ϕ_{1})$
(ii) $y_{2} = a sin 2 ω t$
(iii) $y_{3} = a' sin (ω t + ϕ_{2})$
(iv) $y_{4} = a' sin (3 ω t + ϕ)$
Superposition of which two waves gives rise to interference?
A(i) and (ii)
B(ii) and (iii)
C(i) and (iii)
D(iii) and (iv)
If $i_{1} = i_{0} sin (ω t), i_{2} = i_{0_{2}} sin (ω t + ϕ)$ , then $i_{3} =$

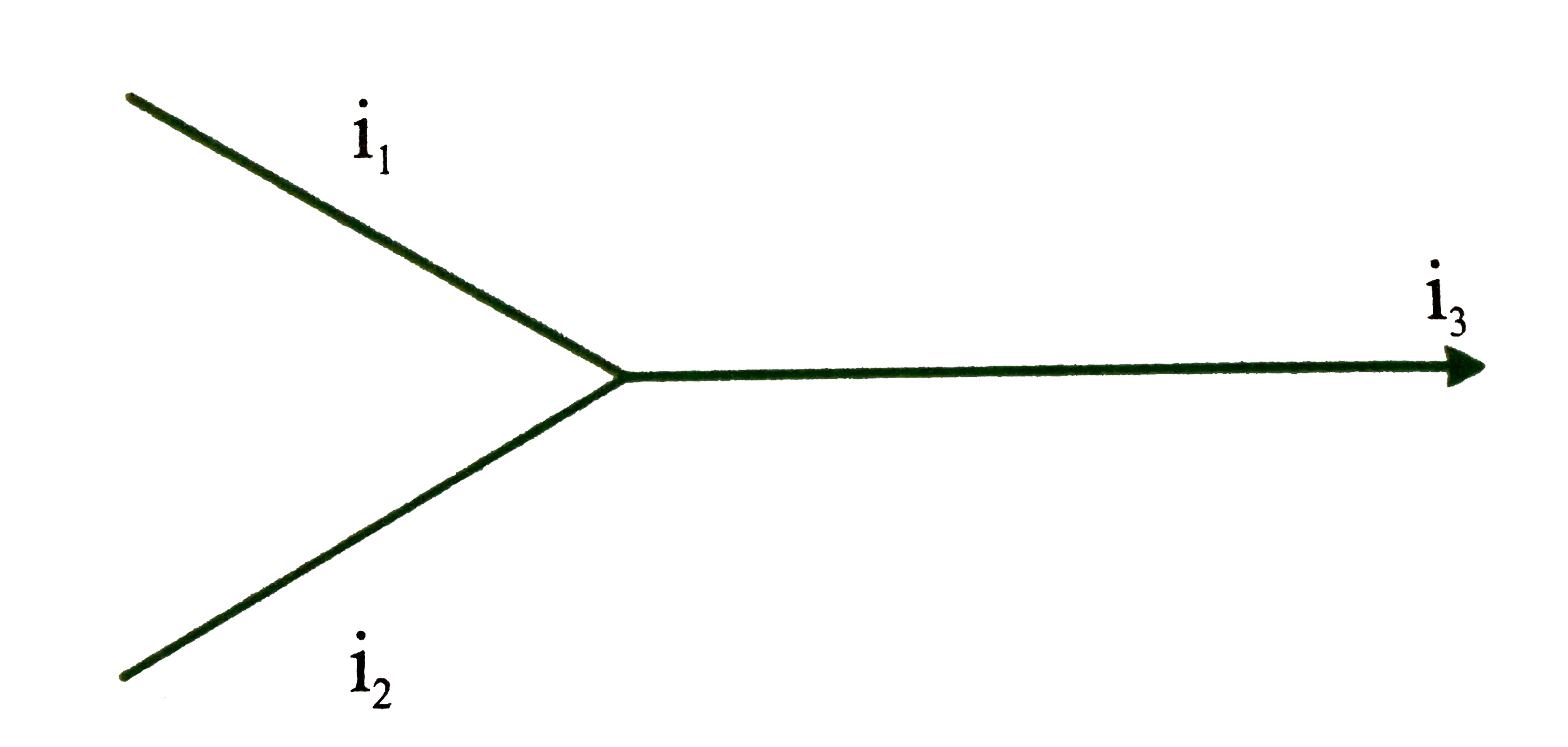A $\sqrt{i_{0_{1}}^{2} + i_{0_{2}}^{2}} sin {ϕ + ω t}$
B $(i_{0_{1}} + i_{0_{2}}) sin (\frac{ϕ}{2} + ω t)$
C $(\sqrt{i_{0_{1}}^{2} + i_{0_{2}}^{2} + 2 i_{0_{1}} i_{0_{2}} cos ϕ}) sin [ϕ + ω t]$
D $(\sqrt{i_{0_{1}}^{2} + i_{0_{2}}^{2} + 2 i_{0_{1}} i_{0_{2}} cos ϕ}) sin [α + ω t]$ where $α = {Tan}^{- 1} [\frac{i_{0_{2}} sin ϕ}{i_{0_{1}} + i_{0_{2}} cos ϕ}]$

AAKASH SERIES-WAVES OPTICS-EXERCISE -II (INTERFERENCE)

Home
Ask Doubt
Profile