A point charge q is placed at a distance d from the centre of a circular disc of radius R. Find electric flux flowing through the disc due to that charge
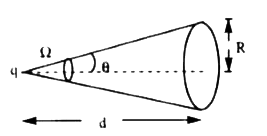
We know that total flux originated from a point charge q in all directions is q∈0. This flux is originated in a solid angle 4π. In the given case solid angle subtended by the cone subtended by the disc at the point charge is Ω=2π(1−cosθ)
So, the flux of q which is passing through the surface of the disc is
ϕ=q∈0Ω4π=q2∈0(1−cosθ)
From the figure, cosθ=d√d2+R2,
so ϕ=q2∈0{1−d√d2+R2}
Topper's Solved these Questions
- ELECTRIC CHARGES AND FIELDSBOOK - AAKASH SERIESCHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - Assess Your Self
13 Videos
- ELECTRIC CHARGES AND FIELDSBOOK - AAKASH SERIESCHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - Exercise-I
80 Videos
- DUAL NATURE OF RADIATION AND MATTERBOOK - AAKASH SERIESCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - PRACTICE EXERCISEX
44 Videos
- ELECTRIC FIELD AND POTENTIALBOOK - AAKASH SERIESCHAPTER - ELECTRIC FIELD AND POTENTIALEXERCISE - PROBLEMS (LEVEL-II)
32 Videos
Similar Questions
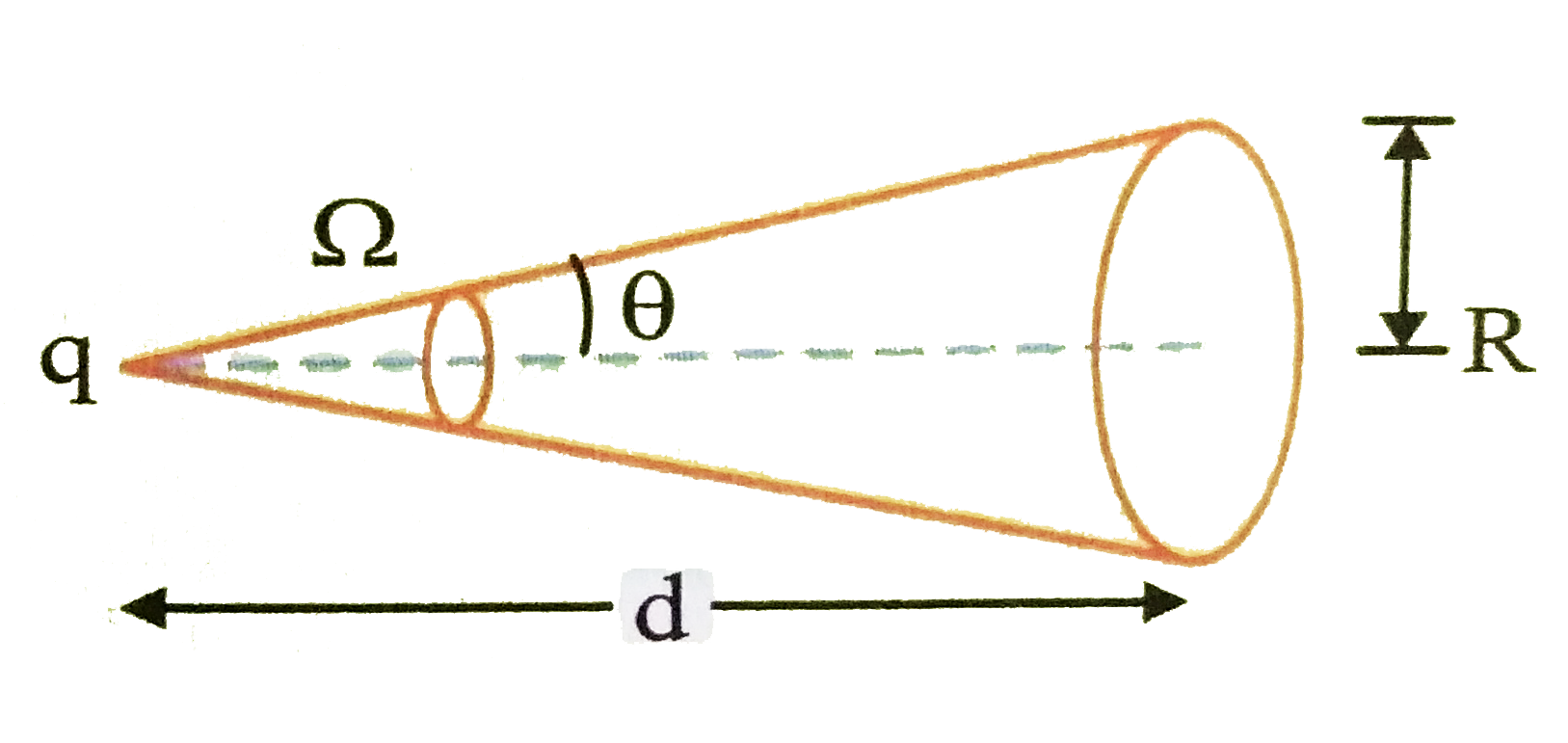
A point charge q is placed at a distance d form the center of circular disc of radius R. Find electric flux through the disc due to that charge
View SolutionA point charge q is placed at a distance a/2 directly above the centre of a square of side a. The electric flux through the square is
View Solution
Knowledge Check
A point charge q is placed at a distance a/2 directly above the center of a square of side a. The electric flux through the square is
Aqε0Bqπε0Cq4ε0Dq6ε0A position charge Q is placed at a distance of 4R above the centre of a disc of radius R. The magnitude of flux through the disc is ϕ. Now a hemispherical shell of radius R is placed over the disc such that it forms a closed surface. The flux through the curved surface (taking direction of area vector along outward normal as positive), is -
 AzeroBϕC−ϕD2ϕ
AzeroBϕC−ϕD2ϕa point charge q is placed at the centre of a cylinder of length L and radius R the electric flux through the curved surface of the cylinder is
Aqε0L√L2+R2Bqε0L√L2+2R2Cqε0L√L2+4R2Dq2ε0
A point charge Q is placed at the centre of circular disc of radius r . Calculate the electric flux associated with circular disc.
View SolutionA charge q is placed at a distance of 2R from the centre of a spherical conducting shell of radius R. What is electrical potential, due to charges induced on the shell, at a point which is farthest from the point charge ?
View SolutionA charge q is placed at a distance of 2R from the centre of a spherical conducting shell of radius R. What is electrical potential, due to charges induced on the shell, at a point which is farthest from the point charge ?
View SolutionA position charge Q is placed at a distance of 4R above the centre of a disc of radius R. The magnitude of flux through the disc is ϕ. Now a hemispherical shell of radius R is placed over the disc such that it forms a closed surface. The flux through the curved surface (taking direction of area vector along outward normal as positive), is -

View SolutionA charge q is placed at the centre of a hollow sphere of radius r, Total electric flux passing through the spherical surface is __________
View Solution
