The co - ordinates of centre of mass of particle of mass 10, 20 and 30 gm are (1, 1, 1) cm. The position co - ordinates of mass 40 gm which when added to the system, the position of combined centre of mass be at (0, 0, 0) are, Solution in Telugu
(3/2, 3/2, 3/2)
(−3/2,−3/2,−3/2)
(3/4, 3/4, 3/4)
(−3/4,−3/4,−3/4)
The correct Answer is:B
Step by step video, text & image solution for The co - ordinates of centre of mass of particle of mass 10, 20 and 30 gm are (1, 1, 1) cm. The position co - ordinates of mass 40 gm which when added to the system, the position of combined centre of mass be at (0, 0, 0) are, by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Topper's Solved these Questions
- BOOK - AAKASH SERIESCHAPTER - CENTRE OF MASSEXERCISE - LECTURE SHEET (EXERCISE - II)4videos
CENTRE OF MASS
- BOOK - AAKASH SERIESCHAPTER - CENTRE OF MASSEXERCISE - LECTURE SHEET (EXERCISE - III)7videos
CENTRE OF MASS
- BOOK - AAKASH SERIESCHAPTER - CALORIMETRY EXERCISE - ADDITIONAL PRACTICE EXERCISE (LEVEL - II) (LECTURE SHEET (ADVANCED)(Matrix Type Questions)1videos
CALORIMETRY
- BOOK - AAKASH SERIESCHAPTER - COLLISIONS EXERCISE - QUESTIONS FOR DESCRIPTIVE ANSWERS4videos
COLLISIONS
Similar Questions
The centre of mass of a non uniform rod of length L whose mass per unit length λ=Kx2L, where L is a constant and x is the distance from one end is
03:53View SolutionA uniform disc of radius R is put over another uniform disc of radius 2R of same thickness and density. The peripheres of the two discs touch eachother. The position of their centre of mass is
03:32View SolutionThree liquids A,B and C having same specific heat and mass m , 2m and 3m have temperature 20∘C,40∘Cand60∘C respectively. Temperature of the mixture when :
Column -IColumn -IIA) A and B are mixed,is atP)35∘CB) A and C are mixed , is at Q)52∘CC) B and C are mixed , is at Q)50∘CD) A , B and C all three are mixed , is at S)45∘CT) None02:48View SolutionFour identical balls A,B,C and D are placed in a line on a frictionless horizontal surface. A and D are moved with same speed .u. towards the middle as shown. Assuming elastic collisions, find the final velocities.
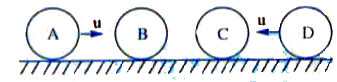 View Solution
View SolutionCentre of mass of 3 particles 10 kg, 20 kg and 30 kg is at (0, 0, 0). Where should a particle of mass 40 kg be placed so that the combination centre of mass will be at (3,3,3)
03:57View SolutionThe position co-ordinates of the centre of mass of a system of three particles of equal masses which are placed on the verctices of an equilateral triangle of a side a are shown. Locate centre mass?
05:30View SolutionThe sum of moments of masses of all the particles in a system about the center of mass is
05:17View Solution(A) : The centre of mass of system of n particles is the weight average of the position vector of the n particles making up the system. (R ) : The position of the centre of mass of a system is independent of co-ordinate system.
03:57View SolutionCentre of mass of two particles with masses 2 kg and 1 kg located at (1, 0, 1) and (2, 2, 0) has the co-ordinates of
View SolutionThe co - ordinates of centre of mass of particle of mass 10, 20 and 30 gm are (1, 1, 1) cm. The position co - ordinates of mass 40 gm which when added to the system, the position of combined centre of mass be at (0, 0, 0) are,
View SolutionThe co-ordinates of the centre of mass of the system as shown in figure are
03:56View SolutionCentre of mass of two particle with masses 2 kg and 1 kg locsted at (1, 0, 1) and (2, 2, 0) has the co - ordinates of
05:30View SolutionThe co-ordinates of centre of mass of particles of mass 10, 20 and 30 gm are (1, 1, 1) cm. The position co-ordinates of mass 40 gm which when added to the system, the position of combined centre of mass be at (0, 0, 0) are,
05:26View SolutionCentre of mass of 3 particles 10 kg, 20 kg and 30 kg is at (0, 0, 0). Where should a particle of mass 40 kg be placed so that the combined centre of mass will be at (3, 3, 3)?
02:50View SolutionThe coordinates of the positions of particles of mass 7, 4 and 10 gm are (1, 5,-3) , (2, 5, 7) and (3, 3,-1) respectively. Find the position of the center of mass of the system?
03:53View Solution

