A solid sphere of radius ‘r’ is kept on a concave mirror of radius of curvature R. The arrangement is kept on a horizontal table. If the sphere is displaced from its equilibrium position and left, then it executes SHM. The period of oscillation will be (sphere does't slip)
2π√[(R−r)(1.4)g]
2π ⎷[(R−r)g]
2π ⎷[(Rr)g]
2π ⎷[(R)gr]
The correct Answer is:A
Topper's Solved these Questions
- SIMPLE HARMONIC MOTION Book:AAKASH SERIESChapter:SIMPLE HARMONIC MOTION Exercise:PRACTICE SHEET (EXERCISE - I)(LEVEL - II)(STRAIGHT OBJECTIVE TYPE QUESTIONS)
Explore 12 Videos
- SIMPLE HARMONIC MOTION Book:AAKASH SERIESChapter:SIMPLE HARMONIC MOTION Exercise:PRACTICE SHEET (EXERCISE - I)(LEVEL - II)(MORE THAN ONE CORRECT ANSWER TYPE QUESTIONS)
Explore 2 Videos
- SIMPLE HARMONIC MOTION Book:AAKASH SERIESChapter:SIMPLE HARMONIC MOTION Exercise:LECTURE SHEET (EXERCISE - V)(INTEGER TYPE QUESTIONS)
Explore 2 Videos
- ROTATIONAL MECHANICSBook:AAKASH SERIESChapter:ROTATIONAL MECHANICSExercise:Questions for Descriptive Answers
Explore 20 Videos
- SURFACE TENSIONBook:AAKASH SERIESChapter:SURFACE TENSIONExercise:ADDITIONAL PRACTICE EXERCISE (PRACTICE SHEET (ADVANCED) INTEGER TYPE QUESTIONS )
Explore 8 Videos
Similar Questions
A sphere of radius r is kept on a concave mirror of radius of curation R. The arrangement is kept on a horizontal surface (the surface of concave mirror is friction less and sliding not rolling). If the sphere is displaced from its equilibrium position and left, then it executes S.h.M. The period of oscillation will be
View SolutionA small lead sphere is made to execute shm inside a concave dish of radius of curvature 1 m. What is its period ?
View Solution
Knowledge Check
A sphere of catius r is kept on a concave of mirror radius of curation R. The arrangement a kept on a horizontal tavke (the surface of concave mirror is fricationless and sliding not rolling). If the sphere is displaced from its equilibrium position and left, then it executes S.h.M. The period of oscillation will be
A2π ⎷((R−r)1.44)B2π√(R−rg)C2π√(rRa)D2π√(Rgr)A solid sphere of radius R is rolled by a force F acting at the topo of the sphere as shown in the figure. There is no slipping and initially sphere is in the rest position, then (CM= centre of mass)
 AWork done by force F when the centre of mass move a distance S is 2 FSBspeed of the CM when CM moves a distance S is √207FSMCwork done by the Force F when CM move a distance S is FSDspeed of the CM when CM moves a distance S is √65FSM
AWork done by force F when the centre of mass move a distance S is 2 FSBspeed of the CM when CM moves a distance S is √207FSMCwork done by the Force F when CM move a distance S is FSDspeed of the CM when CM moves a distance S is √65FSMThe radius of gyration of a solid sphere of radius r about a certain axis is r. The distance of this axis from the centre of the sphere is
ArB0.5 rC√0.6rD√0.4r
A small sphere is placed on a concave mirror of radius of curvature 'R' a little away from the centre.When the sphere is released it oscillates.Assuming the oscillation to be simple harmonic its time period is
View SolutionA solid sphere of radius R is rolled by a force F acting at the topo of the sphere as shown in the figure. There is no slipping and initially sphere is in the rest position, then (CM= centre of mass)

View SolutionIn the arrangement shown in figure a solid sphere is attached to spring and displaced, then it starts S.H.M. without slipping, the time period of sphere is
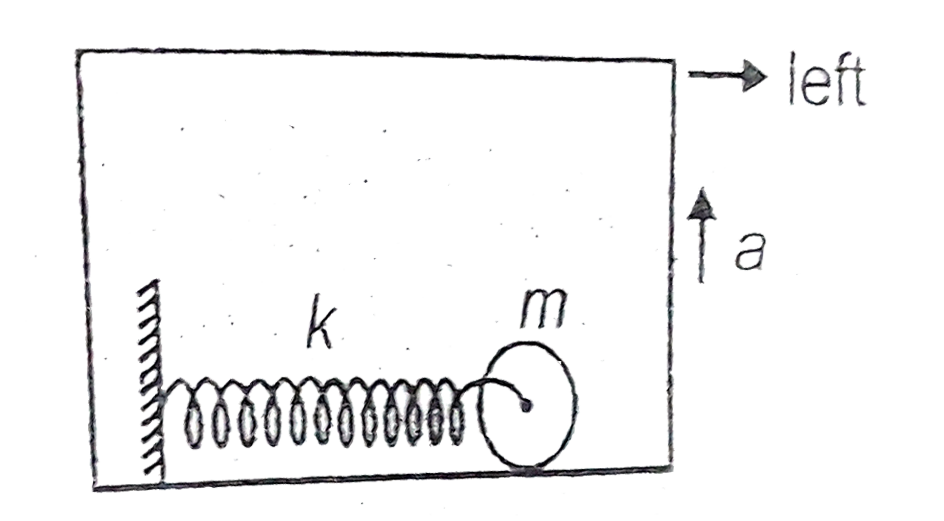
View SolutionA sphere of mass M and radius R is held on the inner wall of a hollow sphere of mass 4M and radius 6R .The bigger sphere is kept on a smooth horizontal table and the centres of the two spheres lie on horizontal line.The system of two spheres is released from this position. The smaller sphere slides on the inner smooth wall to reach the other extreme position (shown in dotted line).The displacement of the centre of the larger sphere as the smaller sphere moves from one extreme to another is xcm. Value of x is: ( Take R=4.5cm) qquad y qquad yR Delta M
View SolutionA non-conducting solid sphere of radius R is uniformly charged. The magnitude of the electric filed due to the sphere at a distance r from its centre
View Solution
