Doubtnut Promotions Sticky

A ball of mass M and a pan of mass m ( m < M ) are attached to the ends of a light inextensible string, passing over a smooth light pulley. The ball rests on a horizontal surface, with the pan hanging. A small particle of mass m collides elastically with the pan, falling from above, with a velocity v. Determine the instantaneous velocity of the ball, as it leaves contact with the surface. Find also the velocity with which the particle rebounds.
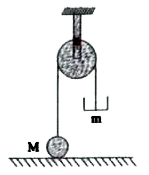
A ball of mass M and a pan of mass m (m lt M) are attached to the ends of a light inextensible string, passing over a smooth light pulley. The ball rests on a horizontal surface, with the pan hanging. A small particle of mass m collides elastically with the pan, falling from above, with a velocity v. Determine the instantaneous velocity of the ball, as it leaves contact with the surface. Find also the velocity with which the particle rebounds.

Text Solution
Verified by Experts

Let v 1 be the velocity of the pan (downwards) as well as the ball (upwards) and v 2 be the velocity (upward) of the particle just after the collision.
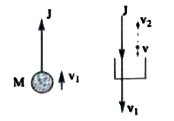
ocr_image
If J be the impulse transmitted through the string, then, conserving the momentum during the impact,
m v = m v 1 - m v 2 + J ... ( 1 ) and J = M v 1 ... ( 2 ) m v ( M + m ) v 1 - m v 2 ... ( 3 )
Since the collision is elastic, e =1
i.e., velocity of separation = velocity of approach.
⇒ v 1 + v 2 = v ... . ( 4 )
Solving (3) and (4), v 1 = 2 m v M + 2 m and v 2 = M v M + 2 m

Doubtnut Promotions Banner
|
Updated on:21/07/2023
Class 11PHYSICSCOLLISIONS

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • Question 1 - Select One or More

    Two masses M and m(M>m) are joined by a light string passing over a smooth light pulley.
    ocr_image

    AThe acceleration of each block is (M−mM+m)g
    BThe tension in the string is 2MmgM+m
    CThe centre of mass of the 'M plus m' system moves down with an acceleration of g(M−mM+m)2
    DThe tension in the string by which the pulley is attached to the rodd is (M+m)g
  • Question 2 - Select One or More

    Two masses m and M(m<M) are joined by a light string passing over a smooth and light pulley (as shown)
    ocr_image

    Athe acceleration of each mass is (M−mM+m)g
    Bthe tension in the string connecting masses is (2MmM+m)g
    Cthe thrust acting on the pulley is (4MmM+m)g
    DNone of the above
  • Question 3 - Select One

    Two masses m and M(>m) are joined by a light string passing over a smooth light pulley. The centre of mass of the system moves with an acceleration
    ocr_image

    Ag(M−mM+m) downward
    Bg(M−mM+m)2 downward
    Cg(M−mM+m)2 upward if M<m
    Dzero