Find the centre of mass of the letter F which is cut from a uniform metal sheet as shown. Take lower left corner as origin.
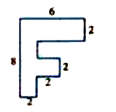
Text Solution
The correct Answer is:xc=15/7,y=33/7
|
Similar Questions
Knowledge Check
The centre of mass of the letter F which is cut from a uniform metal sheet from point A is
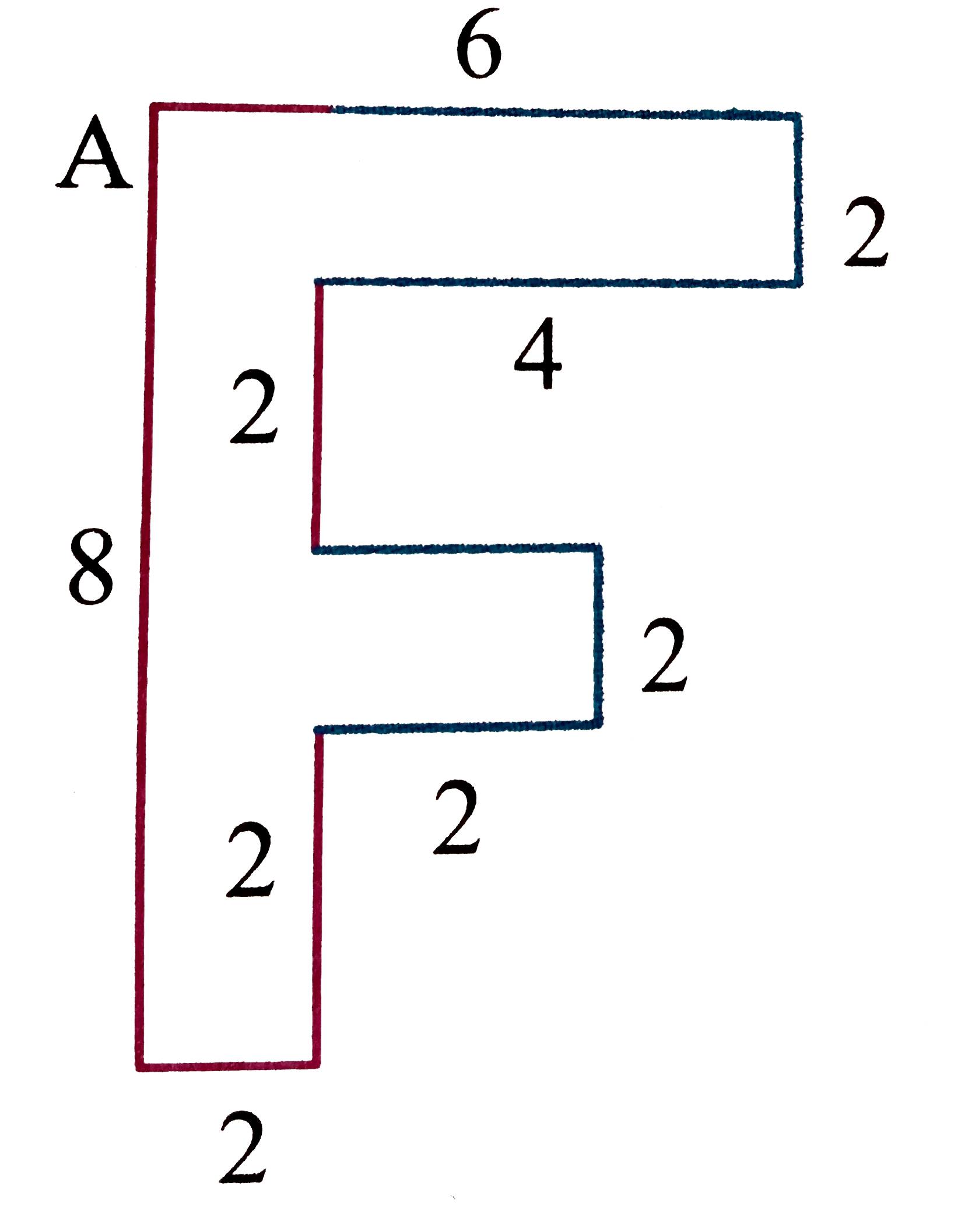 A15/7,33/7B15/7,23/7C22/7,33/7D33/7,22/7
A15/7,33/7B15/7,23/7C22/7,33/7D33/7,22/7The co-ordinates of centre of mass of letter E which is cut from a uniform metal sheet are (take origin at bottom left corner and width of letter 2cm every where)
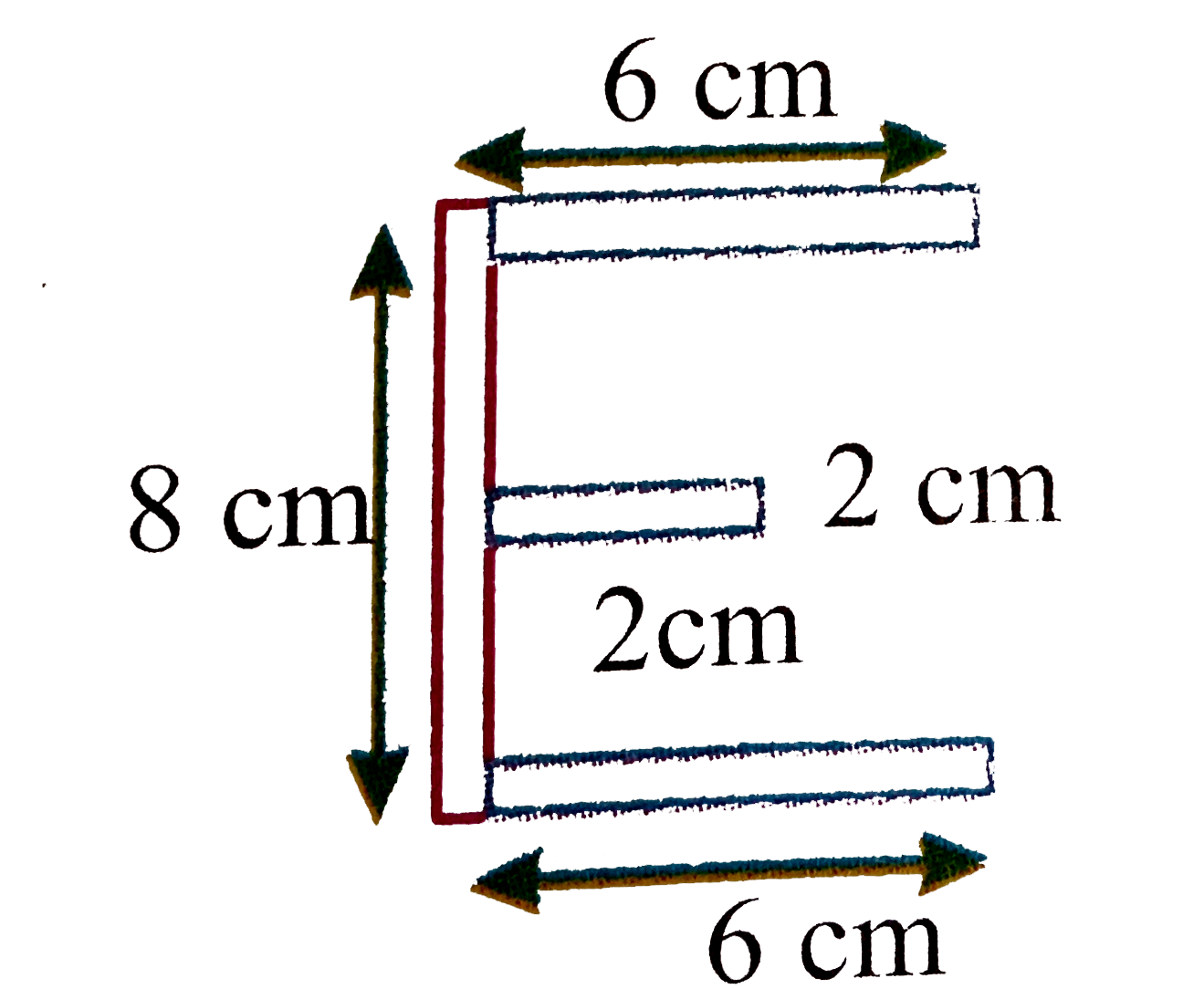 A(2cm,4cm)B(2.4cm,5cm)C(3cm,5cm)D(3.3cm,4cm)
A(2cm,4cm)B(2.4cm,5cm)C(3cm,5cm)D(3.3cm,4cm)Three identical spheres of mass M each are placed at the corners of an equilateral triangle of side 2 m. Taking one of the corners as the origin, the position vector of the centre of mass is
A√3(ˆi−ˆj)Bˆi√3+ˆjCˆi+ˆj/3Dˆi+ˆj/√3
Find the position of centre of mass of the uniform planner sheet shown in figure with respect to the origin (O) -
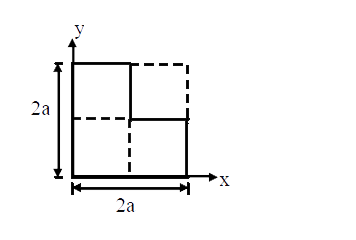
View SolutionFind out the position of centre of mass of the uniform lamina as shown in figure.
View SolutionThree identical spheres of mass M each are placed at the corners of an equilateral triangle of side 2 m. Taking one of the corners as the origin, the position vector of the centre of mass is
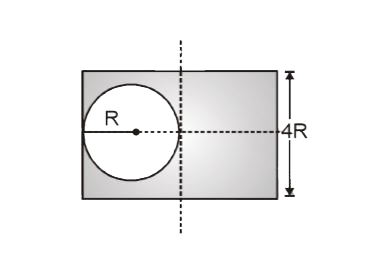
View SolutionFind the centre of mass of a thin, uniform disc of radius R from which a small concentric disc of radius r is cut.
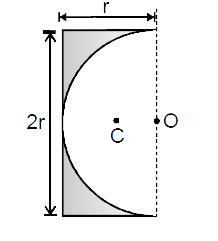
View SolutionA semicircular portion of radius 'r' is cut from a uniform rectangular plate as shown in figure. The distance of centre of mass 'C' of remaining plate, from point 'O' is
View Solution

