Three identical spheres of mass M each are placed at the corners of an equilateral triangle of side 2 m. Taking one of the corners as the origin, the position vector of the centre of mass is
√3(ˆi−ˆj)
√3(ˆi−ˆj)
ˆi√3+ˆj
ˆi+ˆj3
The correct Answer is:D
To find the position vector of the center of mass of three identical spheres placed at the corners of an equilateral triangle with a side length of 2 m, we can follow these steps:
Step 1: Define the Coordinates of the Masses
We place the triangle in the Cartesian coordinate system. Let’s assign the following coordinates to the three masses located at the corners of the triangle:
- Mass M1 at point A (0, 0)
- Mass M2 at point B (2, 0)
- Mass M3 at point C (1, √3) (This is derived from the height of the equilateral triangle, which is √32×2=√3)
Step 2: Use the Center of Mass Formula
The position vector of the center of mass →Rcm for a system of particles is given by:
→Rcm=M1→r1+M2→r2+M3→r3M1+M2+M3
Since all the masses are identical (mass M), we can simplify this to:
→Rcm=M(→r1+→r2+→r3)3M=→r1+→r2+→r33
Step 3: Substitute the Coordinates
Now we substitute the coordinates of the three masses into the formula:
→Rcm=(0,0)+(2,0)+(1,√3)3
Step 4: Calculate the Sum of the Coordinates
Now, we calculate the sum of the coordinates:
→Rcm=(0+2+1,0+0+√3)3=(3,√3)3=(1,√33)
Step 5: Write the Position Vector
Thus, the position vector of the center of mass is:
→Rcm=1^i+√33^j
Final Answer
The position vector of the center of mass is:
→Rcm=^i+√33^j
We place the triangle in the Cartesian coordinate system. Let’s assign the following coordinates to the three masses located at the corners of the triangle:
- Mass M1 at point A (0, 0)
- Mass M2 at point B (2, 0)
- Mass M3 at point C (1, √3) (This is derived from the height of the equilateral triangle, which is √32×2=√3)
The position vector of the center of mass →Rcm for a system of particles is given by:
Now we substitute the coordinates of the three masses into the formula:
Now, we calculate the sum of the coordinates:
Thus, the position vector of the center of mass is:
The position vector of the center of mass is:
Similar Questions
Three particle each of mass m are placed at the corners of equilateral triangle of side l

Which of the following is/are correct ?
View SolutionThree particle of mass m each are placed at the three corners of an equilateral triangle of side a. Find the work which should be done on this system to increase the sides of the triangle to 2a.
View Solution
Knowledge Check
Three point masses each of mass m are placed at the corners of an equilateral triangle of side b. The moment of inertia of the system about an axis coinciding with one side of the triangle is
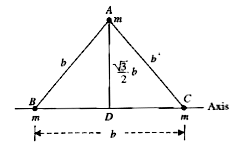 A3mb2Bmb2C(3/4)mb2D(2/3)mb2
A3mb2Bmb2C(3/4)mb2D(2/3)mb2
Three particle of mass m each are placed at the three corners of an equilateral triangle of side a. Find the work which should be done on this system to increase the sides of the triangle to 2a.
View SolutionThree particle of mass m each are placed at the three corners of an equilateral triangle of side a. Find the work which should be done on this system to increase the sides of the triangle to 2a.
View SolutionThree equal charge q0 each are placed at three corners of an equilateral triangle of side 'a'. Find out force acting on one of the charge due to other two charges ?
View Solution3 particles of mass 10 gm each are placed at the corners of an equilateral triangle of side 5 cm. The M.I. of the system about a perpendicular axis to the plane passing through a corner of the triangle will be : (in kg-m2)
View SolutionThree particle of mas m each are placed at the three corners of an equlateral triangle of side a. Find the work which should be done on this system to increase the sides of the triangle to 2a.
View SolutionThree masses 3, 4 and 5 kg are placed at the centre of an equiliteral triangle of 1 m sides. Locate its centre of mass.
View SolutionThree identical charges, each having a value 1.0×10−8C, are placed at the corners of an equilateral triangle of side 20 cm find the electric field and potential at the centre of the triangle.
View Solution
