Calculate the force .F. needed to punch a 1.46 cm diameter hole in a steel plate 1.27 cm thick (as shown in fig). The ultimate shear strength of steel is 345MN/m2

As in punching, shear elasticity is involved, the hole will be punched it
(F11A)> ultimate shears streass :F11> (shear stress) X Area
F11min=(3.45×108)(2πrL)(A=2πrL)
=(3.45×108)(2×3.14)×0.73×10−2×1.27×10−2≈200KN
Topper's Solved these Questions
- ELASTICITYBOOK - AAKASH SERIESCHAPTER - ELASTICITYEXERCISE - TEST YOUR SELF
11 Videos
- ELASTICITYBOOK - AAKASH SERIESCHAPTER - ELASTICITYEXERCISE - Very Short Answer Type Questions
15 Videos
- COLLISIONS BOOK - AAKASH SERIESCHAPTER - COLLISIONS EXERCISE - QUESTIONS FOR DESCRIPTIVE ANSWERS
4 Videos
- ELEMENTS OF VECTORS BOOK - AAKASH SERIESCHAPTER - ELEMENTS OF VECTORS EXERCISE - QUESTIONS FOR DESCRIPTIVE ANSWER
10 Videos
Similar Questions
Calculate the force F needed to punch at 1.46 cm diameter hole in a steel plate 1.27cm thick fig. The ultimates shear stress of steel is 3.45×108Nm−2.

View SolutionSteel ruptures when a shear of3.5×108Nm−2 is applied. The force needed to punch a 1cm diameter hole in a steel sheet 0.3cm thick is nearly:
View Solution
Knowledge Check
Calculate the force 'F' needed to punch a 1.46cm diameter hole in a steel plate 1.27cm thick. The ultimate shear strength of steel s 345MN/m2 (Approx).
A300KNB400KNC200KND100KNThe force required to punch a hole of diameter 2 mm, will be (If a shearing stress 4×108N/m2.)
A400πNB1600πNC1800πND1200πNIf a cylinder of diameter 1.0cm at 30∘C is to be slid into a hole of diameter 0.9997cm in a steel plate at the same temperature, the minimum required rise in the temperature of the plate is: (Coefficient of linear expansion of steel=12×10−6/∘C)
A25∘CB35∘CC45∘CD55∘C
Two wires of diameter 0.25 cm, one made of steel and the other made of brass are loaded as shown in fig. The unloaded length of steel wire is 1.5m and that of brass wire is 1.0m. Compute the elongations of the steel and the brass wires.
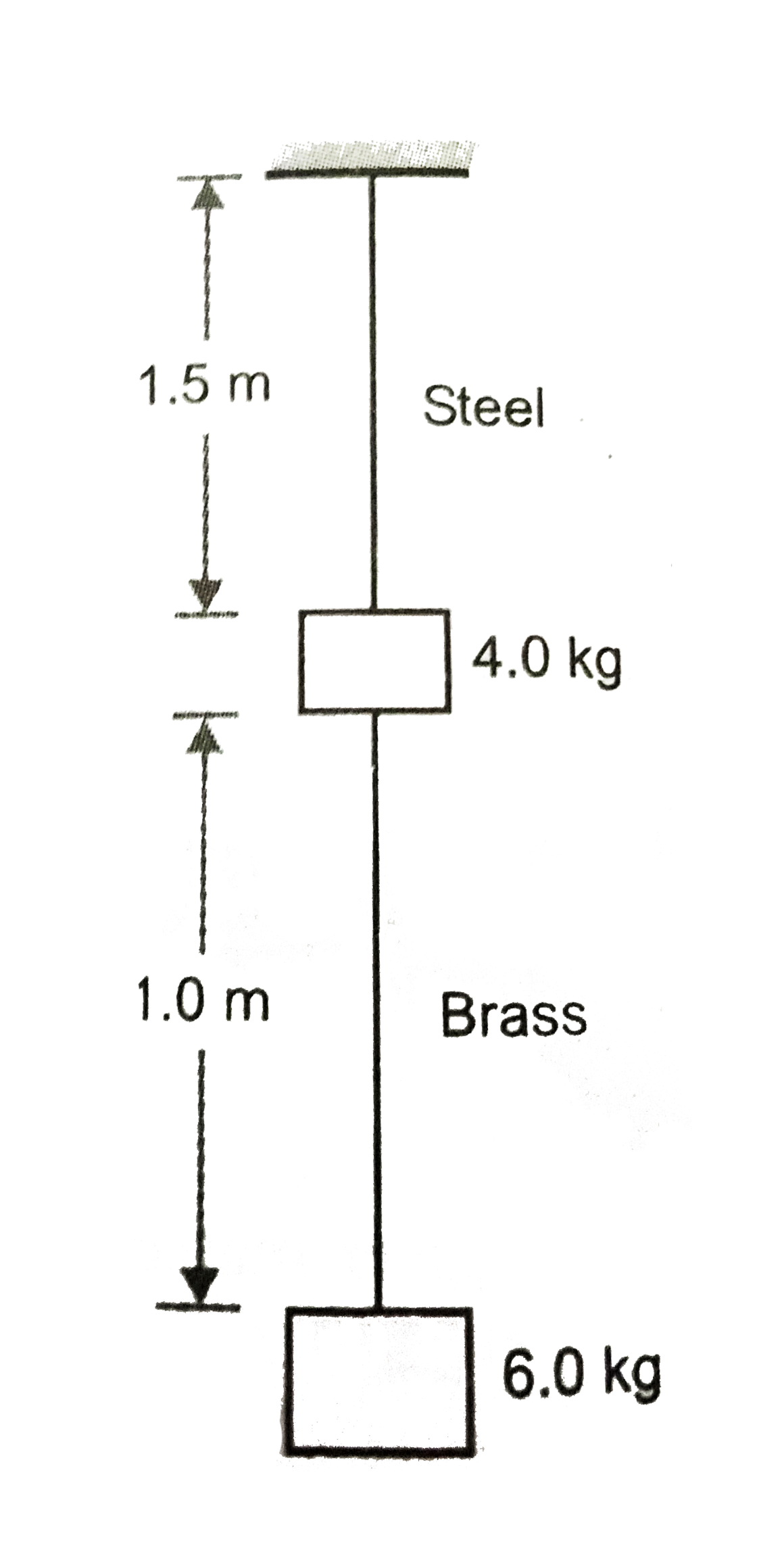
View SolutionTwo wires of diameter 0.25cm, one made of steel and other made of brass, are loaded as shown in the figure. The unloaded length of the steel wire is 1.5m and that of brass is 1.0m. Young's modulus of steel is 2.0×1011Pa and that of brass is 1.0×1011 Pa. Compute the ratio of elongations of steel and brass wires. △lsteel△lbrass=?

View SolutionTwo wires of diameter 0.25cm, one made of steel and other made of brass, are loaded as shown in the figure. The unloaded length of the steel wire is 1.5m and that of brass is 1.0m. Young's modulus of steel is 2.0×1011Pa and that of brass is 1.0×1011 Pa. Compute the ratio of elongations of steel and brass wires. △lsteel△lbrass=?

View SolutionA steel wire of length 600cm and diameter 1.2 mm is stretched through 4 mm by a load. Calculate the work done. Young's modulus of steel =2×1011Nm−2.
View SolutionIn a cylinder whose diameter is exactly 1.0 cm at 30∘C is to be slid into a hole of diameter 0.9997 cm in a steel plate at the same temperature. The minimum required rise in temperature of the plate is (The coefficient of linear expansion of steel = 12×10−6/∘C ) :
View Solution
