Two wires of diameter 0.25 cm, one made of steel and the other made of brass are loaded as shown in fig. The unloaded length of steel wire is $1.5 m$ and that of brass wire is 1.0m. Compute the elongations of the steel and the brass wires.

Video Solution

Text Solution

Verified by Experts

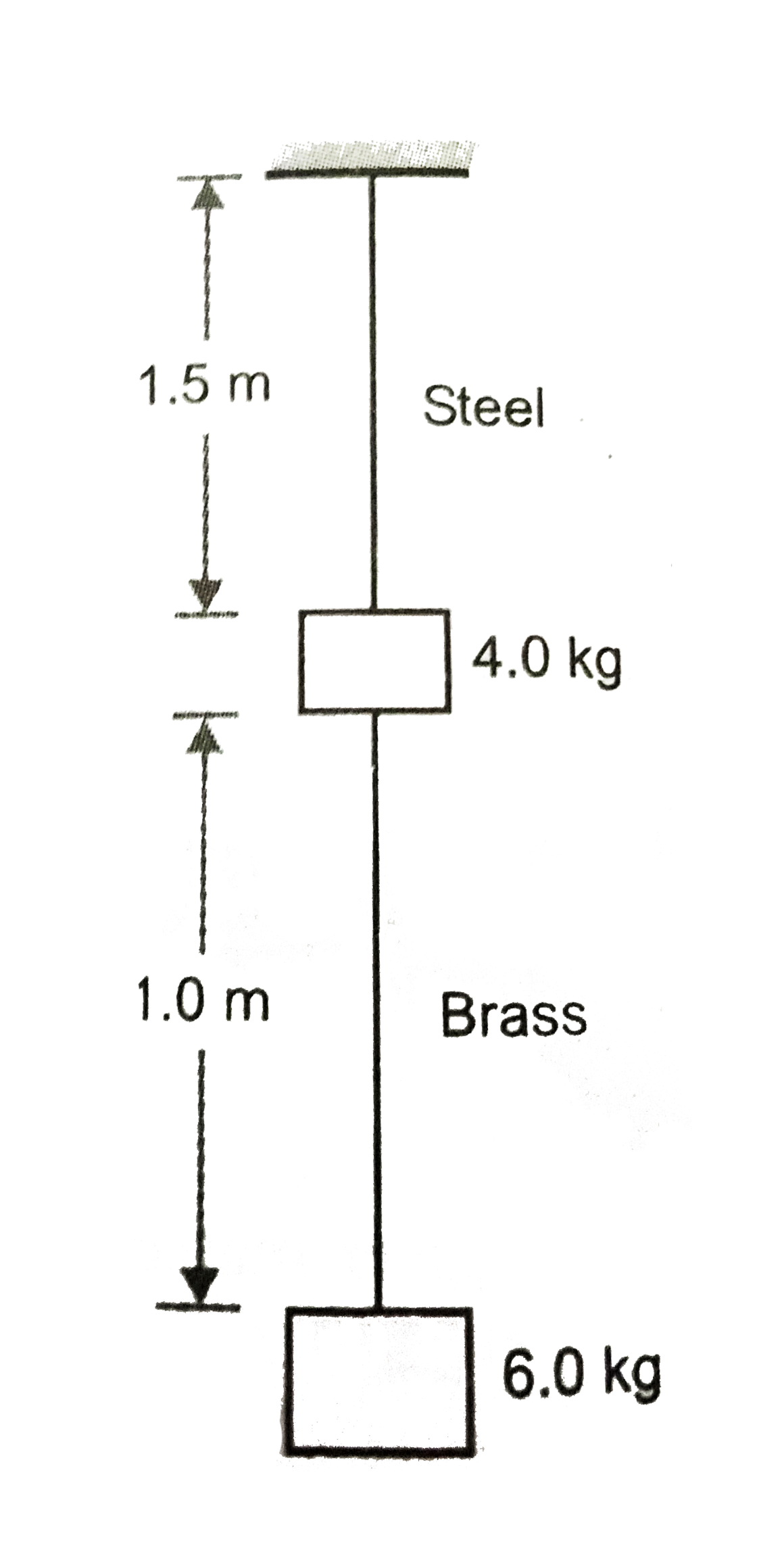
Elongation of the steel wire = $1.49 \times 10^{- 4} m$
Elongation of the brass wire = $1.3 \times 10^{- 4} m$
Diameter of the wires, d = 0.25 m
Hence, the radius of the wires, $r = \frac{d}{2}$ = 0.125 cm
Length of the steel wire, $L_{1}$ = 1.5 m
Length of the brass wire, $L_{2}$ = 1.0 m
Total force exerted on the steel wire:
$F_{1} = (4 + 6) g = 10 \times 9.8$ = 98 N
Young's modulus for steel:
$Y_{1} = \frac{(\frac{F_{1}}{A_{1}})}{(\frac{Δ L_{1}}{L_{1}})}$
Where,
$Δ L_{1}$ = Change in the length of the steel wire
$A_{1}$ = Area of cross-section of the steel wire = $π r_{1}^{2}$
Young’s modulus of steel, $Y_{1} = 2.0 \times 10^{11}$ pa
$∴ Δ L_{1} = \frac{F_{1} \times L_{1}}{A_{1} \times Y_{1}} = \frac{F_{1} \times L_{1}}{π r_{1}^{2} \times Y_{1}}$
$= \frac{98 \times 1.5}{π {(0.125 \times 10^{- 2})}^{2} \times 2 \times 10^{11}} = 1.49 \times 10^{- 4} m$
Total force on the brass wire: $F_{2} = 6 \times 9.8 = 58.8 N$
Young’s modulus for brass:
$Y_{2} = \frac{(\frac{F_{2}}{A_{2}})}{(\frac{Δ L_{2}}{L_{2}})}$
Where,
$Δ L_{2}$ = Change in length
$A_{2}$ = Area of cross-section of the bass wire
$∴ Δ L_{2} = \frac{F_{2} \times L_{2}}{A_{2} \times Y_{2}} = \frac{F_{2} \times L_{2}}{π r_{1}^{2} \times Y_{2}}$
$\frac{58.8 \times 1.0}{π \times {(0.125 \times 10^{- 2})}^{2} \times (0.91 \times 10^{11})} = 1.3 \times 10^{- 4} m$
Elongation of the steel wire = 1.49 $\times$ $10^{- 4}$ m
Elongation of the brass wire = 1.3 $\times$ $10^{- 4}$ m

Updated on:21/07/2023

|

Class 11 PHYSICS PROPERTIES OF SOLIDS AND FLUIDS

Topper's Solved these Questions

Similar Questions

Knowledge Check

A 10 m long steel wire has mass 5 g. If the wire is under a tension of 80 N, the speed of transverse waves on the wire is
A $100 m s^{- 1}$
B $200 m s^{- 1}$
C $400 m s^{- 1}$
D $500 m s^{- 1}$

NCERT ENGLISH-MECHANICAL PROPERTIES OF SOLIDS-EXERCISE

Home
Ask Doubt
Profile