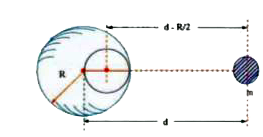
A spherical hole is made in a lead sphere of radius R, such that its surface touches the outside surface of the lead sphere and passes through its centre. The mass of the sphere before hollowing was M. With what gravitational force will the hollowed-out lead sphere attract a small sphere of mass m, which lies at a distance d from the centre of the lead sphere on the straight line connecting the centres of the sphere and of the hollow?
Volume of the sphere removed v=43π(R2)3
Mass of the sphere removed
M.=M43πR3×43π(R2)3=M8
The force on the small sphere of mass m = Force due to sphere of radius R with the distance d -Force due to sphere of radius R/2 with the distance (d-R/2)
i.e.,F=GMmd2−GM.m(d−R/2)2
=GMmd2−G(M8)m(d−R/2)2
=GMm[1d2−18(d−R/2)2]=GMmd2[1−18(1−R/2d)2]
Similar Questions
A spherical hollow is made in lead sphere of radius R, such that its surface touche the outside surface of the lead sphere and passes though its centre. The mass of the sphere before hollowing sphere exert on a point mass m placed at the centre of the hollow?

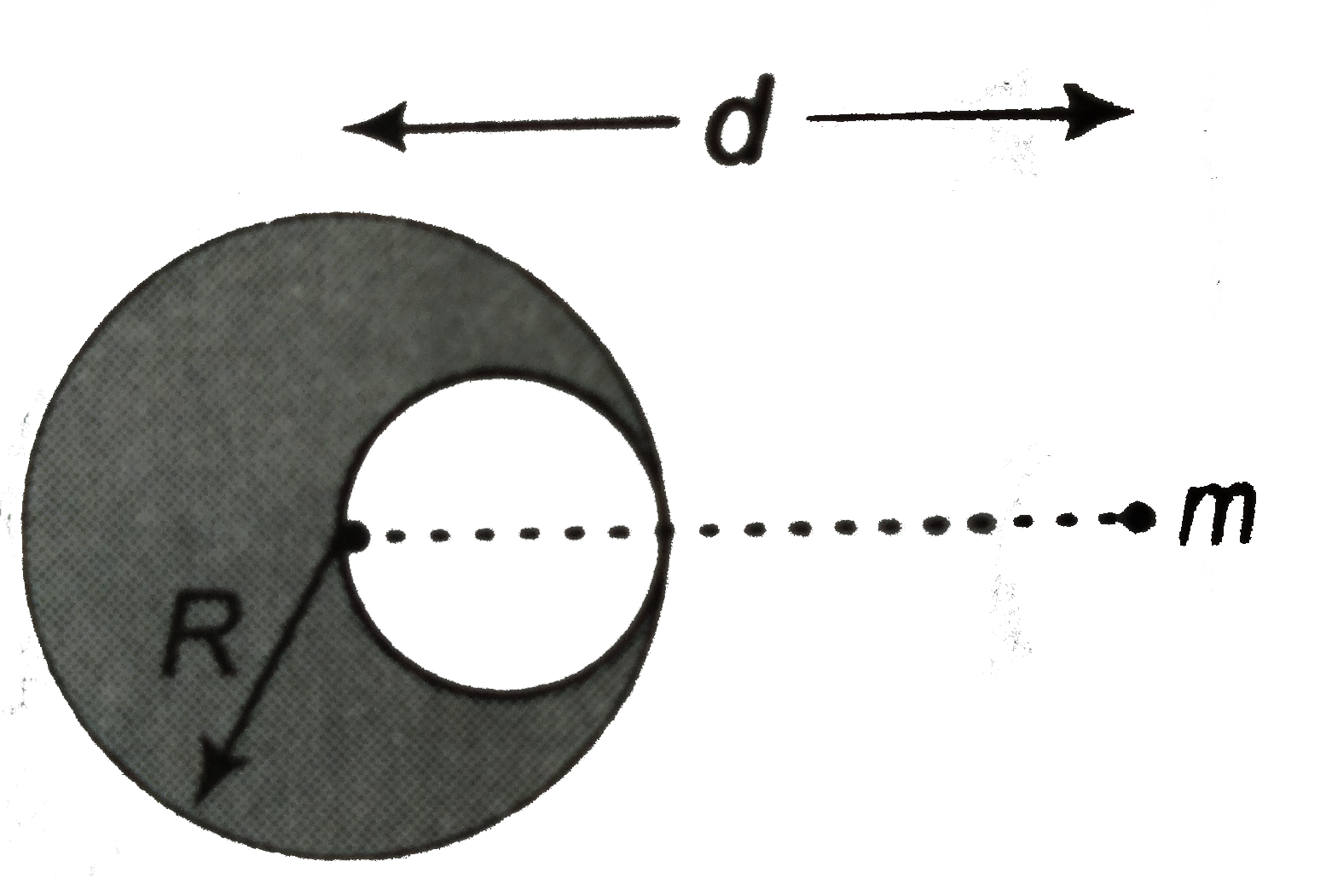
View SolutionFigure shows a spherical cavity inside a lead sphere. The surface of the cavity passes through the centre of the sphere and touches the right side of the sphere. The mass of the sphere before hollowing was M. With what gravitational force does the hollowed out lead sphere attract a particle of mass m that lies at a distance d from the centre of the lead sphere on the straight line connecting the centres of the spheres and of the cavity.
View Solution
Knowledge Check
A spherical hollow cavity is made in a lead sphere of radius R such that is surface touches the outside surface of the lead sphere and passes through its centre. The mass of the sphere before hollowing was M with what gravitational force will the hollowed out lead sphere attract a small sphere of mass m which lies at a distacne d from the centre of the lead sphere on the straight line connecting the centeres of the spheres and that of the hollow if d=2R
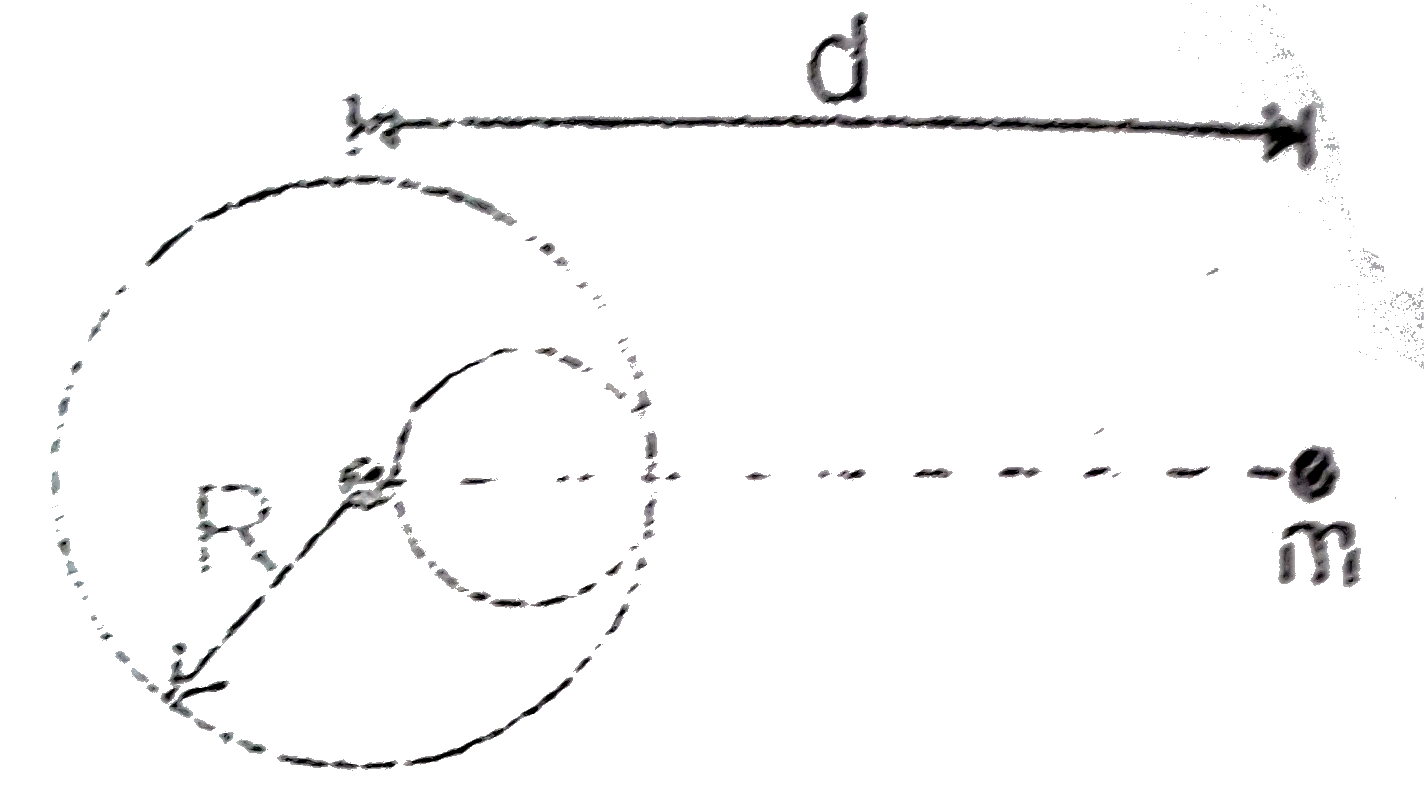 .A7GMm18R2B7GMm36R2C7GMm9R2D7GMm72R2
.A7GMm18R2B7GMm36R2C7GMm9R2D7GMm72R2A spherical hollow is made in a lead sphere of radius R such that its surface touches the outside surface of the lead sphere and passes through the centre. The mass of the lead sphere before hollowing was M. The force of attraction that this sphere would exert on a particle of mass m which lies at a distance d from the centre joining the centre of the sphere and the hollow is
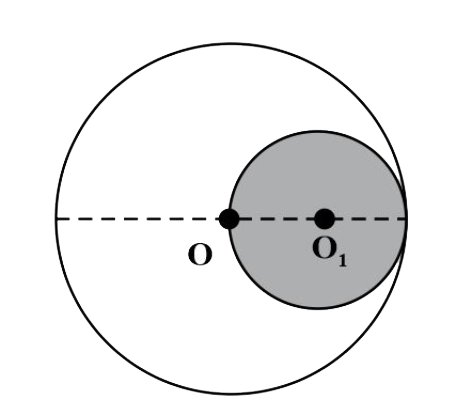
AGMmd2BGMmd2⎡⎢ ⎢ ⎢⎣1−18(1−R2d)2⎤⎥ ⎥ ⎥⎦CGMmd2⎡⎢ ⎢ ⎢⎣1−18(1+R2d)2⎤⎥ ⎥ ⎥⎦DGMm8d2A spherical cavity is made in a lead sphere of radius R such that its surface touches the outsides surface of lead sphere and passes through the centre. The shift in the centre of mass of the lead sphere as a result of this following, is
 AR7BR14CR2DR
AR7BR14CR2DR
Figure shows a spherical cavity inside a lead sphere. The surface of the cavity passes through the centre of the sphere and touches the right side of the sphere. The mass of the sphere before hollowing was M. With what gravitational force does the hollowed out lead sphere attract a particle of mass m that lies at a distance d from the centre of the lead sphere on the straight line connecting the centres of the spheres and of the cavity.

View SolutionA spherical cavity is made in a lead sphere of radius R such that its surface touches the outsides surface of lead sphere and passes through the centre. The shift in the centre of mass of the lead sphere as a result of this following, is

View SolutionA spherical hole is made in a solid sphere of radius R. The mass of the sphere before hollowing was M. The gravitational field at the centre of the hole due to the remaining mass is
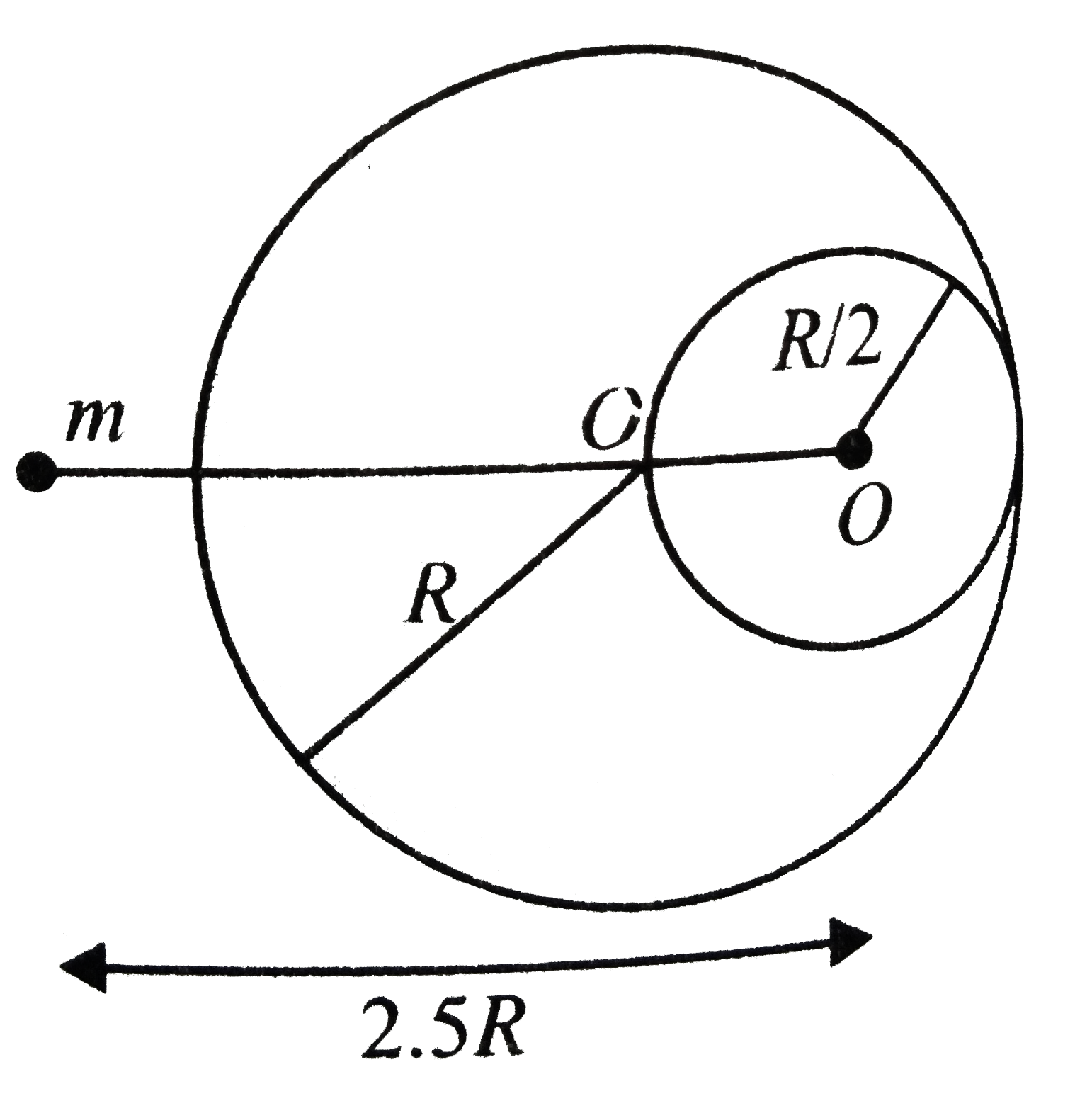
View SolutionA solid sphere of radius R/2 is cut out of a solid sphere of radius R such that the spherical cavity so formed touches the surface on one side and the centre of the sphere on the other side, as shown. The initial mass of the solid sphere was M. If a particle of mass m is placed at a distance 2.5R from the centre of the cavity, then what is the gravitational attraction on the mass m?
View SolutionA spherical hole is made in a solid sphere of radius R. The mass of the sphere before hollowing was M. The gravitational field at the centre of the hole due to the remaining mass is

View Solution
