A particle of mass m is located outside a uniform sphere of mass M at a distance r from its centre. Find:
(a) the potential energy of gravitational interaction of the particle and the sphere,
(b) the gravitational force which the sphere exerts on the particle.

Text Solution

Verified by Experts

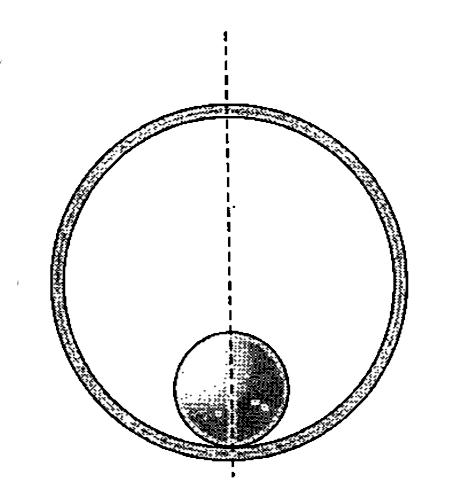
Suppose that the sphere has a radius equal to a. We may imagine that the sphere is made up of concentric thin spherical shells (layers) with radii ranging from 0 to a, and each spherical layer is made up of elementary bands (rings). Let us first calculate potential due to an elementary band of a spherical layer at the point of location of the point mass m (say point P) (figure). As all the points of the band are located at the distance l from the point P, so,
$δ φ = - \frac{γ δ M}{l}$ (where mass of the band) (1)
$δ M = (\frac{d M}{4 π a^{2}}) (2 π a sin θ) (a d t h η)$
$= (\frac{d M}{2}) sin θ d θ$ (2)
And $l^{2} = a^{2} + r^{2} - 2 a r cos θ$ (3)
Differenciating Eq. (3), we get
$l d l = a r sin θ d θ$ (4)
Hence using above equations
$δ φ = - (\frac{λ d M}{2 a r}) d l$ (5)
Now integrating this Eq. over the whole spherical layer
$d φ = \int δ φ = - \frac{γ d M}{2 a r} ¯ r + a \int r = - a$
So $d φ = - \frac{γ d M}{r}$ (6)
Equation (6) demonstrates that the potential produced by a thin uniform spherical layer outside the layer is such as if the whole mass of the layer were concentrated at it's centre,
Hence the potential due to the sphere at point P,
$φ = \int d φ = - \frac{γ}{r} \int d M - \frac{γ M}{r}$ (7)
This expression is similar to that of Eq. (6)
Hence the sought potential energy of gravitational interaction of the particle m and the sphere,
$U = m φ = - \frac{γ M m}{r}$
(b) Using the Eq. $G_{r} = - \frac{δ φ}{δ r}$
$G_{r} = - \frac{γ M}{r^{2}}$ (using Eq. 7)
So $\to G = - \frac{γ M}{r^{3}} \to r$ and $\to F = m \to G = - \frac{γ m M}{r^{3}} \to r$ (8)

Updated on:21/07/2023

A uiform ring of mass M and radius R is placed directly above a uniform sphere of mass 8M and same radius R. The centre of the sphere. The gravitational atraction between the sphere and the ring is
A $\frac{8 G M^{2}}{R^{2}}$
B $\frac{2 G M^{2}}{\sqrt{3} R^{2}}$
C $\frac{3 G M^{2}}{2 R^{2}}$
D $\frac{\sqrt{3}}{2} \frac{G M^{2}}{R^{2}}$
A uniform ring of mass $m$ and radius $a$ is placed directly above a uniform sphere of mass $M$ and of equal radius. The centre of the ring is at distance $\sqrt{3} a$ from the centre of the sphere. Find the gravitational force exerted by the sphere on the ring.
A $\frac{G M m}{8 r^{2}}$
B $\frac{G M m}{4 r^{2}}$
C $\sqrt{3} \frac{G M m}{8 r^{2}}$
D $\frac{G M m}{8 r^{3} \sqrt{3}}$
A particle placed
1.inside a uniform spherical shell of mass M, but not at the center
2.inside a uniform spherical shell of mass M, at the center
3.outside a uniform spherical shell of mass M, a distance r from the center
4.outside a uniform solid sphere of mass M, a distance 2r from the center
If $F_{1}, F_{2}, F_{3}$ and $F_{4}$ are gravitational forces acting on the particle in four cases.
A $F_{1} > F_{2} > F_{3} > F_{4}$
B $F_{1} = F_{2} < F_{3} < F_{4}$
C $F_{1} = F_{2} < F_{4} < F_{3}$
D $F_{1} < F_{2} < F_{3} < F_{4}$