Two straight thin bars, one of brass and the other of steel are joined together side by side by short steel crosspieces at 0∘C, one cm long, the centre lines of the bars being one cm apart. When heated to 100∘C, the composite bar becomes bent into the arc of a circle. Calculate the radius of this circle. α for brass =19×10−6per ∘C, and α for steel =11×10−6 per ∘C
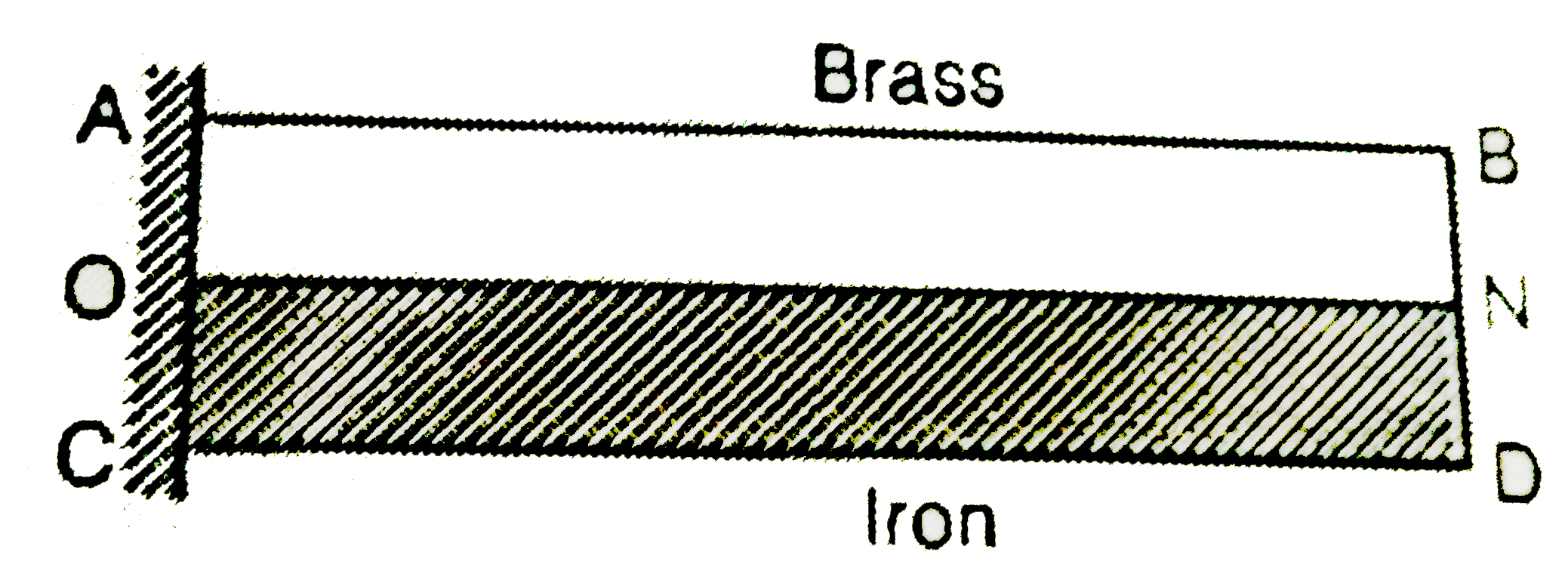
As the expansion of brass of greater, the combination will bend with brass rod outside as shown in figure. In figure, AB is brass bar and CD is steel bar.

Let OC = R and ∠COD=θ
Length of steel cross pieces at 100∘C
=1(1+11×10−6×100)=1.0011cm
Now OA=(R+1.0011)cm ...(a)
and CD=Rθ
and AB=(R+1.0011)θ ...(b)
Also, AB=L0(1+19×10−6×100) ....(c)
and CD=L0(1+11×10−6×100) ...(d)
From equation (a), (b), (c ) and (d), we have
ABCD=R+1.0011R=(1+19×10−6×100)(1+11×10−6×100)(or)1+1.001R=1+19×10−41+11×10−4
1.0011R=8×10−41+11×10−4(or)R=(1.0011)
=(1+11×10−4)8×10−4=1252.8cm
Topper's Solved these Questions
- BOOK - AAKASH SERIESCHAPTER - EXPANSION OF SOLIDS EXERCISE - Test your self6videos
EXPANSION OF SOLIDS
- BOOK - AAKASH SERIESCHAPTER - EXPANSION OF SOLIDS EXERCISE - Very Short Answer Type Questions11videos
EXPANSION OF SOLIDS
- BOOK - AAKASH SERIESCHAPTER - EXPANSION OF LIQUIDS EXERCISE - QUESTIONS FOR DESCRIPTIVE ANSWERS4videos
EXPANSION OF LIQUIDS
- BOOK - AAKASH SERIESCHAPTER - FLUID MECHANICSEXERCISE - Questions for Descriptive Answers14videos
FLUID MECHANICS
Similar Questions
Two straight thin bars, one of brass and the other of steel are joined togethre side by side by short steel cross-pieces at 0∘C, one cm long the centre lines of the bars being one cm apart. When heated to 100∘C, the composite bar becomes bent into the arc of a circle. calculate the radius of this circle.
α for brass =19×10−6 per .∘C
and α for steel =11×10−6 per .∘CView SolutionA bimetallic strip consiting of a brass strip and a steel strip, each of length 1m and thickness 1/2 cm is clamped at one end at 0∘C.Calculate the depression of the free end when it is heated to 100∘C.
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
Two thin metal strips, one of brass and the other of iron are fastended together parallel to each other. Thickness of each strip is 2mm. If the strips are of equal length at 0∘C. The radius of the are formed by the bimetaalic strip when heated to 80∘C is (Coefficient of linear expansion of brass=19×10−6/∘C&
of iron=12×10−6/∘C).A3.57mB2.67mC3.12mD4.56m - Question 2 - Select One
A brass rod and a lead rod each 80 cm long at 0∘C are clamped together at one end with their free ends coinciding. The separatioin of free ends of the rods if the system is placed in a steam bath is (αbrass=18×10−6/∘C and αlead=28×10−6/∘C)
A0.2 mmB0.8 mmC1.4 mmD1.6 mm
A brass rod and a lead rod each 80 cm long at 0∘C are clamped together at one end with their free ends coinciding. The separatioin of free ends of the rods if the system is placed in a steam bath is (αbrass=18×10−6/∘C and αlead=28×10−6/∘C)
View SolutionA brass rod and a lead rod each 80 cm long at 0∘C are clamped together at one end with their free ends coinciding. The separatioin of free ends of the rods if the system is placed in a steam bath is (αbrass=18×10−6/∘C and αlead=28×10−6/∘C)
View SolutionA steel bar of cross sectional area 1cm2 and 50cm long at 300C fits into the space between two fixed supports.. If the bar s now heated to 2800C, what force will it exert against the supports ? (⋅ for steel =11×106/0C and Young's modulus for steel =2×1011N/m2)
View SolutionIn a certain arrangement, two cylinders of brass and steel are laid side by side and there is a constant difference of length between them at any temperature. Calculate the lengths of these cylinders at 0∘C if there is a difference of 5 cm between their lengths at all temperatures.
αbrass=0.0000019∘C−1
αsteel=1.2×10−6C−1View SolutionWhat should be the length of steel and copper rods at 0∘C that the length of steel rod is 5 cm longer than copper at all termperature? Given αCu=1.7×105.∘C−1 and αsteel=1.1×105.∘C−1.
View SolutionWhat should be the length of steel and copper rods at 0∘C that the length of steel rod is 5 cm longer than copper at all termperature? Given αCu=1.7×105.∘C−1 and αsteel=1.1×105.∘C−1.
View Solution

