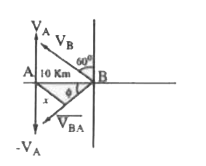
Ship A is 10 km due west of ship B. Ship A heading directly north at a speed of 30 kmph while ship B is heading in a direction $60^{\circ}$ west of north at a speed 20 kmph. Their closest distance of approach will be…….

Text Solution

Verified by Experts

$\bar{V_{A}} = 30 j \bar{V_{B}} = 20 {Sin 60}^{\circ} (\hat{i}) + 20 Cos 60 (\hat{j})$
$= - 10 \sqrt{3} \hat{i} + 10 \hat{j}$
$\bar{V_{B A}} = \bar{V_{B}} - \bar{V_{A}} = - 20 \hat{j} - 10 \sqrt{3} \hat{i}$
If $ϕ$ is the angle made by $\bar{V_{B A}}$ with X axis
$Tan ϕ = \frac{20}{10 \sqrt{3}} = \frac{2}{\sqrt{3}}$
and $Sin ϕ = \frac{2}{\sqrt{7}}$
From $Δ A B C, \frac{x}{10} = \frac{2}{\sqrt{7}}$
$x = \frac{20}{\sqrt{7}} = 7.56 K m$

|

Updated on:21/07/2023

Class 11 PHYSICS PROJECTILE MOTION

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A ship is travelling due north at 40 kmph. The captain of the finds that a second ship is always sailing $60^{\circ}$ west of north at a speed of 40 kmph. The velocity of the second ship then must be
A40 kmph, $30^{\circ}$ west of north
B40 kmph. $60^{\circ}$ west of south
C $40 \sqrt{3}$ kmph, $30^{\circ}$ west of north
D $40 \sqrt{3}$ kmph, $60^{\circ}$ west of south
Question 2 - Select One
A ship is travelling due east at a speed of $15 k m / h .$ Find the speed of a boat heading $30^{\circ}$ east of north if it appears always due north from the ship.
A $30 k m / h$
B $\frac{15 \sqrt{3}}{2} k m / h$
C $10 \sqrt{3} k m / h$
D $20 k m / h$
Question 3 - Select One or More
Ship A is located 4 km north and 3 km east of ship B. Ship A has a velocity of $20 k m h^{- 1}$ towards the south and ship B is moving at $40 k m h^{- 1}$ in a direction $37^{\circ}$ north of east. X and Y-axes are along east and north directions, respectively
AVelocity of A relative to B is $(- 32 ˆ i - 44 ˆ j) k m / h$
BPosition of A relative to B as a function of time is given by $r_{A B} = [(3 - 32 t) ˆ i + (4 - 44 t) ˆ j] k m$
CVelocity of A relative to B is $(32 ˆ i - 44 ˆ j) k \frac{m}{h}$
DPosition of A relative to B as a function of time is given by $(32 t ˆ i - 44 t ˆ j) k m$

AAKASH SERIES-MOTION IN A PLANE -Practice Exercise

Home
Ask Doubt
Profile