The escape velocities on the surface of two planets of masses m1andm2 and having the radius r1andr2arev1andv2 respectively, then
v1v2=m1r1m2r2
v2v1=m1r1m2r2
v2v1=√m2r1m1r2
v2v1=√m1r1m2r2
The correct Answer is:C
Similar Questions
Show that the escape velocity of an object from the speeds of two planets of masses 2M and 3M and radii 2R and R respectively.
View SolutionThe escape velocity corresponding to a planet of mass M and radius R is 50kms−1. If the planet's mass and radius were 4M and R, respectively, then the corresponding escape velocity would be
View Solution
Knowledge Check
The escape velocities of the two planets, of densities ρ1 and ρ2 and having same radius, are v1 and v2 respectively. Then
Av1v2=ρ1ρ2Bv2v1=ρ2ρ1Cv1v2=(ρ1ρ2)2Dv1v2=√ρ1ρ2The escape velocity on the surface of the earth is 11.2 kms−1. If mass and radius of a planet is 4 and 2 tims respectively than that of the earth, what is the escape velocity from the planet?
A11.2kms−1B1.112kms−1C15.8kms−1D22.4kms−1The escape velocity corresponding to a planet of mass M and radius R is 50kms−1. If the planet's mass and radius were 4M and R, respectively, then the corresponding escape velocity would be
A100kms−1B50kms−1C200kms−1D25kms−1
A particle of mass M at rest decays into two particles of masses m1andm2 having velocities V1andV2 respectively. Find the ratio of de- Broglie wavelengths of the two particles
View SolutionA planet of mass M, has two natural satellites with masses m1 and m2. The radii of their circular orbits are R1 and R2 respectively. Ignore the gravitational force between the satellites. Define v1,L1,K1 and T1 to be, respectively, the orbital speed, angular momentum, kinetic energy and time period of revolution of satellite 1 , and v2,L2,K2 and T2 to be he corresponding quantities of satellite 2. Given m1/m2=2 and R1/R2=1/4, match the ratios in List-I to the numbers in List-II.
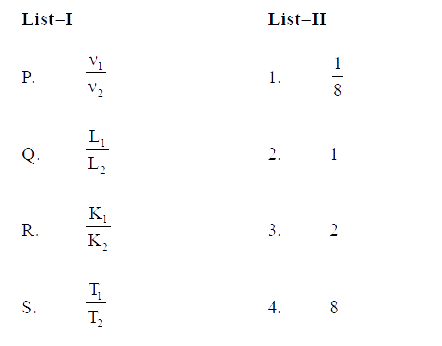
View SolutionSuppose there are two planets, 1 and 2, having the same density but their radii are R1andR2 respectively, where R1>R2. The accelerations due to gravity on the surface of these planets are related as
View SolutionTwo satellites of masses m1 and m2(m1>m2) are revolving around earth in circular orbits of radii r1 and r2(r1>r2) respectively. Which of the following statements is true regarding their velocities V1 and V2
View SolutionThe ratio of accelerations due to gravity g1 : g2 on the surfaces of two planets is 5 : 2 and the ratio of their respective average densities ρ1 : ρ2 is 2 : 1. what is the ratio of respective escape velocities v1 : v2 from the surface of the planets?
View Solution
