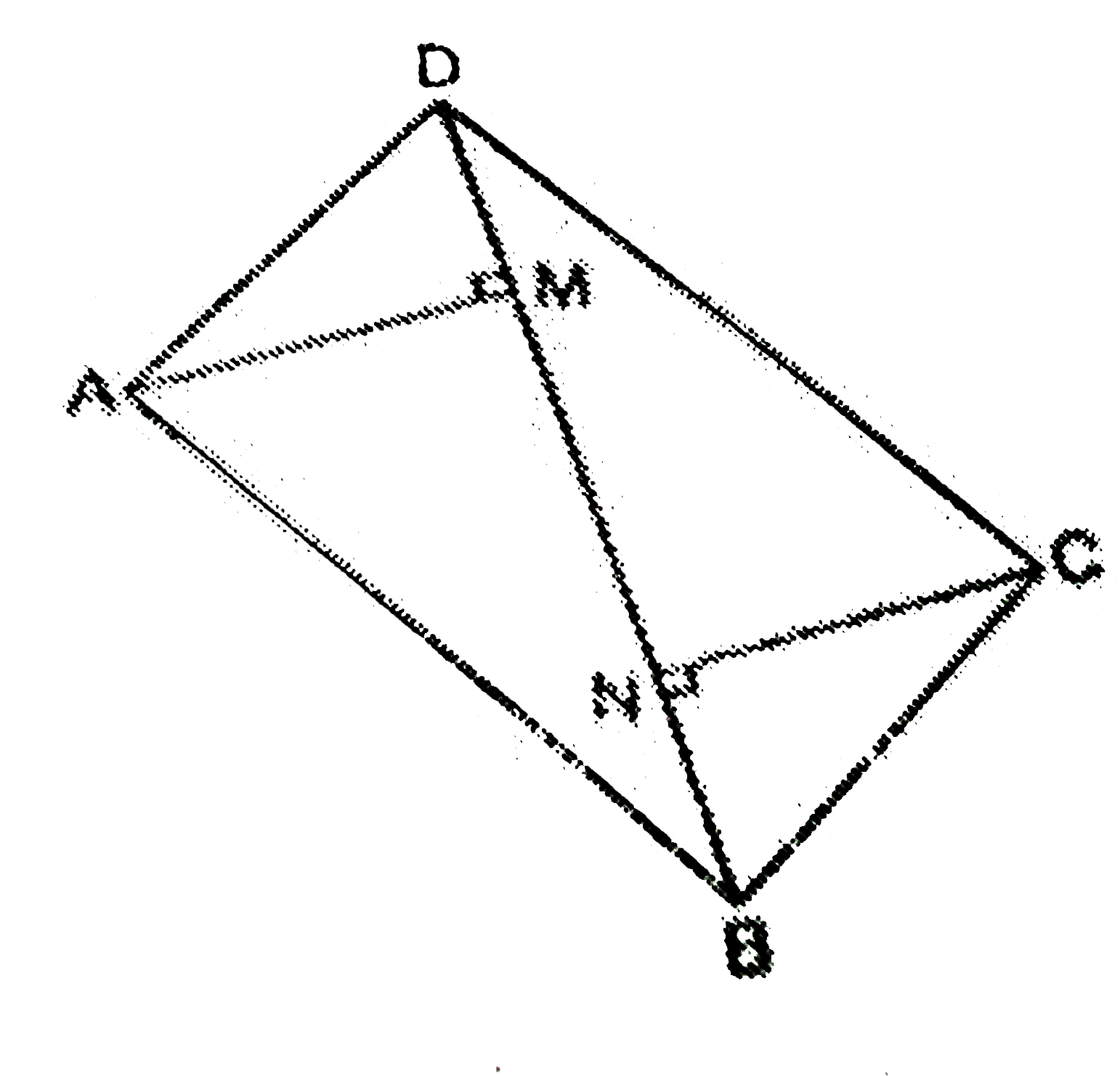
In quadrilateral ABCD, AM and CN are altitudes on diagonal BD drawn from A and C respectively .Prove that
ar(ABCD)=12 BD(AM+CN) Solution in Gujrati
Step by step video & image solution for In quadrilateral ABCD, AM and CN are altitudes on diagonal BD drawn from A and C respectively .Prove that ar(ABCD)=1/2 BD(AM+CN) by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Updated on:21/07/2023Topper's Solved these Questions
- AREAS OF PARALLELOGRAMS AND TRIANGLESBOOK - KUMAR PRAKASHANCHAPTER - AREAS OF PARALLELOGRAMS AND TRIANGLESEXERCISE - Multiple Choice Questions (MCQs)
17 Videos
- AREAS OF PARALLELOGRAMS AND TRIANGLESBOOK - KUMAR PRAKASHANCHAPTER - AREAS OF PARALLELOGRAMS AND TRIANGLESEXERCISE - Sum to Enrich Remember
4 Videos
- BOARD'S SAMPLE QUESTION PAPERS (QUESTION PAPER 1 : FOR THE FIRST TEST) BOOK - KUMAR PRAKASHANCHAPTER - BOARD'S SAMPLE QUESTION PAPERS (QUESTION PAPER 1 : FOR THE FIRST TEST) EXERCISE - Section D (Solve the following)
4 Videos
Similar Questions
Area of a parallelogram =_____
View SolutionIn the given figure, ABED is a parallelogram and DE and EC . Prove that ar (ABF)=ar(BEC)

View SolutionYamini and Fatima , two students of class IX of a school, together contributed Rs 100 towards the prime Minister's Relief Fund to help the earthquake victims . Write a linear equation which satisfies this this data. (You may take their contributions as Rs x and Rs y). Draw the graph of the same.
View SolutionDiagonals AC and BD of a quadrilateral ABCD intersect at O in such a way thatar(AOD)=ar(BOC).Prove that ABCD is a trapezium.
View SolutionABCD is a rectangle. Points M and N are on BD such that AM⊥BD and CN⊥BD . Prove that BM2+BN2=DM2+DN2 .
View SolutionIn a quadrilateral ABCD, AM and CN are perpendiculars from the vertices A and C respectively on diagonal BD. Prove that:
area of □ABCD=12×BD×(AM+CN)
View Solutionचतुर्भुज ABCD में एक विकर्ण BD है। A तथा C से लम्ब AM तथा CN विकर्ण BD पर डाले गए है। दिखाओ कि
क्षेत्रफल (चतुर्भुज ABCD ) =12 BD (AM+CN).
View Solutionएक चतुर्भुज ABCD के विकर्ण AC तथा BD परस्पर O पर इस प्रकार प्रतिच्छेद करते हैं कि ar(ΔAOD)=ar(ΔBOC) है । सिद्ध करों कि ABCD एक समलम्ब है।
View Solutionयदि किसी चतुर्भुज ABCD का एक विकर्ण BD है। यदि शीर्ष A तथा C से विकर्ण BD पर लम्ब क्रमशः AM तथा CN हैं, तो सिद्ध कीजिए कि
ar (चतुर्भुज ABCD )=12BD(AM+CN)
View Solutionसमांतर चतुर्भुज ABCD के विकर्ण AC पर बिंदु M और N इस प्रकार लिए गये हैं कि AM=CN है। सिद्ध कीजिए कि BMDN एक समांतर चतुर्भुज है।
View Solutionएक चतुर्भुज ABCD के विकर्ण AC और BD परस्पर बिन्दु O पर इस प्रकार प्रतिच्छेद करते है कि ar(AOD) = ar(BOC) है। सिद्ध कीजिए कि ABCD एक समलम्ब है।
View Solutionचतुर्भुज ABCD के विकर्ण AC और BD परस्पर चतुर्भुज के भीतर काटते है तो
ar(ABCD)=ar(ABC)+ar(…….).
View SolutionDiagonals AC and BD of a quadrilateral ABCD intersect at O in such a way that ar (AOD) = ar (BOC) . Prove that ABCD is a trapezium.
View SolutionABCD is a rectangle. AM bot BD And CN bot BD Then prove that BM^(2) + BN^(2) = DM^(2) + DN^(2)
View Solutionचतुर्भुज ABCD का एक विकर्ण BD है। यदि AL⊥BD एवं CM⊥BD हो तो सिद्ध करें कि ar(□ABCD)=12×BD(AL+CM)
View Solution
