Let V, T, L, K and r denote the speed, time period, angular momentum, kinetic energy and radius of satellite in circular orbit, then
(a) (b)
(c) (d)
only a & b are true
only b & c are true
only a, b & d are true
only a, b & c are true
The correct Answer is:B
Topper's Solved these Questions
- GRAVITATIONALBook:AAKASH SERIESChapter:GRAVITATIONALExercise:EXERCISE -1B
Explore 46 Videos
- GRAVITATIONALBook:AAKASH SERIESChapter:GRAVITATIONALExercise:EXERCISE-2
Explore 146 Videos
- GRAVITATIONALBook:AAKASH SERIESChapter:GRAVITATIONALExercise:EXERCISE -3
Explore 154 Videos
- GRAVITATIONBook:AAKASH SERIESChapter:GRAVITATIONExercise:ADDITIONAL PRACTICE EXERCISE (LEVEL-II) PRACTICE SHEET (ADVANCED)Integer Type Questions
Explore 6 Videos
- HORIZONTAL CIRCULAR MOTIONBook:AAKASH SERIESChapter:HORIZONTAL CIRCULAR MOTIONExercise:ADDITIONAL PRACTICE EXERCISE (PRACTICE SHEET (ADVANCED) Integer Type Questions)
Explore 3 Videos
Similar Questions
Knowledge Check
If V,T,L,K and r denote speed, time period, angular momentum, kinetic energy and radius of satellite in circular orbit (a)Vαr−1,(b) Lαr1/2
(c)Tαr3/2,(d) Kαr−2Aa,b are trueBb,c are trueCa,b,d are trueDa,b,c are trueAccording to the kinetic theory of gases
(i) P∝vrms
(ii) vrms∝T
(iii) vrms∝T1/2
(iv) P∝v2rmsA(i), (iii)B(ii), (iii)C(iii), (iv)D(i), (iv)If L is the angular momentum of a satellite revolving around earth is a circular orbit of radius r with speed v , then
AL∝vBL∝rCL∝√rDL∝√v
The time period of a satellite in a circular orbit of radius R is T. The period of another satellite in a circular orbit of radius 9R is :
View SolutionName the types of interaction or intermolecular forces of which potential energy-distance function are given below
(a) E∝1r (b) E∝1r2 (c ) E∝1r3
(d) E∝1r4 (e) E∝1r6 .
View SolutionName the types of interaction or intermolecular forces of which potential energy-distance function are given below
(a) E∝1r (b) E∝1r2 (c ) E∝1r3
(d) E∝1r4 (e) E∝1r6 .
View SolutionA planet of mass M, has two natural satellites with masses m1 and m2. The radii of their circular orbits are R1 and R2 respectively. Ignore the gravitational force between the satellites. Define v1,L1,K1 and T1 to be, respectively, the orbital speed, angular momentum, kinetic energy and time period of revolution of satellite 1 , and v2,L2,K2 and T2 to be he corresponding quantities of satellite 2. Given m1/m2=2 and R1/R2=1/4, match the ratios in List-I to the numbers in List-II.
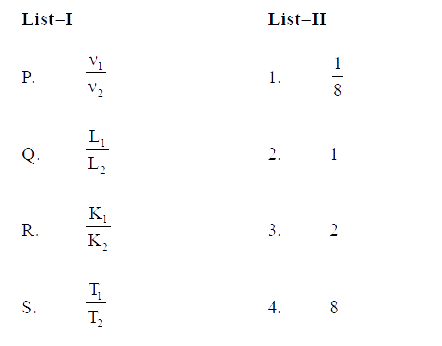
View SolutionThe period of a satellite in a circular orbit of radius R is T. The period of another satellite in circular orbit of radius 4 R is :
View Solution
