A body starts from rest and travels a distance S with uniform acceleration, then moves uniformly a distance 2S and finally comes to rest after moving further 5S under uniform retardation. Find the ratio of average velocity to maximum velocity :
Graphically : Area of (V−t) curve represent displacement
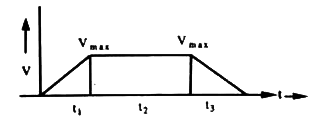
S=12Vmaxt1ort1=2SVmax,
2S=Vmaxt2ort2=2SVmax
5S=12Vmaxt3ort3=10SVmax
Vav=Total displacementTotal time,Vav=S+2S+5S2SVmax+2SVmax+10SVmax
VavVmax=8S14S=47(OR)
VavVmax=Total displacement−−−−−−−−−−−−−−−−−−−−−−−−−−−−−→2⎛⎜⎝total displacementduring accelerationand retardation⎞⎟⎠+⎛⎜⎝DisplacementDuring uniformvelocity⎞⎟⎠
∴VavVmax=8S2(S+5S)+2S=814=47
Similar Questions
A body starts from rest and travels a distance S with unitorm acceleration, then moves uniformly a distance 2S uniformly , and finally cones to rest after moving further 5S under uniform retardation. The ratio of the average velocity to maximum velocity is.
View SolutionA particle starts from rest and traverses a distance l with uniform acceleration, then moves uniformly over a further distance 2l and finally comes to rest after moving a further distance 3l under uniform retardation. Assuming entire motion to be rectilinear motion the ratio of average speed over the journey to the maximum speed on its ways is
View Solution
Knowledge Check
A body starts from rest and travels a distance S with uniform acceleration, then moves uniformly a distance 2S uniformly , and finally cones to rest after moving further 5S under uniform retardation. The ratio of the average velocity to maximum velocity is.
A2/5B3/5C4/7D5/7A particle starts from rest and travel a distance x with uniform acceleration, then moves uniformly a distance 2x and finally comes to rest after moving further 5x with uniform retardation. The ratio of maximum speed to average speed is
A52B53C74D75A particle starts from rest and traverses a distance 2x with uniform acceleration, then moves uniformly over a further distance 4x and finally comes to rest after moving a further distance 6x under uniform retardation. Assuming entire motion to be rectilinear motion, the ratio of average speed over the journey to the maximum speed on its way is
A45B35C25D15
A partical starts from rest and moves in a straight line. It travels a distance L with uniform acceleration and then moves with constant velocity a further distance 2L. Finally, it comes to rest after moving a further distance 4L under uniform retardation. Find the ratio of average speed to the maximum speed.
View SolutionA body starts from rest and travels a distance x with uniform acceleration, then it travels a distance 2x with uniform speed, finally it travels a distance 3x with uniform retardation and comes to rest. If the complete motion of the particle is along a straight line, then the ratio of its average velocity to maximum velocity is
View SolutionA body starts from rest and travels a distance x with uniform acceleration, then it travels a distance 2x with uniform speed, finally it travels a distance 3x with uniform retardation and comes to rest. If the complete motion of the particle is along a straight line, then the ratio of its average velocity to maximum velocity is
View SolutionA particle starts from rest and traverses a distance l with uniform acceleration, then moves uniformly over a further distance 2l and finally comes to rest after moving a further distance 3l under uniform retardation. Assuming entire motion to be rectilinear motion the ratio of average speed over the journey to the maximum speed on its ways is
View SolutionA particle starts from rest and travels a distance s with uniform acceleration, then it travels a distance 2s with uniform speed finally it travels a distance 3s with uniform retardation and comes to rest. If the complete motion of the particle is a straight line then the ratio of its average velocity to maximum velocity is:
View Solution
