The radius of a planet is and a satellite revolve round of in a circule of radius . The time period of revolution is T. The acceleration due to the gravitation of the planet at its surface is
Orbital velocity of the satellite
Where
and angular velocity
But time period of revolution
(or) (or)
Topper's Solved these Questions
- GRAVITATIONALBOOK - AAKASH SERIESCHAPTER - GRAVITATIONALEXERCISE - EXERCISE -1A
262 Videos
- GRAVITATIONALBOOK - AAKASH SERIESCHAPTER - GRAVITATIONALEXERCISE - EXERCISE -1B
46 Videos
- GRAVITATIONBOOK - AAKASH SERIESCHAPTER - GRAVITATIONEXERCISE - ADDITIONAL PRACTICE EXERCISE (LEVEL-II) PRACTICE SHEET (ADVANCED)Integer Type Questions
6 Videos
- HORIZONTAL CIRCULAR MOTIONBOOK - AAKASH SERIESCHAPTER - HORIZONTAL CIRCULAR MOTIONEXERCISE - ADDITIONAL PRACTICE EXERCISE (PRACTICE SHEET (ADVANCED) Integer Type Questions)
3 Videos
Similar Questions
The radius of a planet is R1 and a satellite revolves round it in a circle of radius R2. The time period of revolution is T. find the acceleration due to the gravitation of the plane at its surface.
View SolutionThe radius ofa planet is R1and a satellite revolves round it in a circle of radius R2. The tiemperiod of revolution is T. find the acceleration due to the gravitational of the plane at its surface.
View Solution
Knowledge Check
The radius of a planet is R. A satellite revolves around it in a circle of radius r with angular velocity ω0. The acceleration due to the gravity on planet's surface is
Ar3ω0RBr3ω20R2Cr3ω20RDr3ω20R2The radius of a planet is n times the radius of earth (R). A satellite revolves around it in a circle of radius 4nR with angular velocity ω. The acceleration due to gravity on planet's surface is
ARω2B16 Rω2C32nRω2D64nRω2Two satellites of masses M and 16 M are orbiting a planet in a circular orbitl of radius R. Their time periods of revolution will be in the ratio of
A1:1B1:4C4:1D1:16
The radius of a planet is R. A satellite revolves around it in a circle of radius r with angular velocity ω0. The acceleration due to the gravity on planet's surface is
View Solution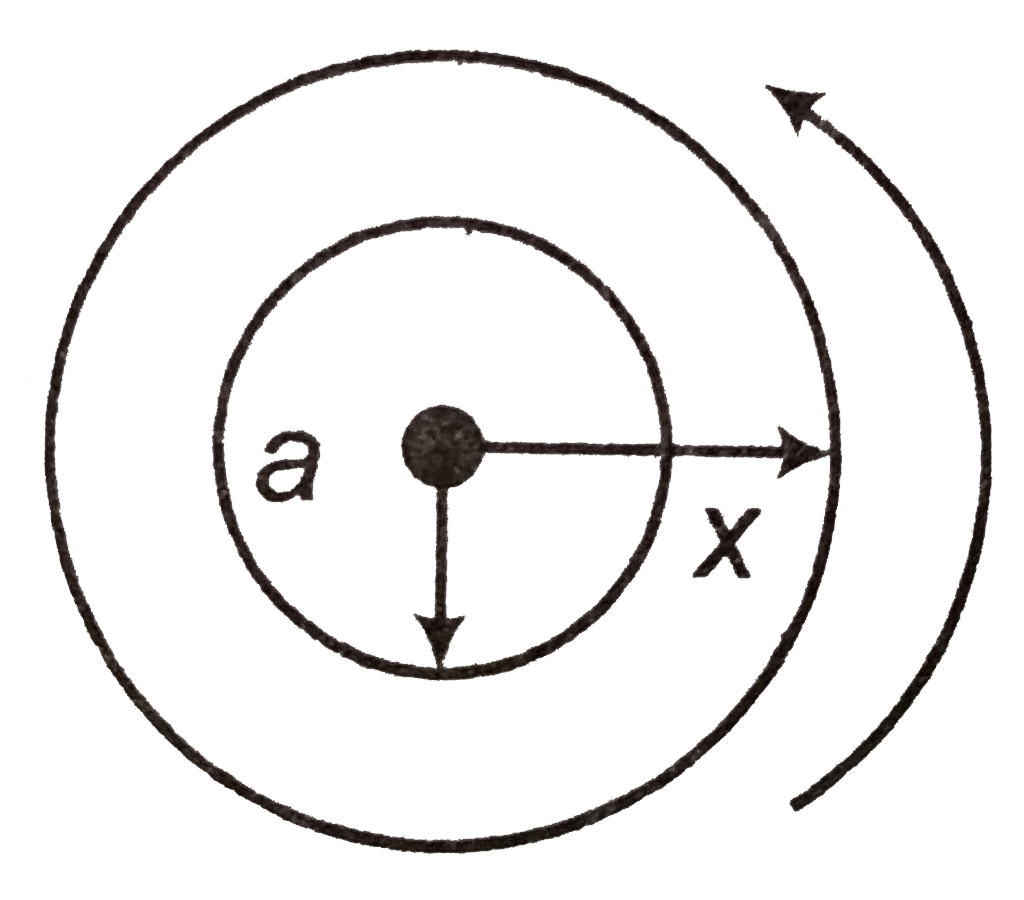
The radius of a planet is a. satelite revolves around it in a circle of radius x with angular velocity ω. The acceleration due to the gravity on planet's surface is
View SolutionTwo satellites of masses M and 16 M are orbiting a planet in a circular orbitl of radius R. Their time periods of revolution will be in the ratio of
View SolutionRadius of a geostationary satellite revolving round the earth is 'r'. Then period of revolution of another satellite revolving in an orbit of radius r/2 is
View SolutionImagine a light planet revolving around a very massive star in a circular orbit of radius R with a period of revolution T. If the gravitational force of attraction between the planet and the star is proportional to R−5/2, then T2 is proportional to
View Solution
