A bus accelerates from rest at a constant rate α for some time, after which it decelerates at a constant rate β to come to rest. If the total time elapsed is t seconds then, evaluate.
(a) the maximum velocity achieved and
(b) the total distance travelled graphically.
(a) Let t1 be the time of acceleration and t2 that of deceleration of the bus.
The total time is t=t1+t2.
Let vmax be the maximum velocity.
As the acceleration and deceleration are constant the velocity time graph is a straight line as shown in the figure with +ve slope for acceleration and −ve slope for deceleration.
From the graph,
the slope of the line OA gives the acceleration α.
∴α = slpe of the line OA=vmaxt1⇒t1=vmaxα the slope of AB gives the deceleration β
∴β = slope of AB=vmaxt2⇒t2=vmaxβ
t=t1+t2=vmaxα+vmaxβ
t=vmax(α+βαβ)
∴vmax=(αβα+β)t
(b) Displacement = area under the v-t graph
= area of ΔOAB
=12(base)(height)=12tvmax
=12t(αβtα+β)=12(αβt2α+β)
Similar Questions
A car accelerates from rest at a constant rate α for some time, after which it decelerates at a constant rate β, to come to rest. If the total time elapsed is t seconds. Then evalute (a) the maximum velocity reached and (b) the total distance travelled.
View SolutionA car accelerates from rest at a constant rate α for some time, after which it decelerates at a constant rate β, to come to rest. If the total time elapsed is t seconds. Then evalute (a) the maximum velocity reached and (b) the total distance travelled.
View Solution
Knowledge Check
- Question 1 - Select One
A car accelerates from rest at a constant rate for some time after which it decelerates at a constant rate β to come to rest. If the total time elapsed is t, the maximum velocity acquired by the car is given by :
Aαβα+βtBα+βαβtCα2+β2βαDα+β2βα - Question 2 - Select One
A car accelerates from rest at a constant rate for some time after which it decelerates at a constant rate β to come to rest. If the total time elapsed is t, the maximum velocity acquired by the car is given by :
Aab(a+b)tBab(a−b)tCat2(a+b)Dtb2(a+b)
A car accelerates from rest at a constant rate α for some time, after which it decelerates at a constant rate β, to come to rest. If the total time elapsed is t seconds. Then evalute (a) the maximum velocity reached and (b) the total distance travelled.
View SolutionA car accelerates from rest at a constant rate α for some time, after which it decelerates at a constant rate β, to come to rest. If the total time elapsed is t seconds. Then evalute (a) the maximum velocity reached and (b) the total distance travelled.
View SolutionA car accelerates from rest at a constant rate α for some time, after which it decelerates at a constant rate β, to come to rest. If the total time elapsed is t seconds. Then evalute the maximum velocity aquired by the car is
View SolutionA car acceleration form rest at a constant rate α for some time, after which it decelerates at a constant rate β, to come to rest. If the total time elapsed is t evaluate (a) the maximum velocity attained and (b) the total distance travelled.
View Solution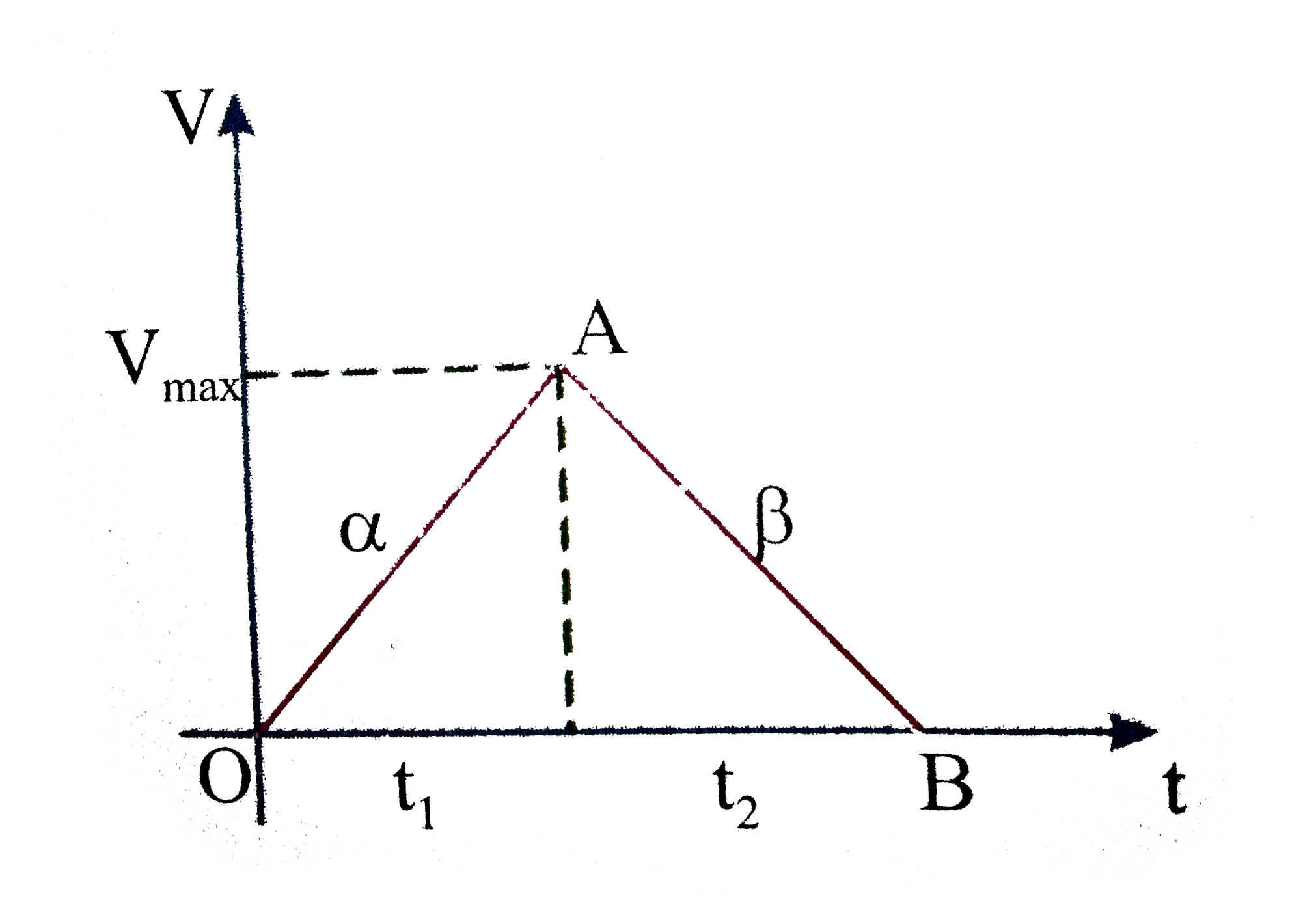
A bus accelerated from rest at a constant rate α for some time, after which it decelerates at a constant rate β to come to rest. If the total time elapsed is t seconds. Then evaluate following parameters from the given graph
(a) the maximum velocity achieved.
(b) the total distance travelled graphically and
(c) Average velocity.View SolutionA car accelerates from rest at a constant rate 'alpha' for some time after which it decelerates at a constant rate β to come to rest. If the total time elapsed is t, the maximum velocity acquired by the car is given by :
View Solution
