In a planet a sucrose solution of coefficient of viscosity is driven at a velocity of through xylem vessels of radius m and length m. The hydrostatic pressure difference across the length of xylem vessels in is
5
8
10
15
The correct Answer is:D
Similar Questions
in a plate, a sucrose solution of coefficient of visocity 1.5×10−3Nsm−2 is driven at a velocity of 10−3ms−1 throught x ylem vessel of radius 2μ m and length5μm. Find the hydrostatic pressure difference across the length of xylem vessel.
View SolutionA liquid solution of coefficient of viscosity 2×10−3Nsm−2 made to drive with velocity of 10−4 m/s along the Xylem vessels of radius 3/4 m and length 7cm. Calculate the pressure difference across the length of Xylem vessel.
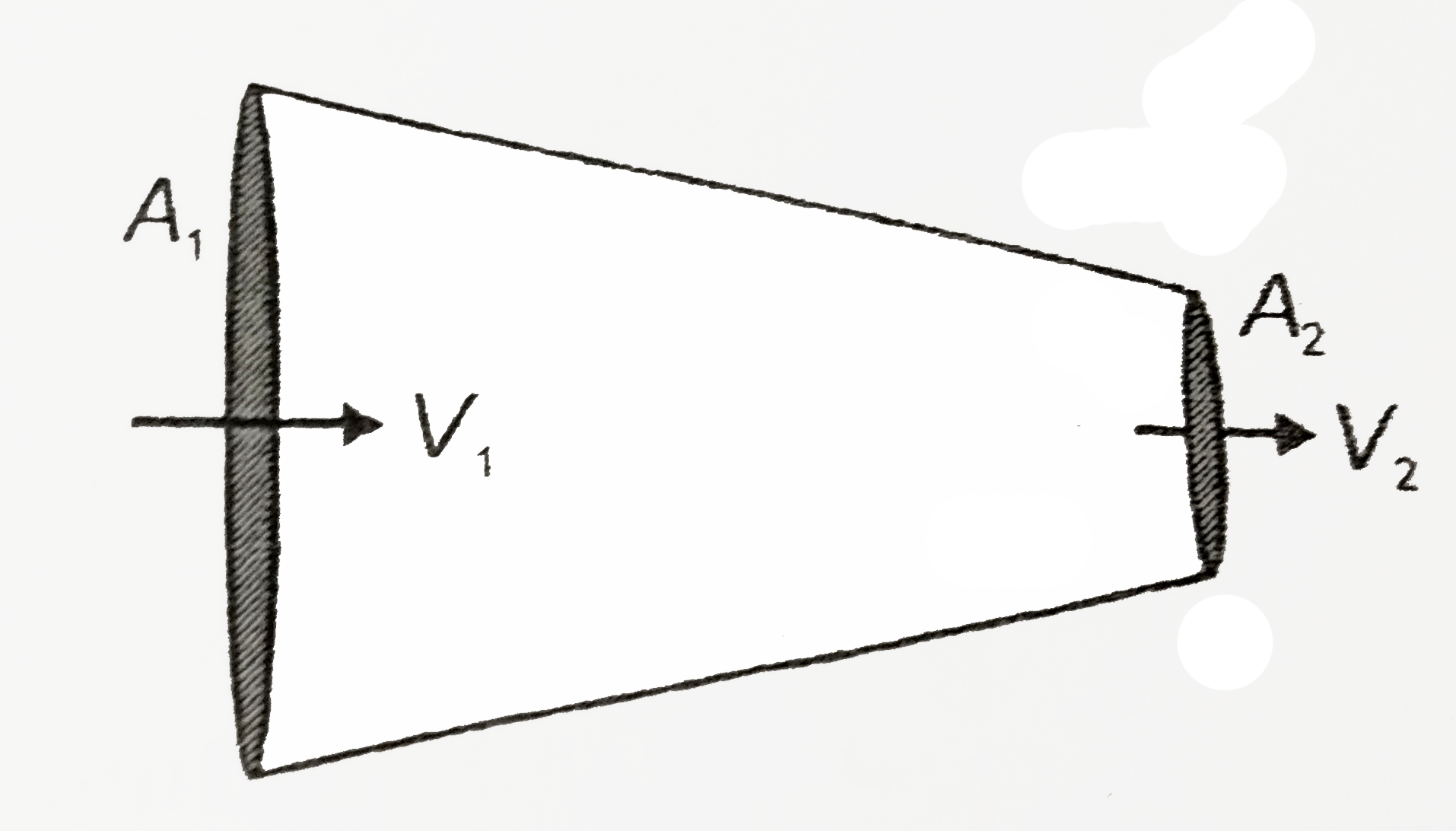
View SolutionCalculate the rate of flow of glycerine of density 1.25×103kgm−3 through the conical section of a pipe, if the radii of its ends are0.1 m and 0.04 m and the pressure drop across its length is 10Nm−2.
View SolutionCalculate the rate of flow of glycerine of density 1.25×103kgm−3 through the conical section of a pipe, if the radii of its ends are0.1 m and 0.04 m and the pressure drop across its length is 10Nm−2.
View SolutionCalculate the rate of flow of glycerine of density 1.20 ×103kg/m3 through the conical section of a pipe. If the radii of its ends are 0.1 m and 0.02 m and the pressure drop across the length is 10 Nm2.
 View Solution
View SolutionCalculate the rate of flow of glycerine of density 1.20 ×103kg/m3 through the conical section of a pipe. If the radii of its ends are 0.1 m and 0.02 m and the pressure drop across the length is 10 Nm2.
 View Solution
View SolutionCalculate the viscous force on a liquid drop of radius 0.3 mm moving with a velocity of 5cms−1 through a medium of viscosity 2×10−5Nm−2s.
View SolutionAn air bubble of radius 10−2 m is rising up at a steady rate of 2×10−3ms−1 through a liquid of density 1.5×103kgm−3 , the coefficient of viscosity neglecting the the density of air, will be (g=10ms−2)
View SolutionAn air bubble of radius 10−2 m is rising up at a steady rate of 2×10−3ms−1 through a liquid of density 1.5×103kgm−3 , the coefficient of viscosity neglecting the the density of air, will be (g=10ms−2)
View SolutionCalculate the rate of flow of glycerine of density 1.25×103kg/m3 through the conical section of a pipe if the radii of its ends are 0.1m and 0.04 m and the pressure drop across its lengths is 10N/m2.
View Solution

