Te magnetic induction field at the centroid or an equilateral triangle of side 'I' nnd canying a current 'i' is
A
2√2μ0iπl
B
9μ0i2πl
C
4μ0iπl
D
3√3μ0iπl
Text Solution
The correct Answer is:B
|
Topper's Solved these Questions
- MOVING CHARGES AND MAGNETISMBOOK - AAKASH SERIESCHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - EXERCISE-III
45 Videos
- MOVING CHARGES AND MAGNETISMBOOK - AAKASH SERIESCHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - EXERCISE-IB
71 Videos
- MOTION IN A STRAIGHT LINE BOOK - AAKASH SERIESCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - very Short answer type question
15 Videos
- NUCLEAR PHYSICSBOOK - AAKASH SERIESCHAPTER - NUCLEAR PHYSICSEXERCISE - ADDITIONAL PRACTICE PRACTICE SHEET (ADVANCED) Integer Type Questions
4 Videos
Similar Questions
Knowledge Check
The magnetic field at the centre of an equilateral triangular loop of side 2L and carrying a current i is
A9μ0i4πLB3√3μ0i4πLC2√3μ0iπLD3μ0i4πLMagnetic field induction at the centre of a square loop of side 'a' carrying current I as shown in figure is
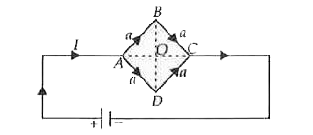 Aμ0I√2πaB2μ0IπaCμ0I2πaDzero
Aμ0I√2πaB2μ0IπaCμ0I2πaDzeroA wire is bent in the form of an equilateral triangle of side 1m and carries a current of 2A.. It is placed in a magnetic field of induction 2.0 T directed into the plane of paper. The direction and magnitude of magnetic force acting on each side of the triangle will be
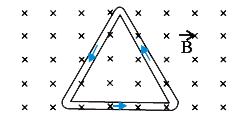 A2N, downwardsB2N, upwardsC4N, upwardsD4N, downwards
A2N, downwardsB2N, upwardsC4N, upwardsD4N, downwards
Magnetic field at center of an equilateral triangle of side length 9cm having current 2A is
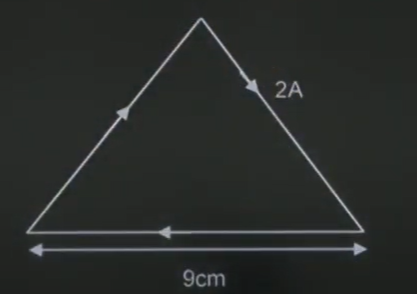
View SolutionFind the magnetic field at the centroid of an equilateral triangle of side0.03 m and a current 2 A flowing through the sides of it.
View SolutionCalculate the magnetic field at the orthocenter of an equilateral triangle, 20 cm on each side. The current through the wire forming the triangle is 10A.
View SolutionFind an expression for the magnetic field induction at the centre of a coil bent in the form of a square of side 2a, carrying current I, figure
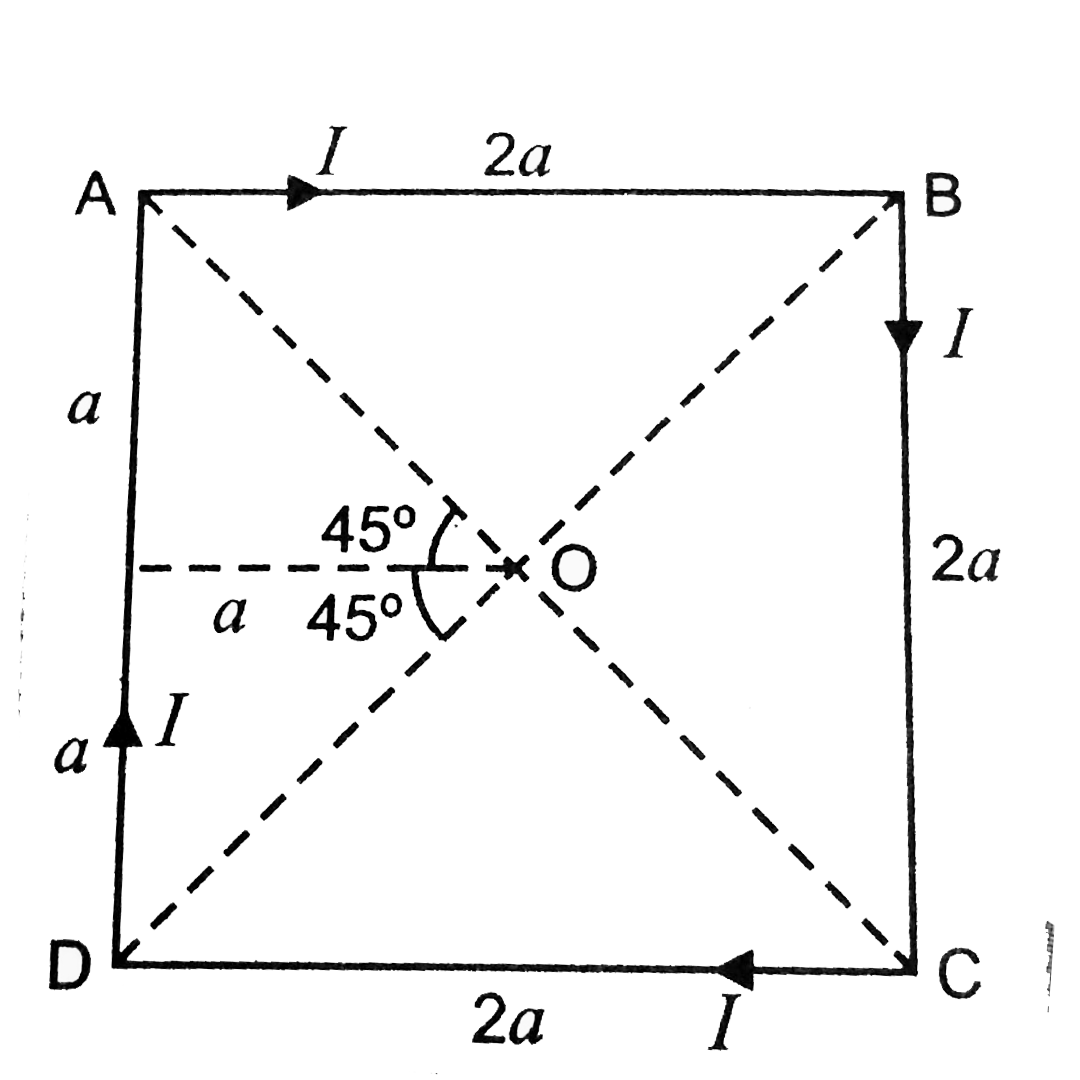
View SolutionMagnetic field induction at the centre O of a square loop of side a carrying current I as shown in figure.
View Solution
