For the given uniform square lamina ABCD, whose centre is O,

A
B
C
D
Text Solution
The correct Answer is:D
Let I be the moment of inertia of the square lamina about an axis through O and perpendicular to its plane . Then by perpendicular axes theorem ,
or
Again by perpendicular axes theorem ,
or
Hence

|
Topper's Solved these Questions
- MOTION OF SYSTEM OF PARTICLES AND RIGID BODY BOOK - MTG GUIDECHAPTER - MOTION OF SYSTEM OF PARTICLES AND RIGID BODY EXERCISE - CHECK YOUR NEET VITALS
14 Videos
- MOTION OF SYSTEM OF PARTICLES AND RIGID BODY BOOK - MTG GUIDECHAPTER - MOTION OF SYSTEM OF PARTICLES AND RIGID BODY EXERCISE - AIPMT / NEET (MCQs)
37 Videos
- MOTION OF SYSTEM OF PARTICLES AND RIGID BODY BOOK - MTG GUIDECHAPTER - MOTION OF SYSTEM OF PARTICLES AND RIGID BODY EXERCISE - AIPMT / NEET (MCQs)
37 Videos
- LAWS OF MOTIONBOOK - MTG GUIDECHAPTER - LAWS OF MOTIONEXERCISE - AIPMT /NEET (MCQ)
24 Videos
- OSCILLATIONS AND WAVES BOOK - MTG GUIDECHAPTER - OSCILLATIONS AND WAVES EXERCISE - AIPMT / NEET MCQs
43 Videos
Similar Questions
Knowledge Check
In the adjoining diagram ABCD is a square with side 'a' cm. In the diagram the area of the larger circle with centre 'O' is equal to the sum of the areas of all the rest four circles with equal radii, whose centres are P,Q,R and S . What is the ratio between the side of square and radius of a smaller circle ?
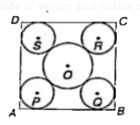 A(2√2+3)B(2+3√2)C(4+3√2)Dcan't be determined
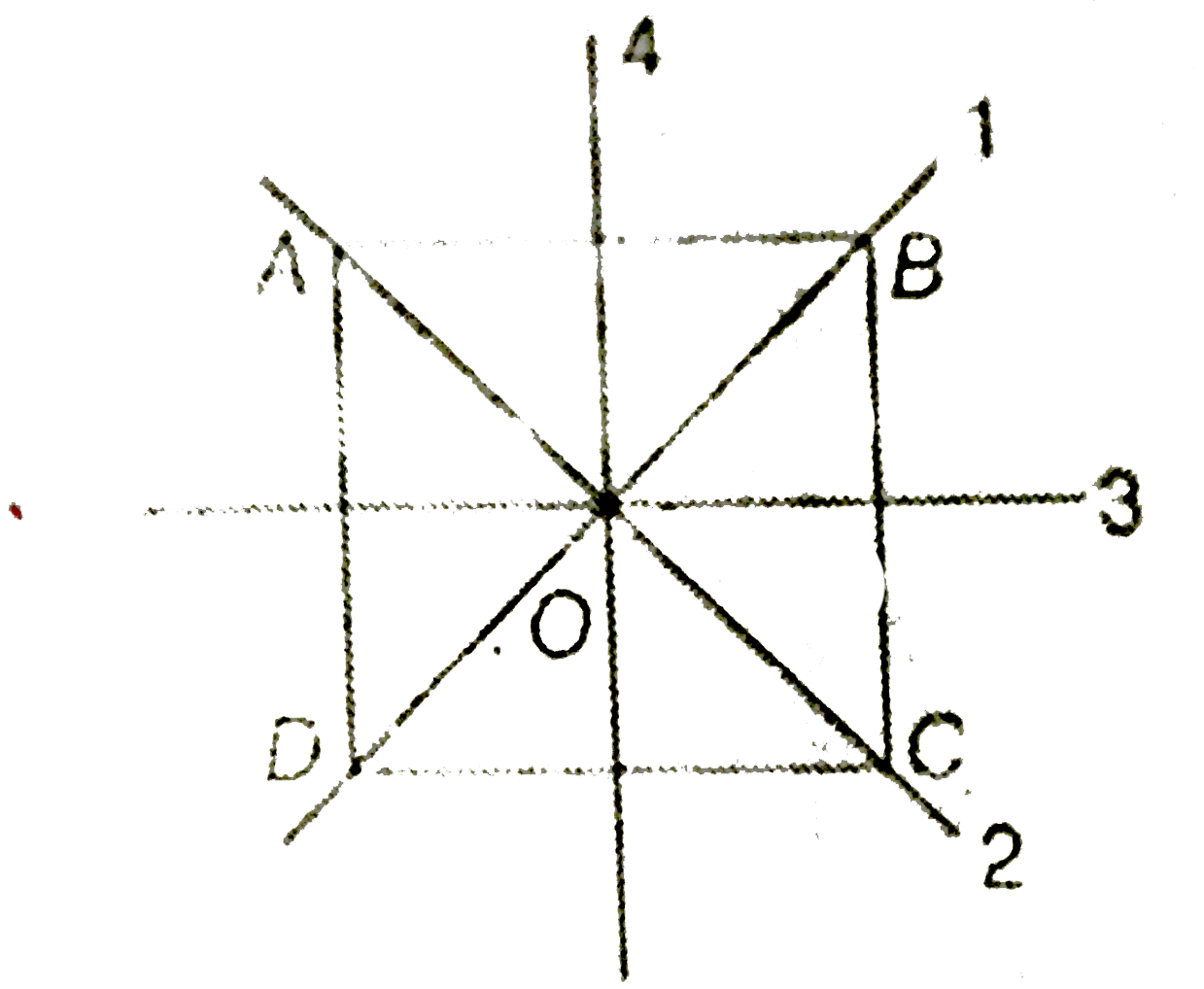
A(2√2+3)B(2+3√2)C(4+3√2)Dcan't be determinedThe moment of inertia of a thin square plate ABCD, as shown A in the figure, of uniform thickness about an axis passing through the centre O and perpendicular to the plane of
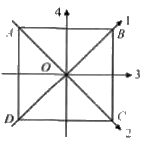 Al1+l2Bl3+l4Cl1+l3Dl1+l2+l3+l4 ltbRgt where l1,l2,l3 and l4 are respectively the moments of inertia about axis 1, 2, 3 and 4 which are in the plane of the plate.
Al1+l2Bl3+l4Cl1+l3Dl1+l2+l3+l4 ltbRgt where l1,l2,l3 and l4 are respectively the moments of inertia about axis 1, 2, 3 and 4 which are in the plane of the plate.The x, y coordinates of the centre of mass of a uniform L-shaped lamina of mass 3 kg is
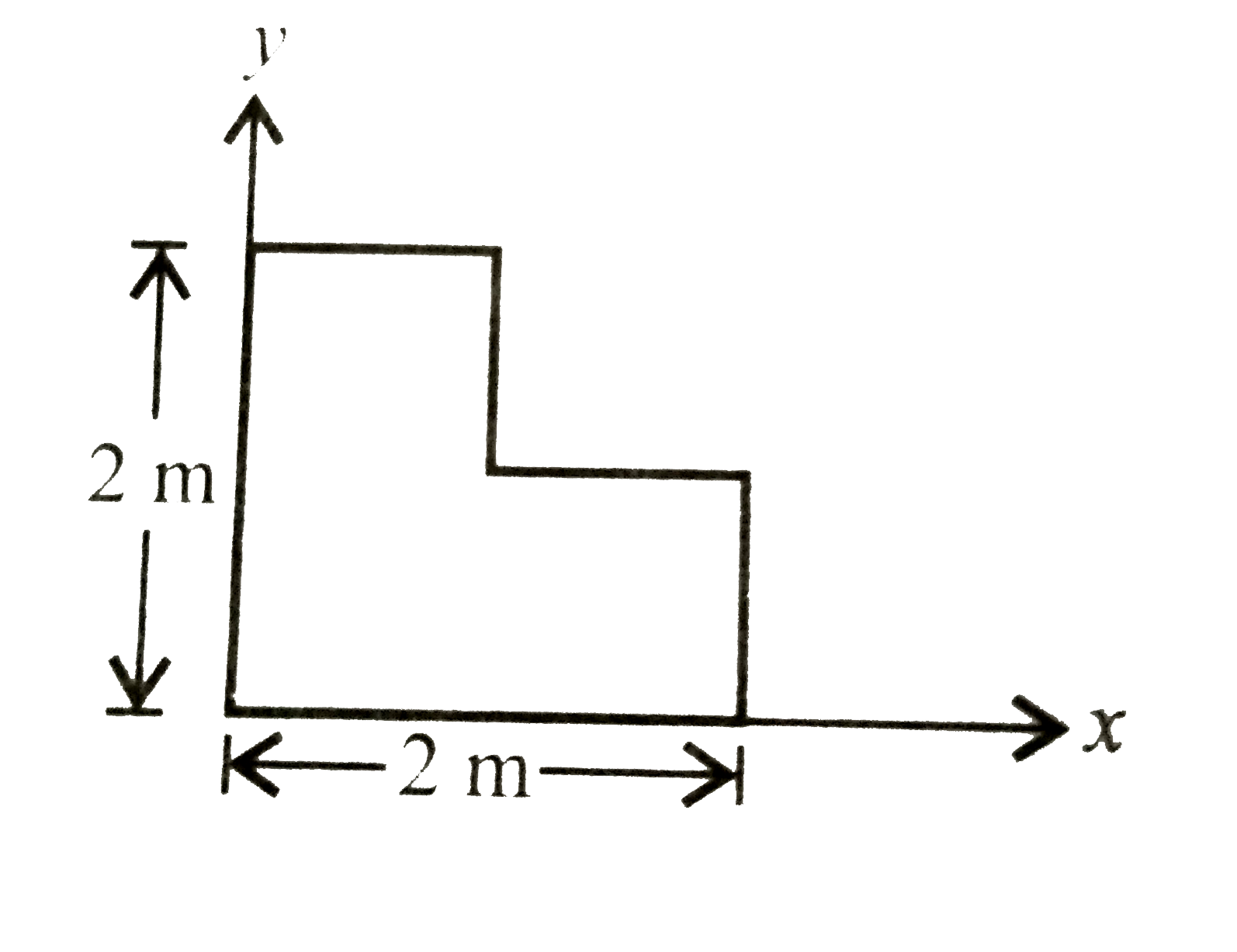 A(5/6 m, 5/6 m)B(1 m, 1 m)C(6/5 m, 6/5 m)D(2 m, 2m)
A(5/6 m, 5/6 m)B(1 m, 1 m)C(6/5 m, 6/5 m)D(2 m, 2m)
Find out the position of centre of mass of the uniform lamina as shown in figure.from center of cavity

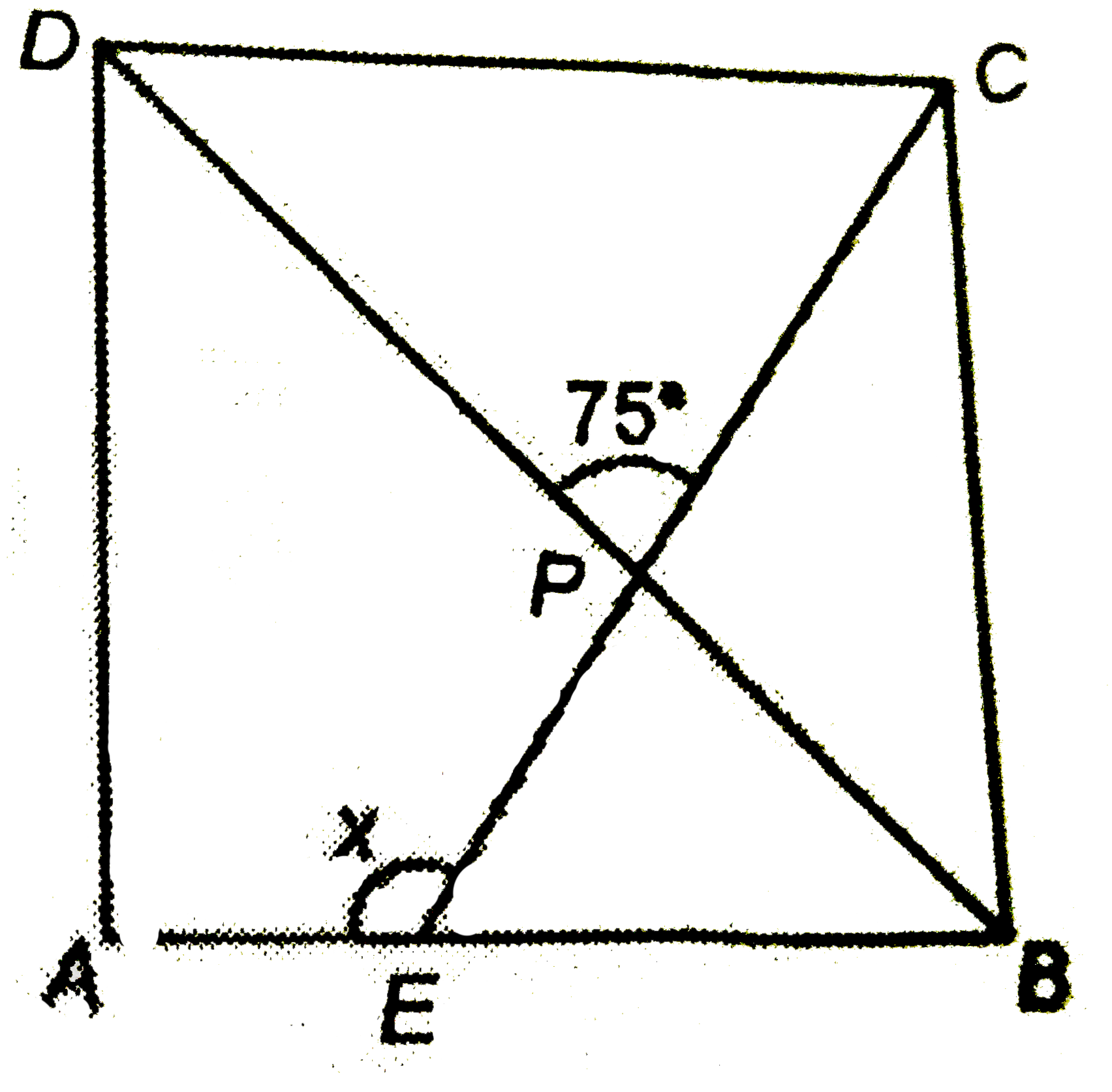
View SolutionIn the given figure, ABCD is a square. Find x.
View SolutionThe x, y coordinates of the centre of mass of a uniform L-shaped lamina of mass 3 kg is

View SolutionIn the given figure, ABCD is a square. Find x.

View SolutionThe moment of inertia of a thin square plate ABCD of uniform thickness about an axis passing through the centre O and perpendicular to plate is
View Solution