A sphere of mass m and radius r rolls on a horizontal plane without slipping with the speed u . Now if it rolls up vertically , the maximum height it would attain will be
3u2/4g
5u2/2g
7u2/10g
u2/2g
The correct Answer is:C
The rolling sphere has rotational as well as translational kinetic energy .
∴ Kinetic energy = 12mu2+12Iω2
=12mu2+12(25mr2)ω2=12mu2+mu25=710mu2 .
Potential energy = kineti energy
∴mgh=710mu2orh=7u210g
Topper's Solved these Questions
- BOOK - MTG GUIDECHAPTER - MOTION OF SYSTEM OF PARTICLES AND RIGID BODY EXERCISE - CHECK YOUR NEET VITALS14videos
MOTION OF SYSTEM OF PARTICLES AND RIGID BODY
- BOOK - MTG GUIDECHAPTER - MOTION OF SYSTEM OF PARTICLES AND RIGID BODY EXERCISE - AIPMT / NEET (MCQs)37videos
MOTION OF SYSTEM OF PARTICLES AND RIGID BODY
- BOOK - MTG GUIDECHAPTER - MOTION OF SYSTEM OF PARTICLES AND RIGID BODY EXERCISE - AIPMT / NEET (MCQs)37videos
MOTION OF SYSTEM OF PARTICLES AND RIGID BODY
- BOOK - MTG GUIDECHAPTER - LAWS OF MOTIONEXERCISE - AIPMT /NEET (MCQ)24videos
LAWS OF MOTION
- BOOK - MTG GUIDECHAPTER - OSCILLATIONS AND WAVES EXERCISE - AIPMT / NEET MCQs43videos
OSCILLATIONS AND WAVES
Similar Questions
A sphere of mass m and radius r rolls on a horizontal plane without slipping with a speed u. Now it rolls up vertically, then maximum height it would be attain will be
View SolutionA mass m of radius r is rolling horizontally without any slip with a linear speed v. It then rolls up to a height given by 34v2g
View Solution
Knowledge Check
- Question 1 - Select One
A sphere of mass m and radius r rolls on a horizontal plane without slipping with a speed u. Now it rolls up vertically, then maximum height it would be attain will be
A3u24gB5u22gC7u210gDu22g - Question 2 - Select One
A body of mass M and radius R is rolling horizontally without slipping with speed v . It then rolls up a hill to a maximum height h . If h=5v26g , what is the M.I of the body ?
AMR22B23MR2C34Mr2D25MR2 - Question 3 - Select One
An object of radius R and mass M is rolling horizontally without slipping with speed v . It then rolls up the hill to a maximum height h=3v24g . The moment of inertia of the object is ( g = acceleration due to gravity)
Asolid sphereBhollow sphereCdiscDring
A ring, disc, spherical shell and solid sphere of same mass and radius are rolling on a horizontal surface without slipping with same velocity. If they move up an inclined plane, which can reach to a maximum height on the inclined plane?
View SolutionA sphere of moment of inertia 'I' and mass 'm' rolls down on an inclined plane without slipping its K.E. of rolling is
View SolutionA solid cylinder of mass M and radius R rolls down an inclined plane without slipping. THE speed of its centre of mass when it reaches the bottom is
View SolutionA disc of radius R and mass M is rolling horizontally without slipping with speed with speed v. It then moves up an incline as shown in figure. The maximum height upto which it can reach is.
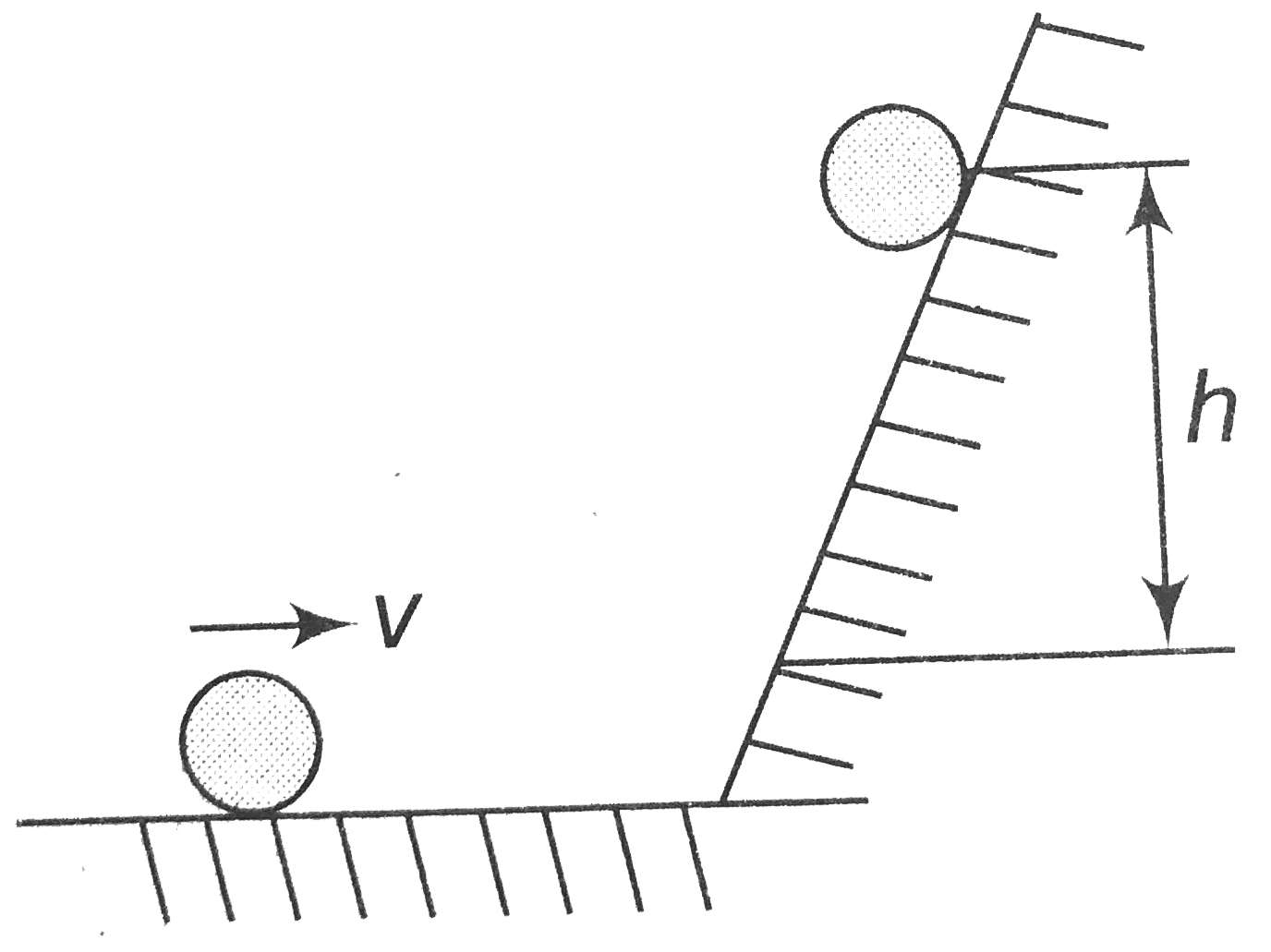 .View Solution
.View SolutionA mass m of radius r is rolling horizontally without any slip with a linear speed v. It then rolls up to a height given by 34v2g
View Solution
