A block of mass M is held against a rough vertical wall by pressing it with a finger . If the coefficient of friction between the block and the wall is μ and the acceleration due to gravity is g , calculate the minimum force required to be applied by the finger to hold the block against the wall.
μMg
Mg
Mgμ
2μMg
The correct Answer is:C
To solve the problem of finding the minimum force required to hold a block of mass M against a rough vertical wall, we can follow these steps:
Step 1: Identify the forces acting on the block
- The block experiences several forces:
- The weight of the block W=Mg acting downwards (where g is the acceleration due to gravity).
- A normal force N acting horizontally from the wall on the block.
- A frictional force f acting upwards, which opposes the weight of the block.
Step 2: Understand the role of friction
- The frictional force f can be expressed in terms of the normal force N and the coefficient of friction μ:
f=μN
- Since the block is not moving, the frictional force must balance the weight of the block:
f=Mg
Step 3: Set up the equation
- From the previous step, we can equate the frictional force to the weight of the block:
μN=Mg
Step 4: Solve for the normal force N
- Rearranging the equation gives us:
N=Mgμ
Step 5: Relate the normal force to the force applied by the finger
- The force F applied by the finger is equal to the normal force N exerted by the wall on the block:
F=N
- Therefore, substituting for N:
F=Mgμ
Final Answer
The minimum force required to be applied by the finger to hold the block against the wall is:
F=Mgμ
- The block experiences several forces:
- The weight of the block W=Mg acting downwards (where g is the acceleration due to gravity).
- A normal force N acting horizontally from the wall on the block.
- A frictional force f acting upwards, which opposes the weight of the block.
- The frictional force f can be expressed in terms of the normal force N and the coefficient of friction μ:
f=μN
- Since the block is not moving, the frictional force must balance the weight of the block:
f=Mg
- From the previous step, we can equate the frictional force to the weight of the block:
μN=Mg
- Rearranging the equation gives us:
N=Mgμ
- The force F applied by the finger is equal to the normal force N exerted by the wall on the block:
F=N
- Therefore, substituting for N:
F=Mgμ
The minimum force required to be applied by the finger to hold the block against the wall is:
F=Mgμ
Topper's Solved these Questions
- LAWS OF MOTIONBOOK - NCERT FINGERTPS ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - HOTS
8 Videos
- LAWS OF MOTIONBOOK - NCERT FINGERTPS ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Exemplar Problems
9 Videos
- KINETIC THEORYBOOK - NCERT FINGERTPS ENGLISHCHAPTER - KINETIC THEORYEXERCISE - Assertion And Reason
10 Videos
- MECHANICAL PROPERTIES OF FLUIDSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - NCERT Exemplar
5 Videos
Similar Questions
A body is pushed against vertical wall as shown. If the coefficient of friction between the body and the wall is 0.5, the magnitude of acceleration of the block is 10 K. Where K is
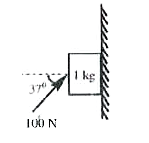
View SolutionA block of mass 0.1 is held against a wall applying a horizontal force of 5N on block. If the coefficient of friction between the block and the wall is 0.5, the magnitude of the frictional force acting on the block is:
View Solution
Knowledge Check
A girl press her physics text book against a rough vertical wall with her hand. The direction of the frictional force on the book exerted by the wall is
AdownwardsBupwardsCout from the wallDinto the wall
A block of mass 1 kg is pushed against a rough vertical wall of μ=0.1
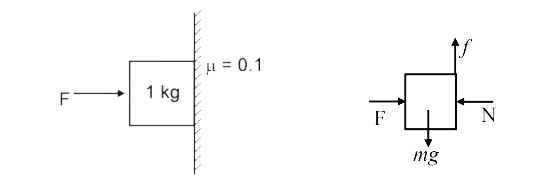
For F = 150, 50 or 100 N the force of friction on the blocks is respectively :
View SolutionA block of mass 1 kg is held at rest against a rough vertical surface by pushing by a force F horizontally. The coefficient of friction is 0.5. When

View SolutionA block of mass 1 kg is pressed against a wall by applying a horizontal force of 10N on the block. If the coefficient of friction between the block and the wall is 0.5, magnitude of the frictional force acting on the block is
View SolutionA block of mass 0.1 kg is held against a wall applying a horizontal force of 5 N on the block. If the coefficient of friction between the block and the wall is 0.5, the magnitude of the frictional force acting on the block is :
View SolutionA block of mass m sides down an inclined right angled trough .If the coefficient of friction between block and the trough is μk acceleration of the block down the plane is
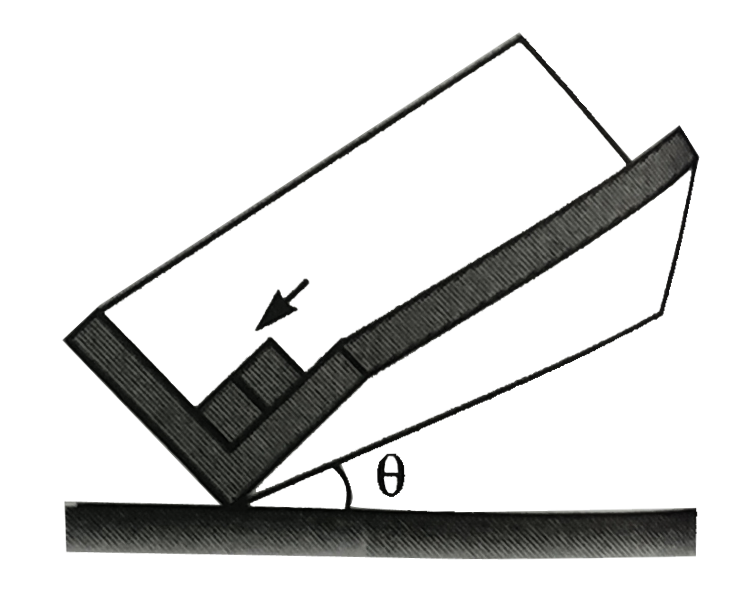
View Solution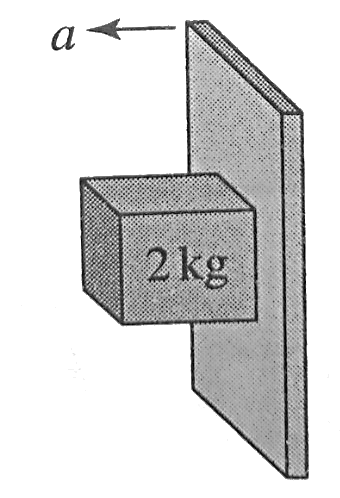
A rough vertical board has an acceleration a so that a 2 kg block pressing against it dows not fall. The coefficient of friction between the block and the board should be
View Solution
A rough vertical board has an acceleration a so that a 2 kg block pressing against it dows not fall. The coefficient of friction between the block and the board should be
View Solution