Two identical balls A and B are released from the position shown in Fig. They collide elastically with each other on the horizontal portion. The ratio of heights attained by A and B after collision is (neglect friction)
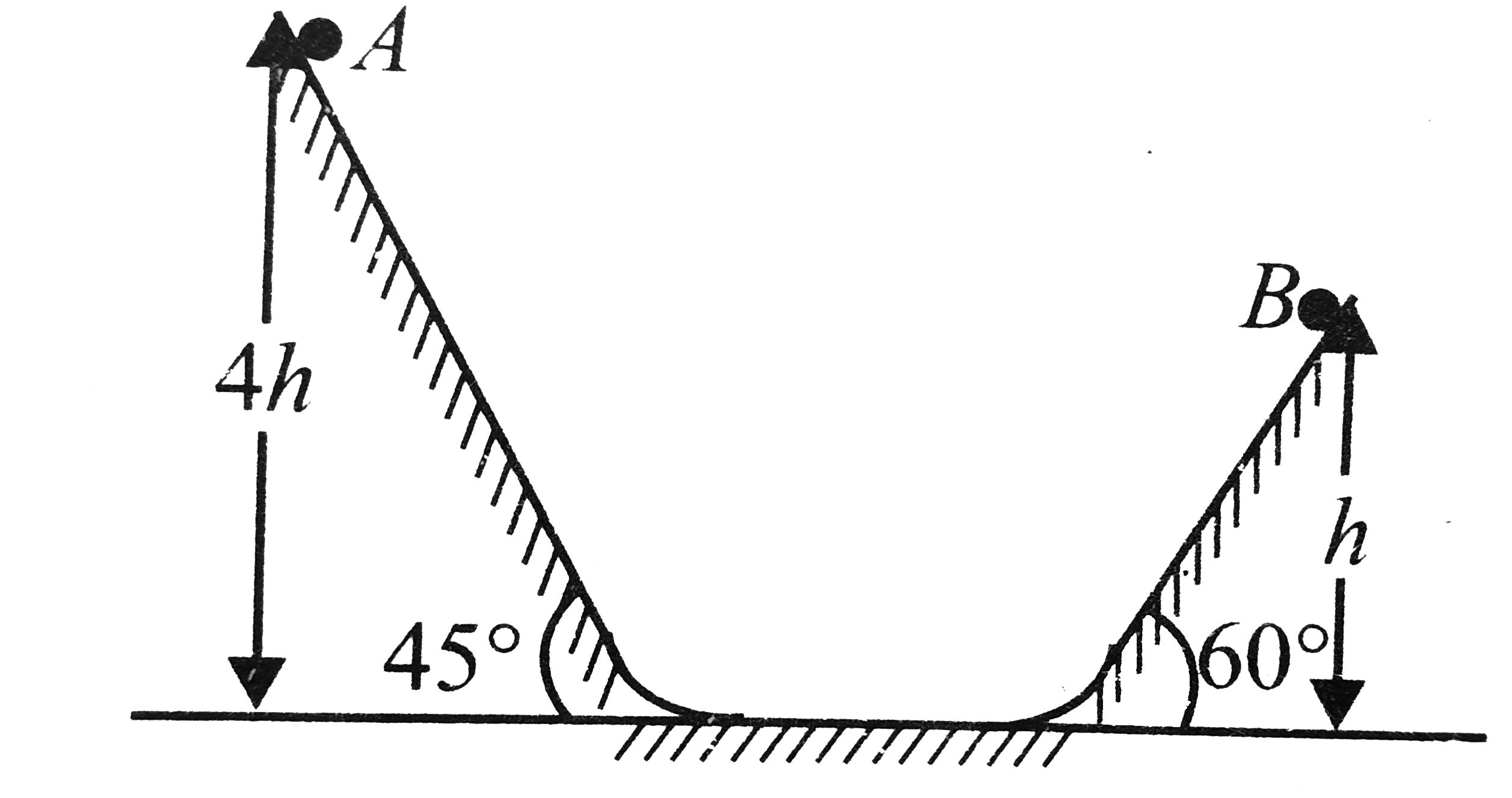
1:4
2:1
4:13
2:11
The correct Answer is:C
After collision balls exchange their velocities
i.e., vA=√2ghandvB=√2g(4h)=2√2gh
Height attained by A will be hA=v2A2g=h
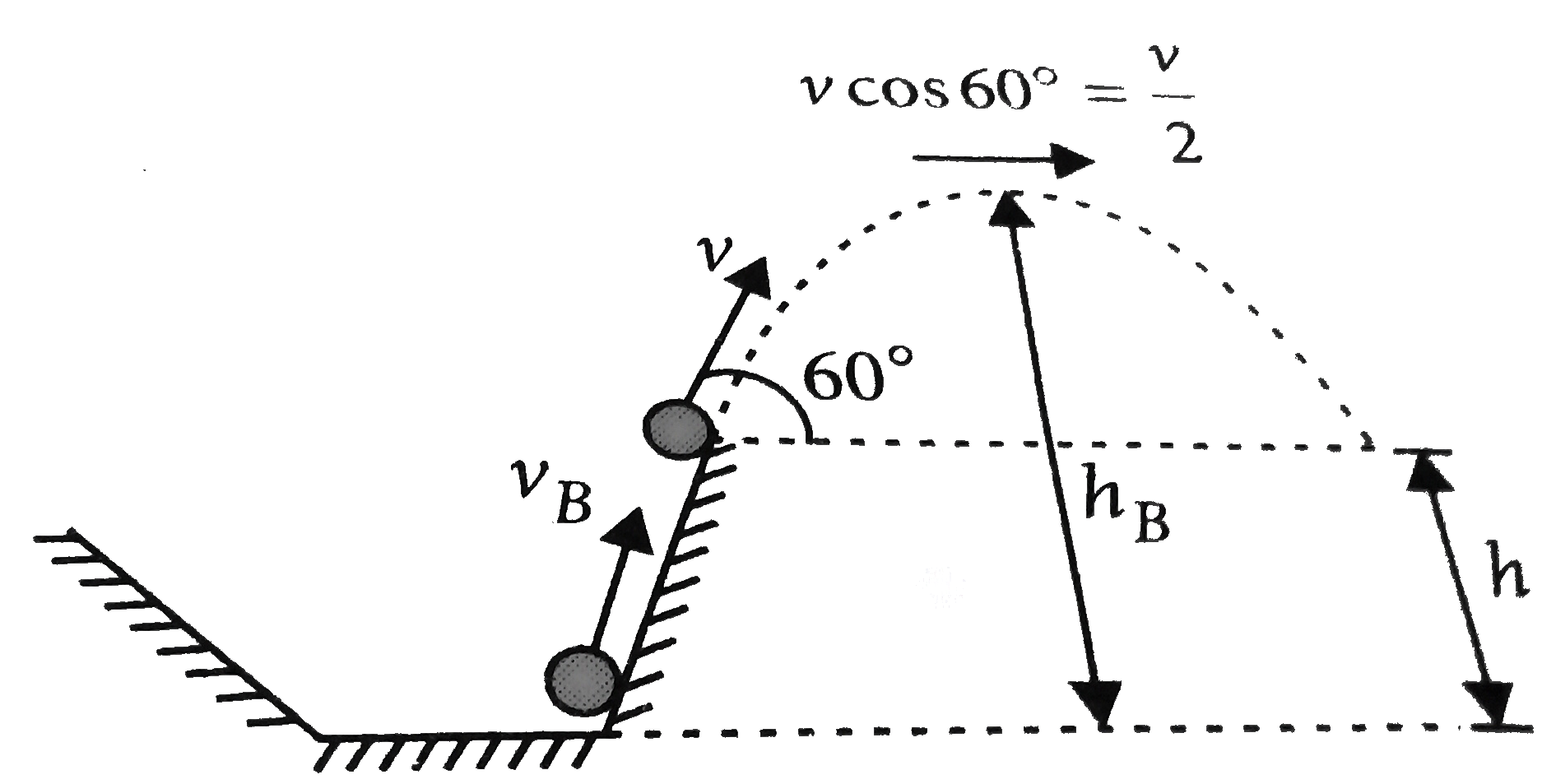
But path of B will be first straight line and then parabolic as shown in figure.
Using energy conservation for ball B.
12mv2B=12mv2+mgh orv=√6gh
∴hB=h+v2sin260∘2g=h+9h4=13h4
Hence, hAhB=413
Topper's Solved these Questions
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - WORK , ENERGY AND POWEREXERCISE - NCERT18videos
WORK , ENERGY AND POWER
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - WORK , ENERGY AND POWEREXERCISE - ASSERTION & REASON15videos
WORK , ENERGY AND POWER
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - WAVESEXERCISE - Assertion And Reason15videos
WAVES
Similar Questions
A small ball of mass m moving with speed v collides elastically with a simple pendulum with bob of mass m at rest. The maximum height attained by the bob after collision is
View SolutionThree solid spheres each of mass m and radius R are released from the position shown in Fig. What is the speed of any one sphere at the time of collision?
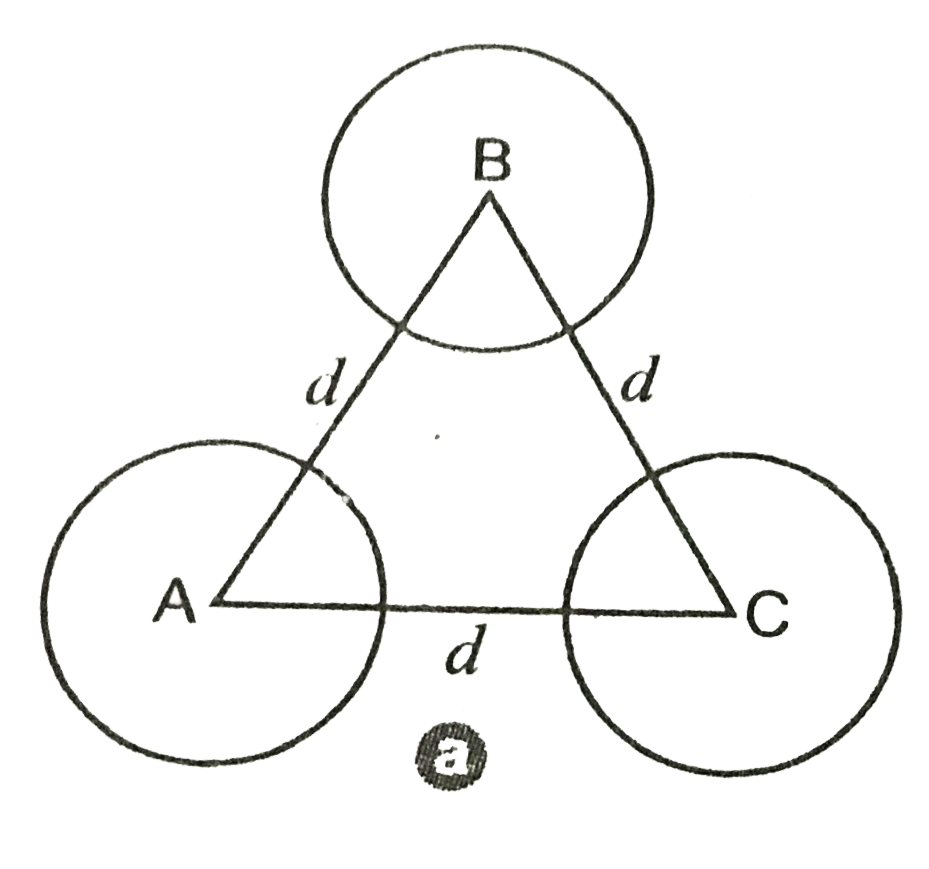 View Solution
View SolutionA ball is released from point A as shown in figure. The ball leaves the track at B. All surfaces are smooth.

If track makes an angle 30∘ with horizontal at B, then maximum height attained by ball will beView SolutionIn the arrangement shown in Fig. the ball and the block have the same mass m=1kg each, θ=60∘ and length l=2.50m. Coefficient of friction between the block and the floor is 0.5. When the ball is released from the position shown in Fig. it collides with the block and the block stops after moving a distance 2.50m.
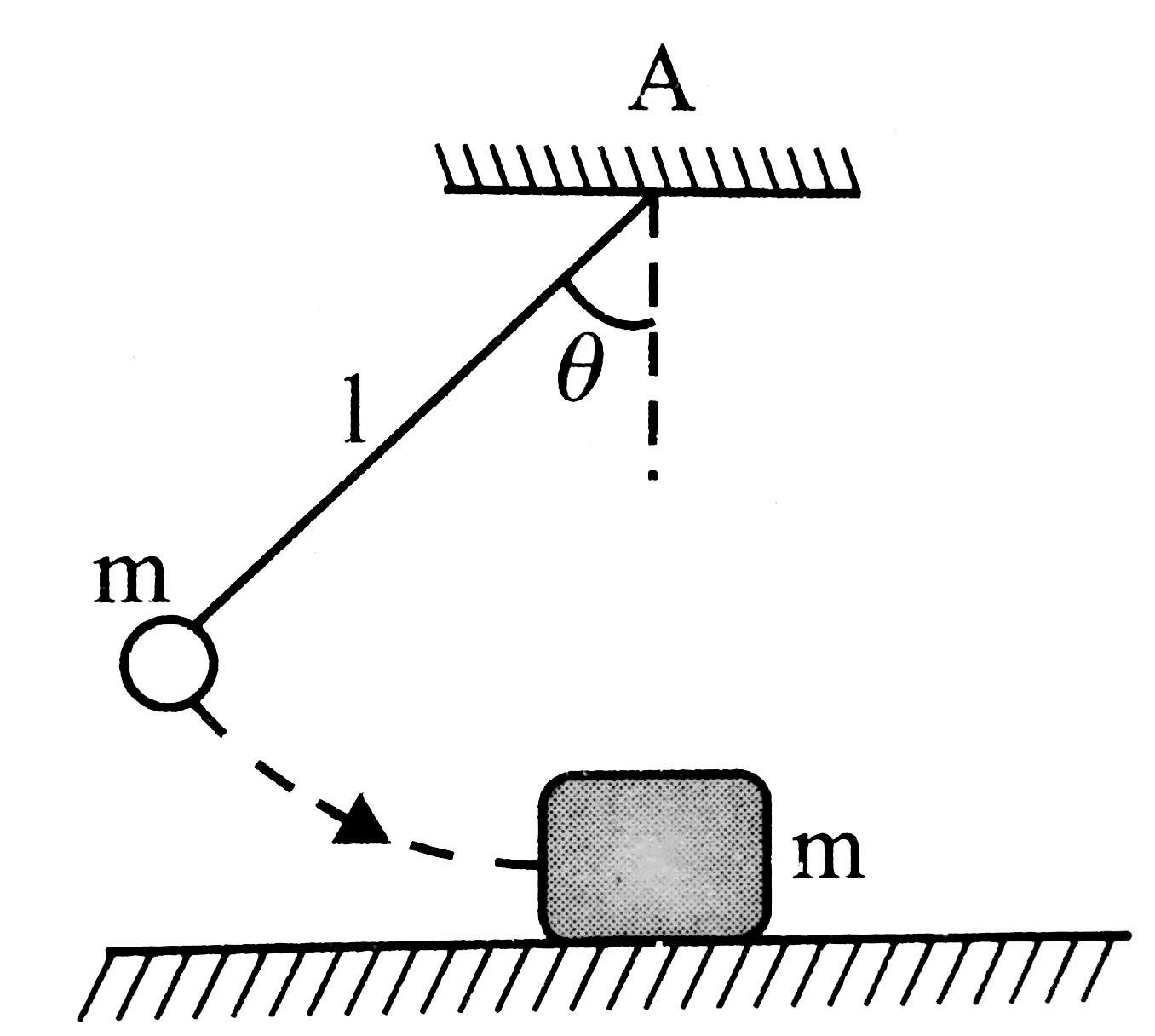
The velocity of block just after collision isView SolutionIn the arrangement shown in Fig. the ball and the block have the same mass m=1kg each, θ=60∘ and length l=2.50m. Coefficient of friction between the block and the floor is 0.5. When the ball is released from the position shown in Fig. it collides with the block and the block stops after moving a distance 2.50m.
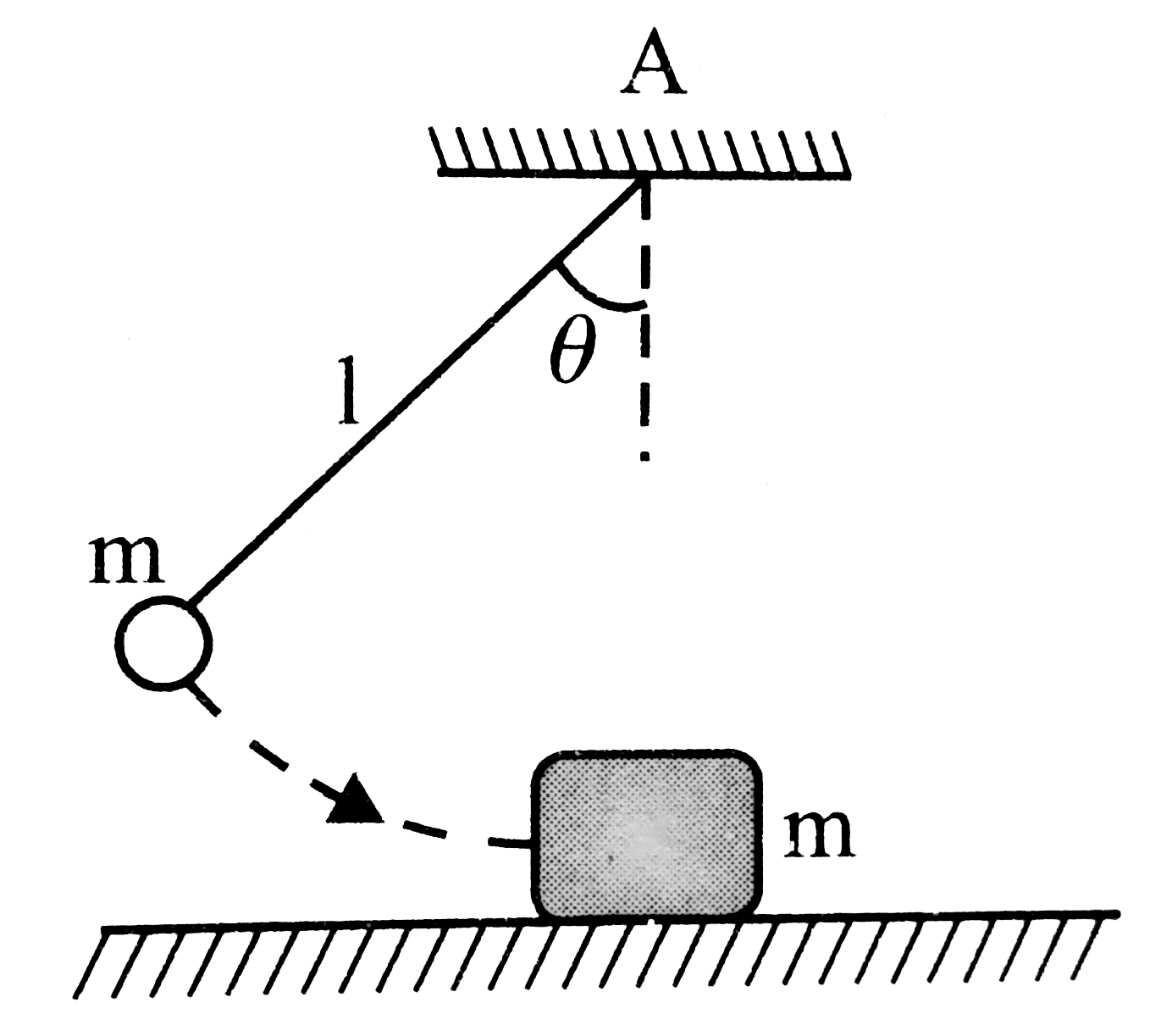
The coefficient of restitution for collision between the ball and the block isView SolutionTwo identical balls each moving with speed v at right angle to each other collide perfectly inelastically. Their speed after collision is
View SolutionA ball of mass 'm is released from the top of a smooth movable wedge of mass 'm'. When the ball collides with the floor, velocity is 'v'. Then the maximum height attained by the ball after an elastic collision with the floor is : (Nelect any edge at the lower end of the wedge)
 View Solution
View SolutionA ball of mass m moving with velocity v collides head on elastically with another identical ball moving with velocity - V. After collision
View SolutionTwo identical balls AandB having velocity of 0.5m/sand−0.3m/s respectively collide elastically in one dimension. The velocities of BandA after the collision respectively will be
View SolutionTwo identiacal balls A and B having velocities of 0.5 m/s and 0.3 m/s respectively collide elastically in one dimension. The velocities of B and A after the collision respectively will be
View Solution

