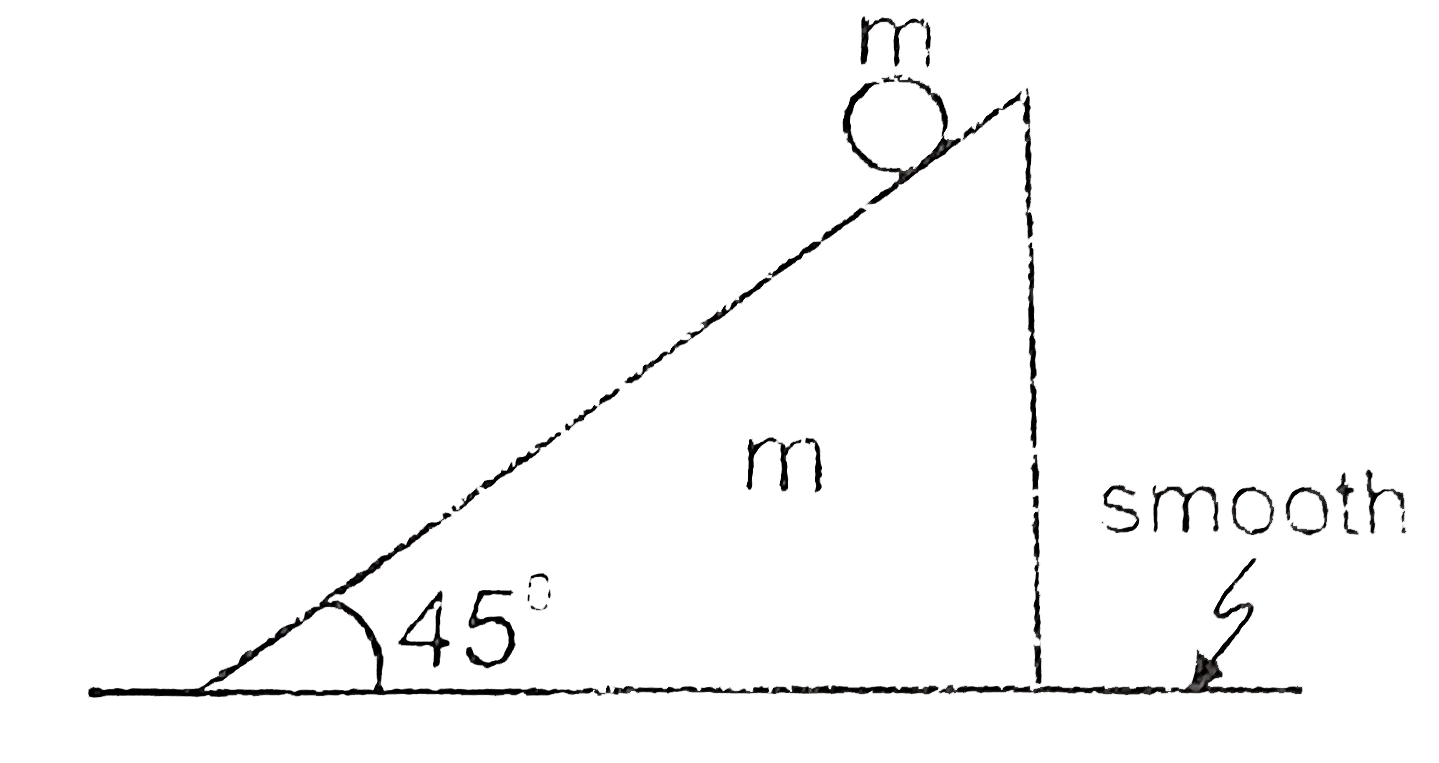
A ball of mass 'm is released from the top of a smooth movable wedge of mass 'm'. When the ball collides with the floor, velocity is 'v'. Then the maximum height attained by the ball after an elastic collision with the floor is : (Nelect any edge at the lower end of the wedge)
2v2g
v24g
4v2g
v22g
The correct Answer is:A
Let 'u' be the velocity of the ball .w.r.t. wedge when it reaches the floor. Then, the x-component of velocity of the ball .w.r.t. ground will be (v−u√2) towards right.
By momentum conservation : 0=m(v)+m(v−u√2)⇒u=2√2v
Therefore, the y-component of velocity of the ball after the elastic collision with the floor will be ucos45∘
=u√2=2v (upward)
∴ Maximum height =(2v)22g=2v2g
Topper's Solved these Questions
- DAILY PRACTICE PROBLEMBOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.64
20 Videos
- DAILY PRACTICE PROBLEMBOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.65
9 Videos
- DAILY PRACTICE PROBLEMBOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.62
9 Videos
- CURRENT ELECTRICITYBOOK - RESONANCE ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - High Level Problems (HIP)
19 Videos
- ELECTRO MAGNETIC WAVESBOOK - RESONANCE ENGLISHCHAPTER - ELECTRO MAGNETIC WAVESEXERCISE - Exercise 3
27 Videos
Similar Questions
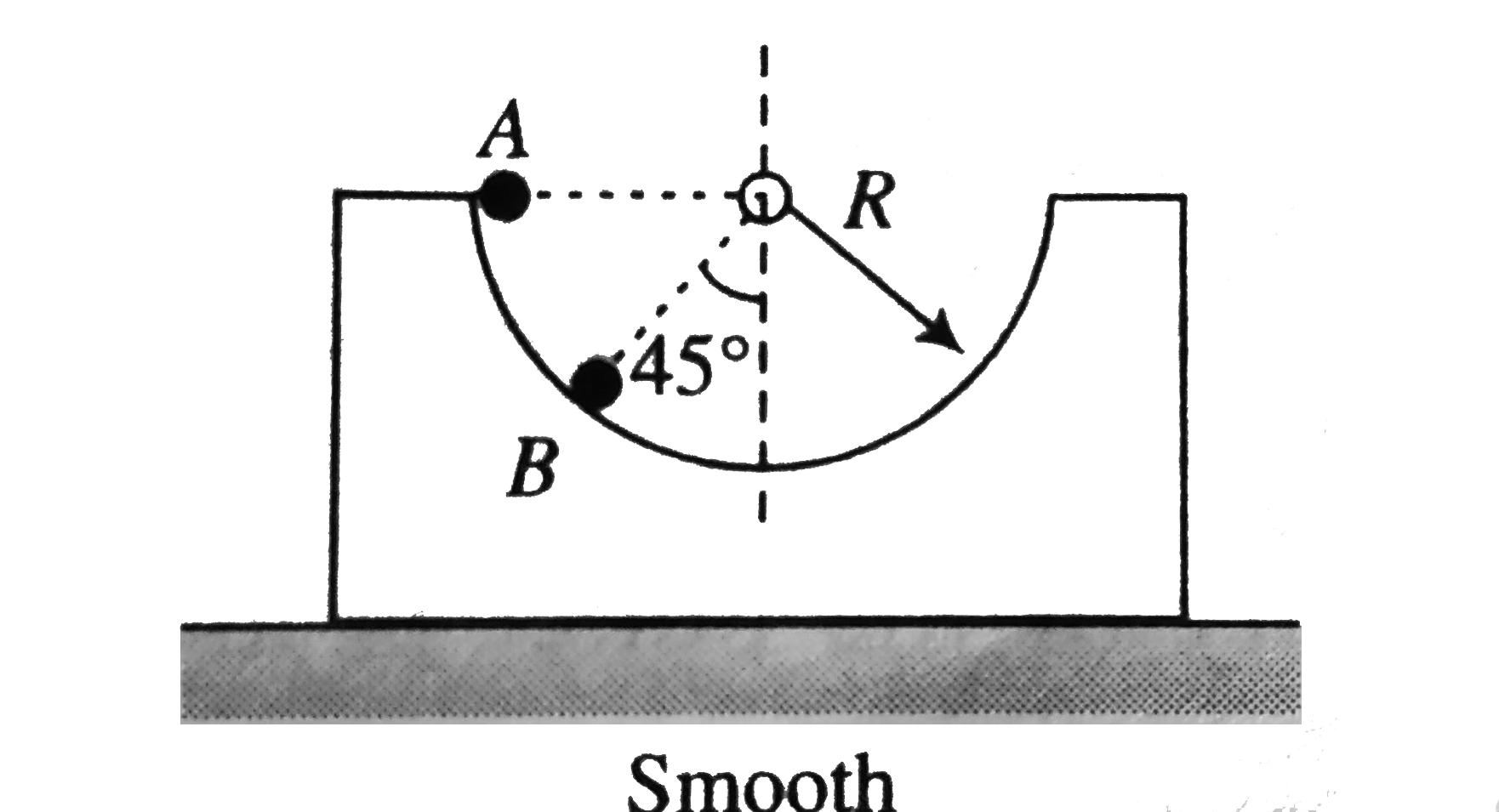
A ball of mass m is released from A inside a smooth wedge of mass m as shown in figure. What is the speed of the wedge when the ball reaches point B?
View SolutionA small ball of mass m moving with speed v collides elastically with a simple pendulum with bob of mass m at rest. The maximum height attained by the bob after collision is
View SolutionA small ball of mass m is thrown upward with velocity u from the ground. The ball experiences a resistive force mkv2 where v is its speed. The maximum height attained by the ball is :
View SolutionA small ball ( uniform solid sphere ) of mass m is released from the top of a wedge of the same mass m. The wedge is free to move on a smooth horizontal surface. The ball rolld without sliding on the wedge. The required height of the wedge are mentioned in the figure.
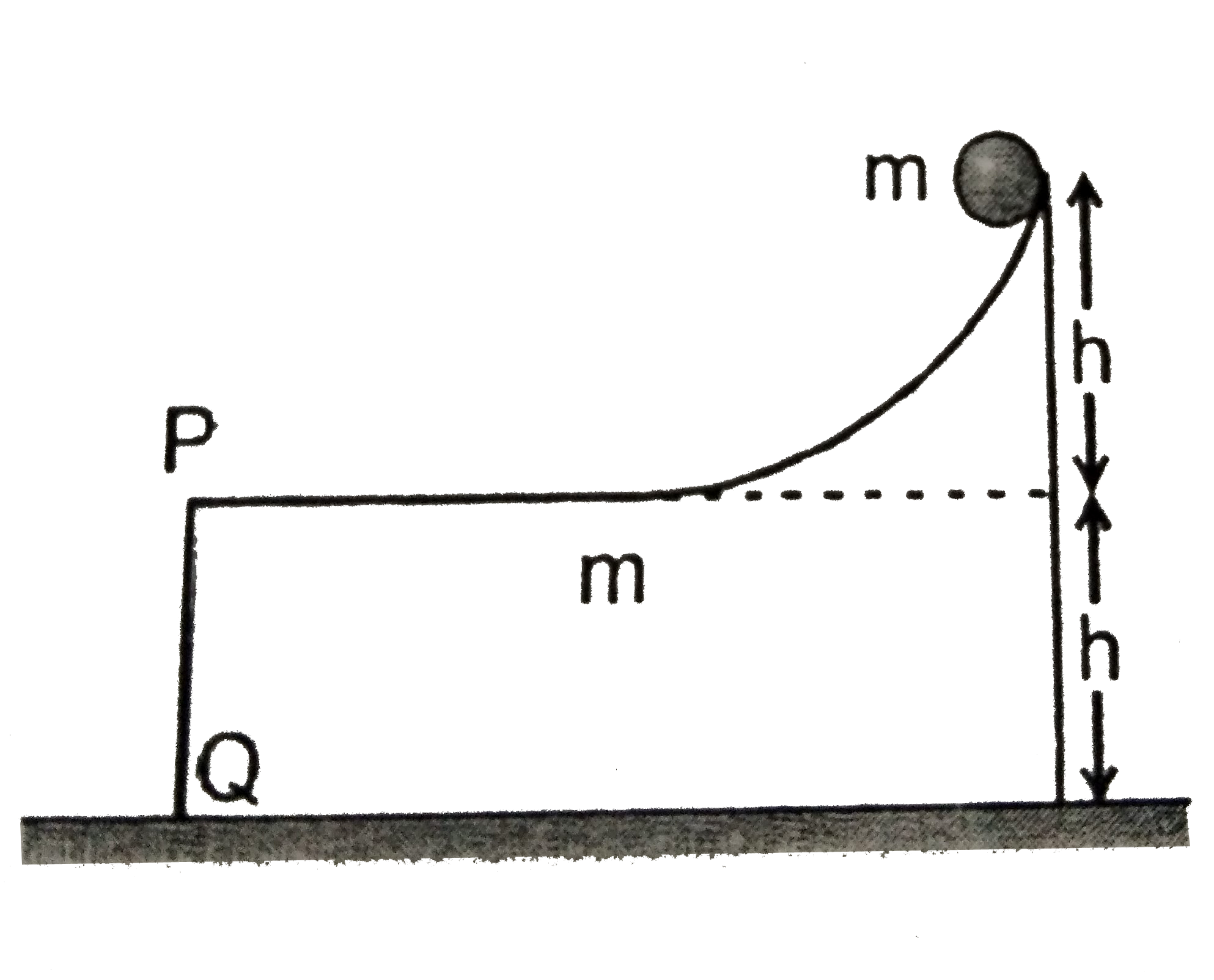
The speed of the wedge when the ball is just going to leave the wedge at point 'P' of the wedge is
View SolutionA small ball ( uniform solid sphere ) of mass m is released from the top of a wedge of the same mass m. The wedge is free to move on a smooth horizontal surface. The ball rolld without sliding on the wedge. The required height of the wedge are mentioned in the figure.
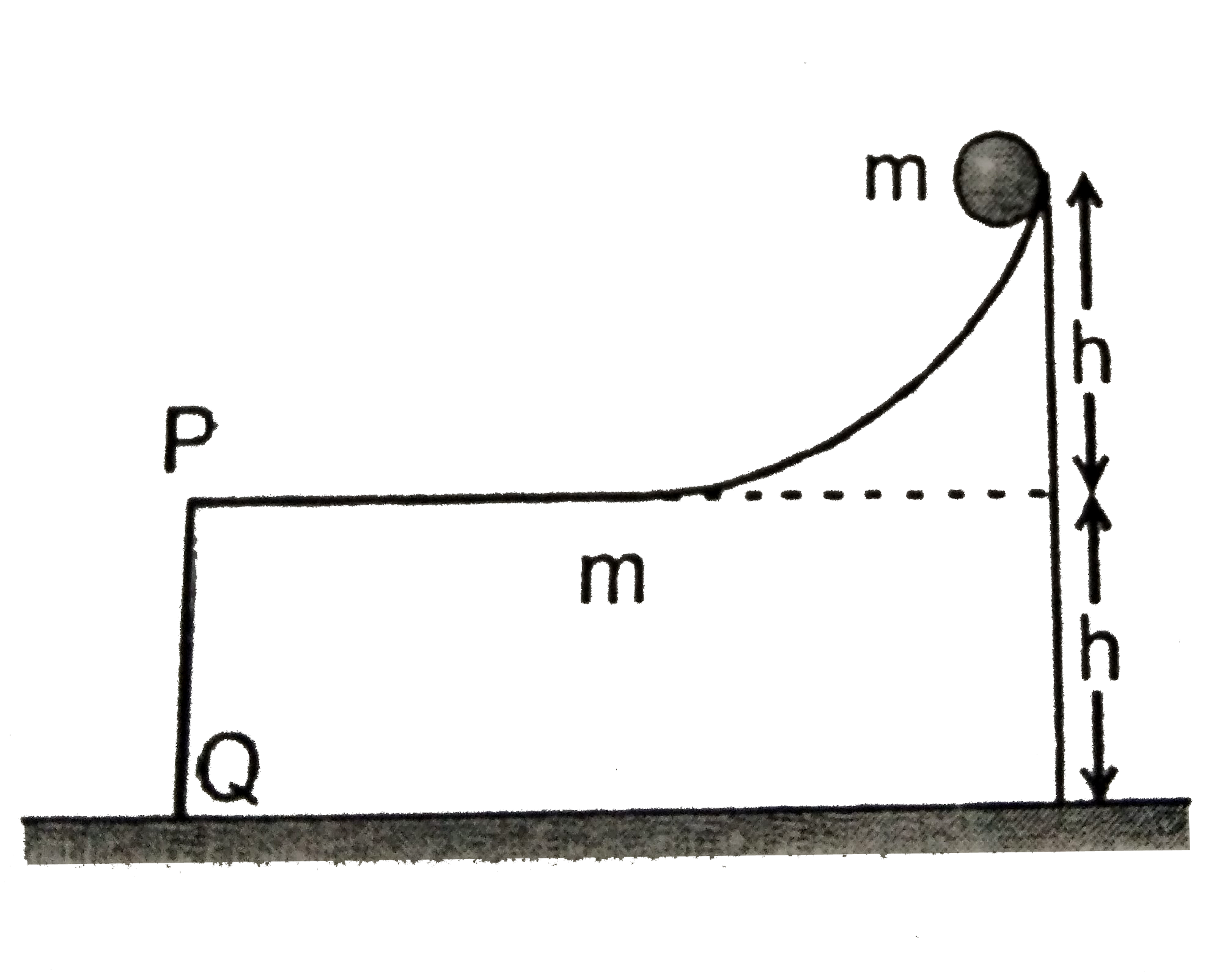
The total kinetic energy of the ball just before it falls on the ground
View SolutionA ball falls from a height such that it strikes the floor of lift at 10 m/s. If lift is moving in the upward direction with a velocity 1 m/s, then velocity with which the ball rebounds after elastic collision will be
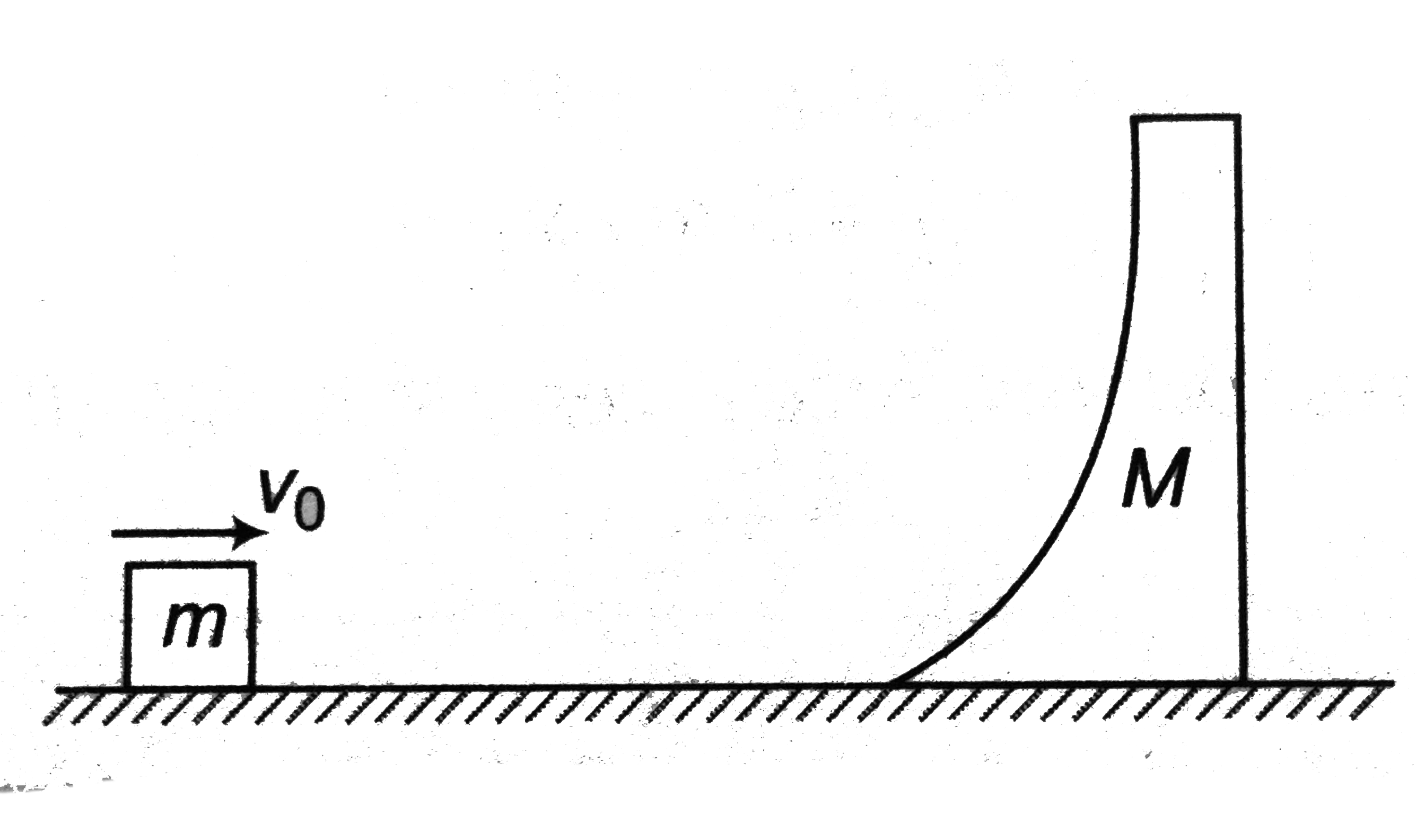
View SolutionAll surfaces shown in figure are smooth. Wedges of mass 'M' is free to move. Block of mass 'm' is given a horizontal velocity v0 as shown. Find the maximum height 'h' attained by 'm' (over the wedges or outside it).
View SolutionA ball of mass m is pushed with a horizontal velocity v0 from one end of a sledge of mass M and length l. if the ball stops after is first collision with the sledge, find the speeds of the ball ad sledge after the second collision of the ball with the sledge.

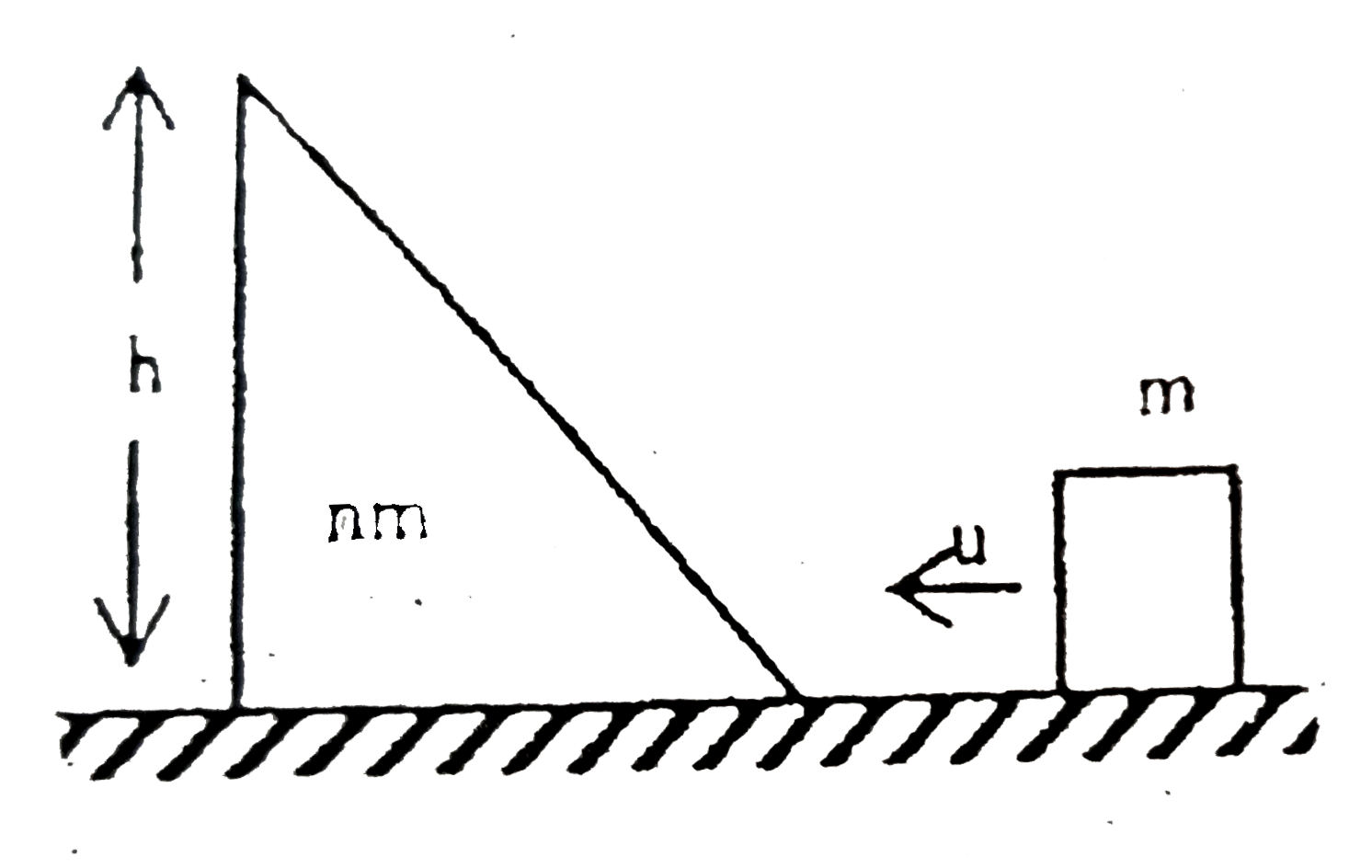
View SolutionA block of mass m is pushed towards a movable wedge of mass nm and height h, with a velocity u. All surfaces are smooth. The minimum value of u for which the block will reach the top of the wedge is
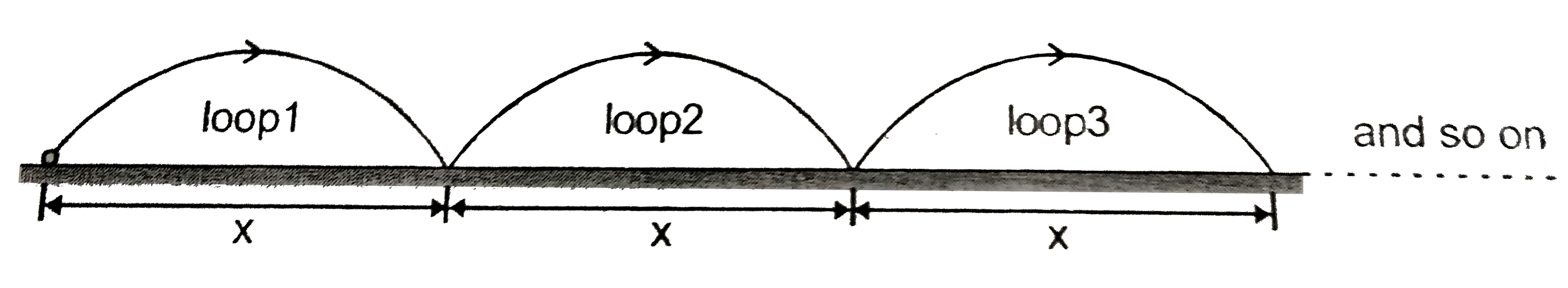
View SolutionA ball is projected on a very long floor. There may be two conditions (i) floor is smooth &(ii) the collision is elastic
If both the considered then the path of ball is as follows.
Now if collision is inelastic and surface is rough then the path is as follows.
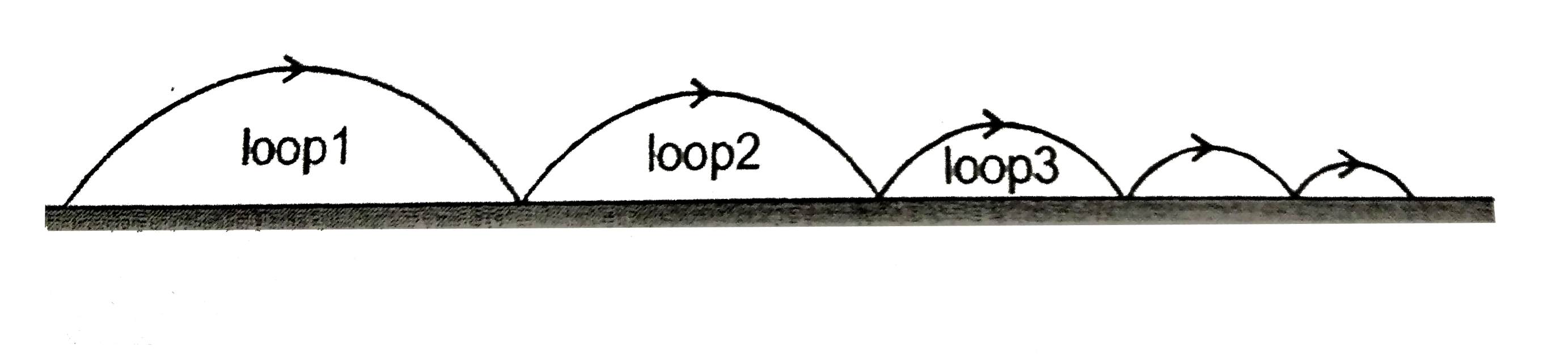
Successive range is decreasing .
Roughness of surface decreases the horizontal component of ball during collision and inelastic nature of collision decreases the vertical component of velocity of ball. In first case both components remain unchanged in magnitude and in second case both the components of the velocity will change.
Let us consider a third case here surface is rough but the collision of ball with floor is elastic. A ball is projected withe speed u at an angle 30∘ with horizontal and it is known that after collision with the floor its speed becomes u√3 . Then answer the following questions.
If the ball after first collision with the floor has rebounded vertically then the speed of the ball just after the collision with the floor would have been :
View Solution
