If the pressure and the volume of certain quantity of ideal gas are halved, then its temperature
is doubled
becomes one-fourth
remains constant
become four times
The correct Answer is:B
To solve the problem of how the temperature of an ideal gas changes when both its pressure and volume are halved, we can use the ideal gas law, which is given by the equation:
PV=nRT
Where:
- P = pressure of the gas
- V = volume of the gas
- n = number of moles of the gas
- R = universal gas constant
- T = temperature of the gas (in Kelvin)
1. Initial Conditions:
Let the initial pressure, volume, and temperature of the gas be P1, V1, and T1 respectively. According to the ideal gas law, we have:
P1V1=nRT1
2. New Conditions:
If the pressure and volume are both halved, the new pressure P2 and volume V2 can be expressed as:
P2=P12,V2=V12
3. Applying the Ideal Gas Law Again:
For the new conditions, we can write:
P2V2=nRT2
Substituting the new values of pressure and volume:
(P12)(V12)=nRT2
Simplifying this, we get:
P1V14=nRT2
4. Relating the Two Equations:
From the initial conditions, we know that P1V1=nRT1. We can substitute this into our equation for the new conditions:
nRT14=nRT2
5. Canceling Common Terms:
Since nR is common on both sides, we can cancel it out (assuming n and R are not zero):
T14=T2
6. Final Result:
Thus, the new temperature T2 is:
T2=T14
Conclusion:
If the pressure and volume of a certain quantity of ideal gas are halved, then its temperature becomes one-fourth of the initial temperature.
Answer:
The temperature is T14.
- P = pressure of the gas
- V = volume of the gas
- n = number of moles of the gas
- R = universal gas constant
- T = temperature of the gas (in Kelvin)
Let the initial pressure, volume, and temperature of the gas be P1, V1, and T1 respectively. According to the ideal gas law, we have:
P1V1=nRT1
If the pressure and volume are both halved, the new pressure P2 and volume V2 can be expressed as:
P2=P12,V2=V12
For the new conditions, we can write:
P2V2=nRT2
Substituting the new values of pressure and volume:
(P12)(V12)=nRT2
Simplifying this, we get:
P1V14=nRT2
From the initial conditions, we know that P1V1=nRT1. We can substitute this into our equation for the new conditions:
nRT14=nRT2
Since nR is common on both sides, we can cancel it out (assuming n and R are not zero):
T14=T2
Thus, the new temperature T2 is:
T2=T14
If the pressure and volume of a certain quantity of ideal gas are halved, then its temperature becomes one-fourth of the initial temperature.
The temperature is T14.
Topper's Solved these Questions
- KINETIC THEORYBOOK - NCERT FINGERTPS ENGLISHCHAPTER - KINETIC THEORYEXERCISE - Kinetic Theory of An Ideal Gas
18 Videos
- KINETIC THEORYBOOK - NCERT FINGERTPS ENGLISHCHAPTER - KINETIC THEORYEXERCISE - LAW OF EQUIPARTITION OF ENERGY
5 Videos
- KINETIC THEORYBOOK - NCERT FINGERTPS ENGLISHCHAPTER - KINETIC THEORYEXERCISE - Assertion And Reason
10 Videos
- GRAVITATIONBOOK - NCERT FINGERTPS ENGLISHCHAPTER - GRAVITATIONEXERCISE - Assertion And Reason
15 Videos
- LAWS OF MOTIONBOOK - NCERT FINGERTPS ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Assertion And Reason
15 Videos
Similar Questions
If both the temperature and the volume of an ideal gas are doubled, the pressure
View SolutionAt constant pressure, the ratio of increases in volume of an ideal gas per degree rise in kelvin temperature to its volume is
View SolutionWhich of the following is a correct plot of the volume of fixed amount of ideal gas as a function of temperature (at constant pressure)
View SolutionA certain quantity of a gas measured 500 mL at a temperature of 15∘C and 750 mm Hg What pressure is required to compress this quantity of gas into a 400 mL vessel at a temperature of 50∘C ?
View SolutionIf pressure and temperature of an ideal gas are doubled and volume is halved, the number of molecules of the gas
View SolutionAt a particular temperature, a certain quantity of gas occupies a volume of 74cm3 at a pressure of 760 mm. If the pressure is decreased to 740 mm, what will be the volume of the gas at the same temperaute ?
View SolutionOne mole of an ideal monotomic gas undergoes a linear process from A to B in which its pressure p and its volume V change as shown in figure.
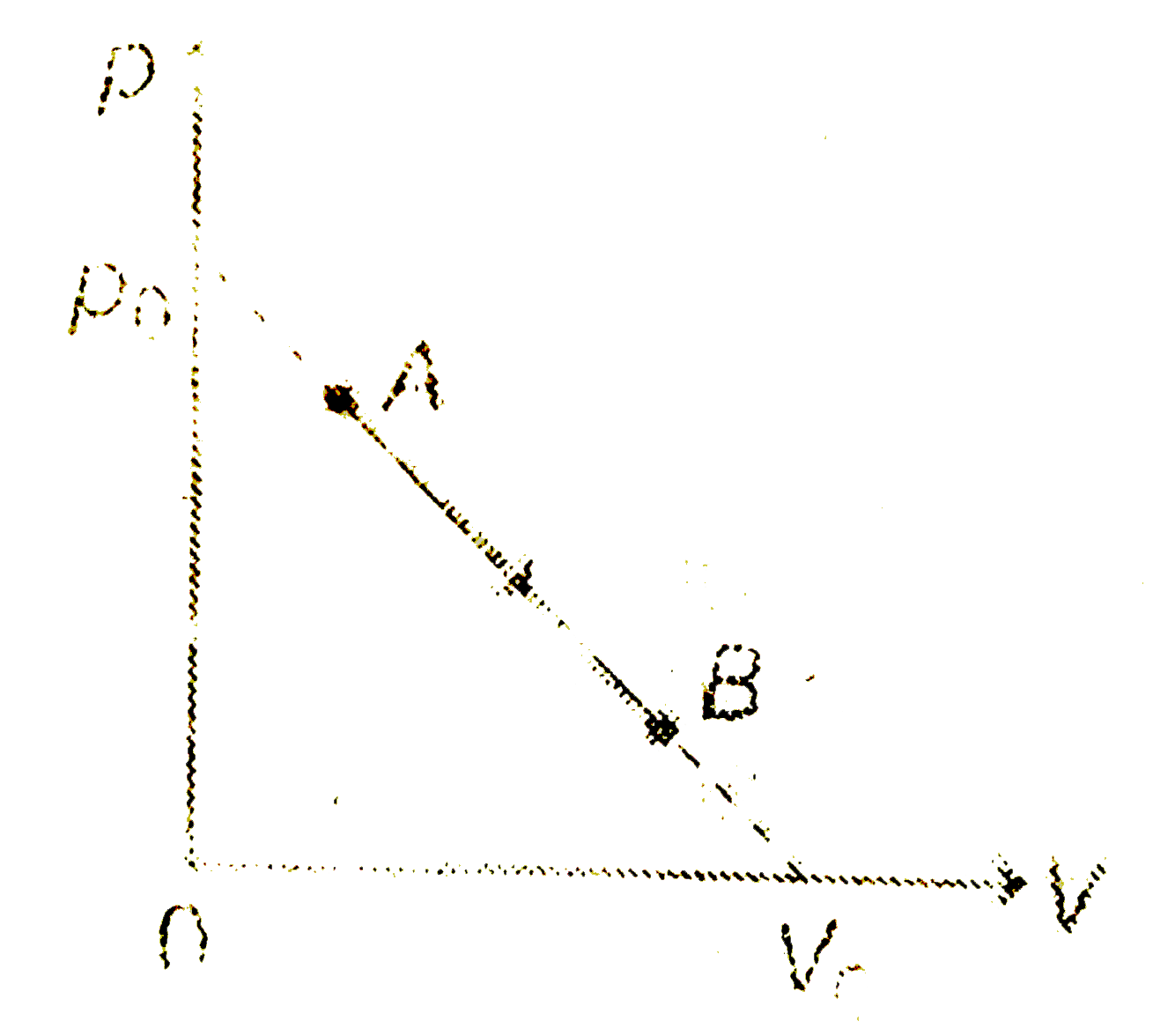
The maximum temperature of the gas during this process is
View SolutionAt a particular temperature, a certain quantity of gas occupies a volume of 74cm3 at a pressure gas occupies a volume of 74 cm at a pressure 740 mm, what will be the volume of the gas at the same temperature ?
View SolutionPressure versus temperature graph of an ideal gas at constant volume V is shown by the straight line A. Now mass of the gas is doubled and the volume is halved, then the corresponding pressure versus temperature graph will be shown by the line
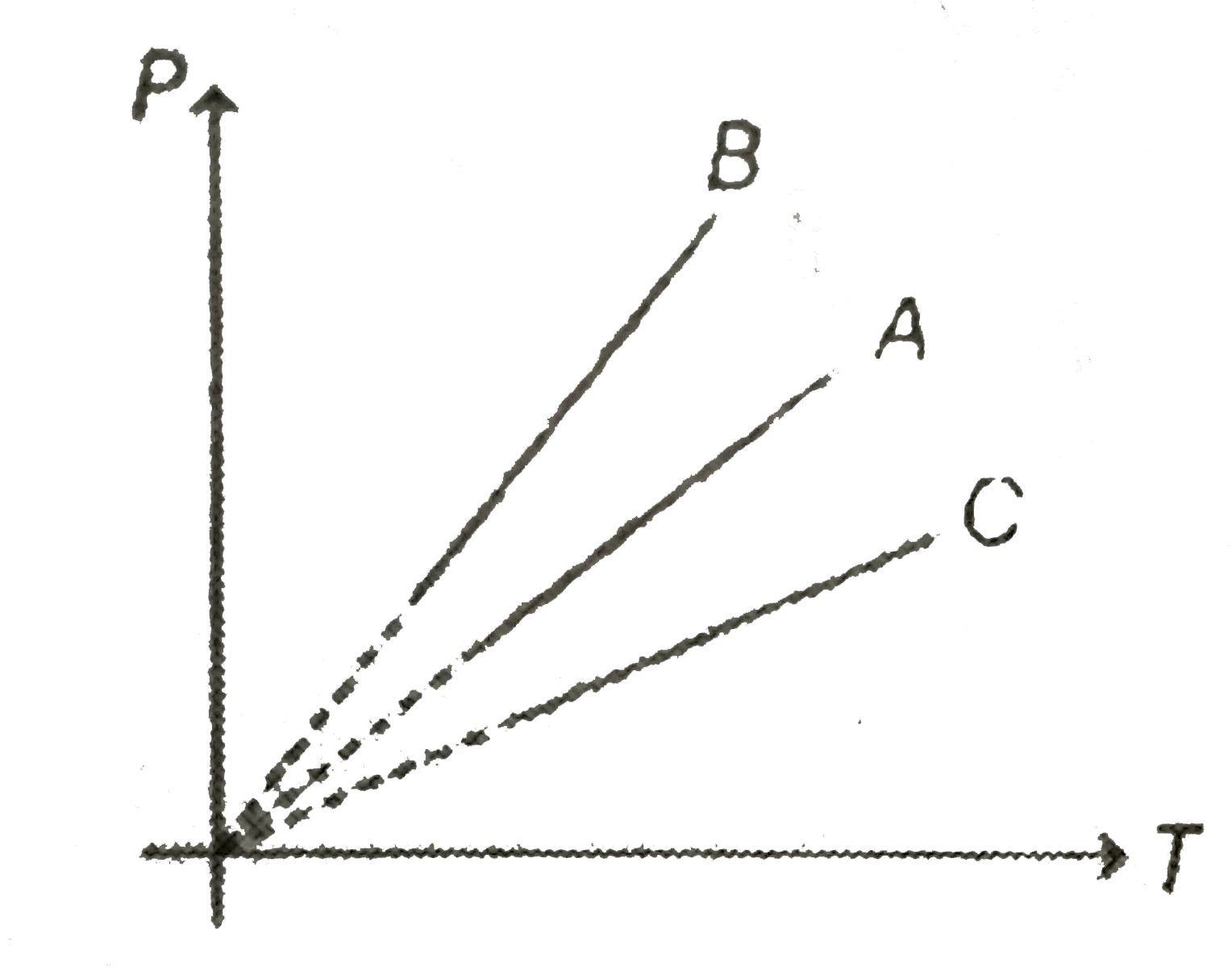
View SolutionA quantity of hydrogen gas occupies a volume of 30.0mL at a certain temperature and pressure. What volume would half of this mass of hydrogen occupy at triple the initial temperature, if the pressure was one-ninth that of the original gas?
View Solution
