Assertion: Each vibrational mode gives two degrees of freedom.
Reason: By law of equipartition of energy, the energy for each degree of freedom in thermal equlibrium is 2kBT.
If both assertion and reason are true and reason is the correct explanation os assertion.
If both assertion and reason are true but reason is not be correct explanation of assertion.
If assertion is true but reason is false.
If both assertion and reason are false.
The correct Answer is:C
To solve the question, we need to analyze both the assertion and the reason provided.
Step 1: Analyze the Assertion
The assertion states that "each vibrational mode gives two degrees of freedom."
- In kinetic theory, each vibrational mode of a molecule contributes two degrees of freedom: one for kinetic energy (due to the motion of the atoms in the mode) and one for potential energy (due to the displacement of the atoms from their equilibrium positions).
- Therefore, the assertion is true.
Step 2: Analyze the Reason
The reason states that "by the law of equipartition of energy, the energy for each degree of freedom in thermal equilibrium is 2kBT."
- The law of equipartition of energy actually states that each degree of freedom contributes an energy of 12kBT for kinetic energy and 12kBT for potential energy. Therefore, for each vibrational mode, which has two degrees of freedom (kinetic and potential), the total energy contribution is 12kBT+12kBT=kBT.
- The statement in the reason is incorrect because it states 2kBT instead of kBT. Hence, the reason is false.
Step 3: Conclusion
- The assertion is true, and the reason is false. Therefore, the correct answer to the question is that the assertion is true, but the reason is false.
Final Answer
- Assertion: True
- Reason: False
- Correct Answer: Assertion is true, Reason is false.
---
The assertion states that "each vibrational mode gives two degrees of freedom."
- Therefore, the assertion is true.
The reason states that "by the law of equipartition of energy, the energy for each degree of freedom in thermal equilibrium is 2kBT."
- The statement in the reason is incorrect because it states 2kBT instead of kBT. Hence, the reason is false.
- The assertion is true, and the reason is false. Therefore, the correct answer to the question is that the assertion is true, but the reason is false.
- Assertion: True
- Reason: False
- Correct Answer: Assertion is true, Reason is false.
Topper's Solved these Questions
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - KINETIC THEORYEXERCISE - Specific Heat Capacity13videos
KINETIC THEORY
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - KINETIC THEORYEXERCISE - Higher Order Thinking Skills10videos
KINETIC THEORY
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - KINETIC THEORYEXERCISE - EXEMPLAR PROBLEMS8videos
KINETIC THEORY
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - GRAVITATIONEXERCISE - Assertion And Reason15videos
GRAVITATION
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Assertion And Reason15videos
LAWS OF MOTION
Similar Questions
Knowledge Check
- Question 1 - Select One
According to the law of equipartition of energy, the energy associated with each degree of freedom is :
AE=kBTBE=12kBTCE=3kBTDE=32kBT
Assertion : The molecules of a monatomic gas has three degrees freedom.
Reason : The molecules of a diatomic gas has five degrees of freedom.View SolutionAssertion : Vibrational energy of diatomic molecule corresponding to each degree of freedom is kBT.
Reason : For every molecule, vibrational degree of freedom is 2.View SolutionThe total energy of molecules is divided equally amongst the various degrees of freedom of a molecule. The distribution of kinetic energy along x, y, z axis are EKx,EKy,EKz
Total K.e =EKx+EKy+EKz
Since the motion of molecule is equally probable in all the three directions, therefore
EKx=EKy=EKz=13EK=13×32kT=12kT, where k=RNA = Botzman constant.
K.E.=12kT per molecule or =12RT per mole.
In vibration motion, molecules possess both kinetic energy as well as potential energy. This means energy of vibration involves two degrees of fiuedom.
Vibration energy =2×12kT=2×12RT [∴ two degrees of freedom per mole]
If the gas molecules have n1 translational degrees of freedom, n2 rotational degrees of freedom and n3 vibrational degrees of freedom, that total energy = n1[kT2]+n2[kT2]+n3[kT2]×2
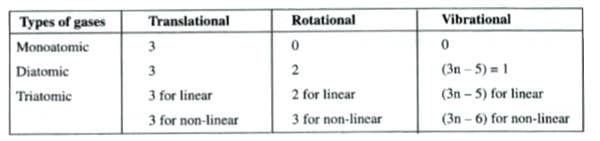
Where 'n' is atomicity of gas.
How many total degrees of freedom are present in H2 molecules in all types of motions ?View SolutionThe total energy of molecules is divided equally amongst the various degrees of freedom of a molecule. The distribution of kinetic energy along x, y, z axis are EKx,EKy,EKz
Total K.e =EKx+EKy+EKz
Since the motion of molecule is equally probable in all the three directions, therefore
EKx=EKy=EKz=13EK=13×32kT=12kT, where k=RNA = Botzman constant.
K.E.=12kT per molecule or =12RT per mole.
In vibration motion, molecules possess both kinetic energy as well as potential energy. This means energy of vibration involves two degrees of fiuedom.
Vibration energy =2×12kT=2×12RT [∴ two degrees of freedom per mole]
If the gas molecules have n1 translational degrees of freedom, n2 rotational degrees of freedom and n3 vibrational degrees of freedom, that total energy = n1[kT2]+n2[kT2]+n3[kT2]×2
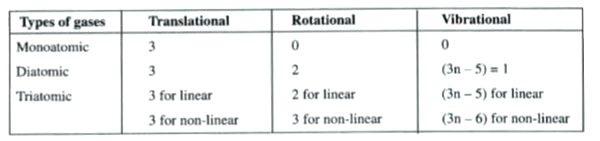
Where 'n' is atomicity of gas.
The vibrational kinetic energy of CO2 molecule isView SolutionThe total energy of molecules is divided equally amongst the various degrees of freedom of a molecule. The distribution of kinetic energy along x, y, z axis are EKx,EKy,EKz
Total K.e =EKx+EKy+EKz
Since the motion of molecule is equally probable in all the three directions, therefore
EKx=EKy=EKz=13EK=13×32kT=12kT, where k=RNA = Botzman constant.
K.E.=12kT per molecule or =12RT per mole.
In vibration motion, molecules possess both kinetic energy as well as potential energy. This means energy of vibration involves two degrees of fiuedom.
Vibration energy =2×12kT=2×12RT [∴ two degrees of freedom per mole]
If the gas molecules have n1 translational degrees of freedom, n2 rotational degrees of freedom and n3 vibrational degrees of freedom, that total energy = n1[kT2]+n2[kT2]+n3[kT2]×2
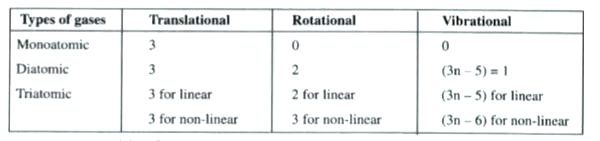
Where 'n' is atomicity of gas.
The rotational kinetic energy of H20 molecule is equal toView SolutionThe number of degrees of freedom for each atom of a monoatomic gas will be
View SolutionAccording to equipartition law of energy each particle in a system of particles have thermal energy E equal to
View Solution

